1. Home
  2. >Calculus
  3. >Limits
Help

Limits at infinity - horizontal asymptotes

Everything You Need in One Place

Homework problems? Exam preparation? Trying to grasp a concept or just brushing up the basics? Our extensive help & practice library have got you covered.

Learn and Practice With Ease

Our proven video lessons ease you through problems quickly, and you get tonnes of friendly practice on questions that trip students up on tests and finals.

Instant and Unlimited Help

Our personalized learning platform enables you to instantly find the exact walkthrough to your specific type of question. Activate unlimited help now!

Get the most by viewing this topic in your current grade. Pick your course now.

?
Intros
Lessons
  1. Introduction to Horizontal Asymptotes
  2. opposite relationship between "vertical asymptote" and "horizontal asymptote"
  3. how horizontal asymptotes are defined on each end of a function
  4. evaluate limits at infinity algebraically –"Highest Power Rule"!
  5. lesson overview
?
Examples
Lessons
  1. Relate Asymptotes to Limits
    Express all asymptotes in limit notations for the function f f whose graph is shown below.
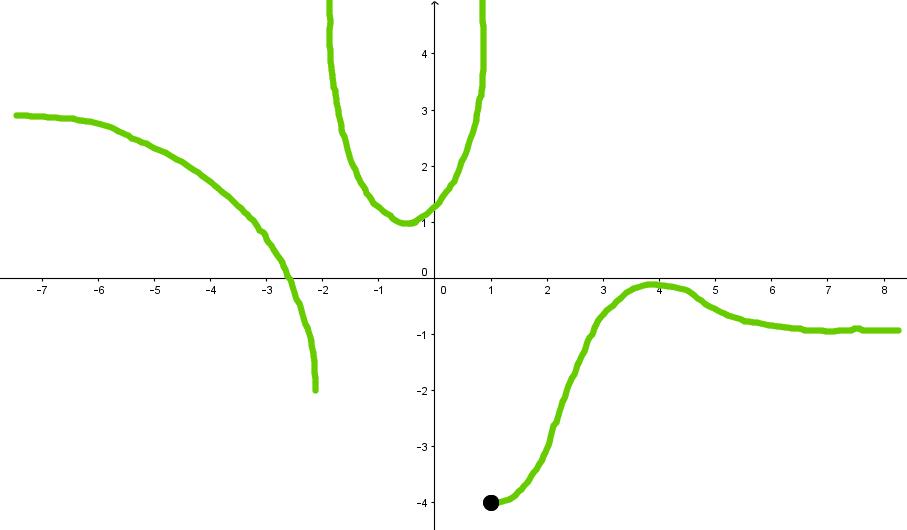
    Finding limits of a function algebraically by direct substitution
    1. Discuss the Foundation of Limits at Infinity
      Find:
      i) limx→∞  1xlim_{x \to \infty } \;\frac{1}{x}
      ii) limx→−∞  1xlim_{x \to - \infty } \;\frac{1}{x}
      1. Use "Highest Power Rule" to Evaluate Limits at Infinity of Rational Functions in 3 Types
        Find:
        1. limx→∞  −5x2+13x+1002x2−8lim_{x \to \infty } \;\frac{{-5x^2+13x+100}}{{2x^2-8}}
          (Type 1: degree of numerator = degree of denominator)
        2. limx→−∞  2x−9x3+7x2+10x+21lim_{x \to - \infty } \;\frac{{2x - 9}}{{{x^3} + 7{x^2} + 10x + 21}}
          (Type 2: degree of numerator < degree of denominator)
        3. limx→∞  x2−3x+115−xlim_{x \to \infty } \;\frac{{{x^2} - 3x + 11}}{{5 - x}}
          (Type 3: degree of numerator > degree of denominator)
      2. Evaluate Limits at Infinity of Functions Involving Radicals
        Find the horizontal asymptotes of the function f(x)=3x2+7x−10005x+8f\left( x \right) = \frac{{\sqrt {3{x^2} + 7x - 1000} }}{{5x + 8}} by evaluating:
        i) limx→∞  3x2+7x−10005x+8lim_{x \to \infty } \;\frac{{\sqrt {3{x^2} + 7x - 1000} }}{{5x + 8}}
        ii) limx→−∞  3x2+7x−10005x+8lim_{x \to - \infty } \;\frac{{\sqrt {3{x^2} + 7x - 1000} }}{{5x + 8}}
        1. Multiply Conjugates First, then Evaluate Limits
          Find:
          1. limx→∞  (9x2+12x−3x)lim_{x \to \infty } \;\left( {\sqrt {9{x^2} + 12x} - 3x} \right)
          2. limx→−∞  (x+x2−5x)lim_{x \to - \infty } \;\left( {x + \sqrt {{x^2} - 5x} } \right)
        2. Infinite Limits at Infinity
          Find:
          i) limx→∞  x3lim_{x \to \infty } \;{x^3}
          ii) limx→−∞  x3lim_{x \to - \infty } \;{x^3}
          1. Ambiguous Case: ∞−∞ \infty - \infty
            Find limx→∞  x2−xlim_{x \to \infty } \;{x^2} - x
            1. Limits at Infinity of Exponential Functions
              Find:
              i) limx→∞  exlim_{x \to \infty } \;{e^x}
              ii) limx→−∞  exlim_{x \to - \infty } \;{e^x}
              1. Limits at Infinity of Trigonometric Functions
                Find limx→∞  sin⁡xlim_{x \to \infty } \;\sin x
                Topic Notes
                ?
                There are times when we want to see how a function behaves near a horizontal asymptote. Much like finding the limit of a function as x approaches a value, we can find the limit of a function as x approaches positive or negative infinity. In this section, we will learn how to evaluate limits at infinity algebraically using the "Highest Power Rule", with tricks like using conjugates, common denominators, and factoring.
                The line y=Ly = L is a horizontal asymptote of the curve y=f(x)y = f(x) in any of the following two cases:
                i)limx→∞f(x)=Llim_{x \to\infty } f\left( x \right) = L
                ii) limx→,−∞f(x)=Llim_{x \to,-\infty } f\left( x \right) = L

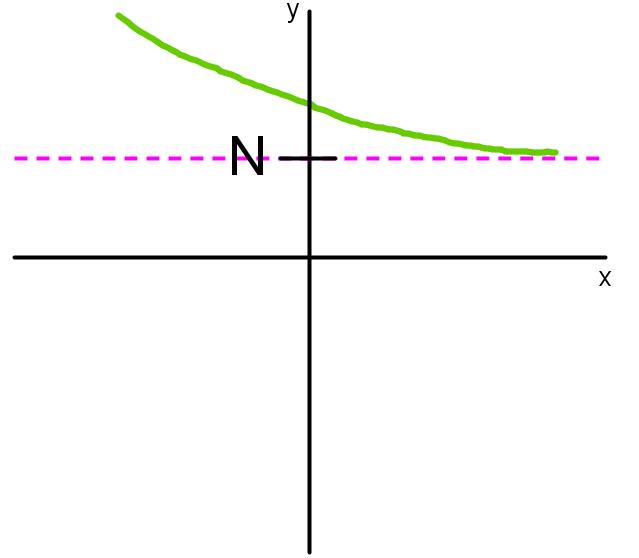
                horizontal asymptote in limit notation positive infinity

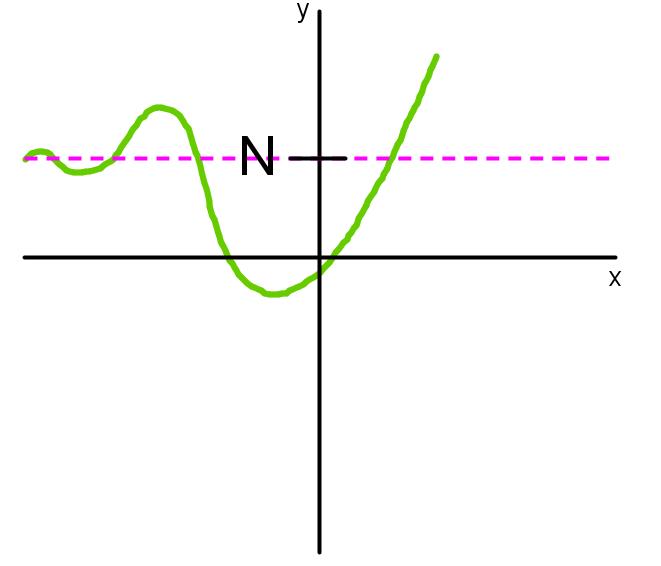
                horizontal asymptote in limit notation negative infinity

                or

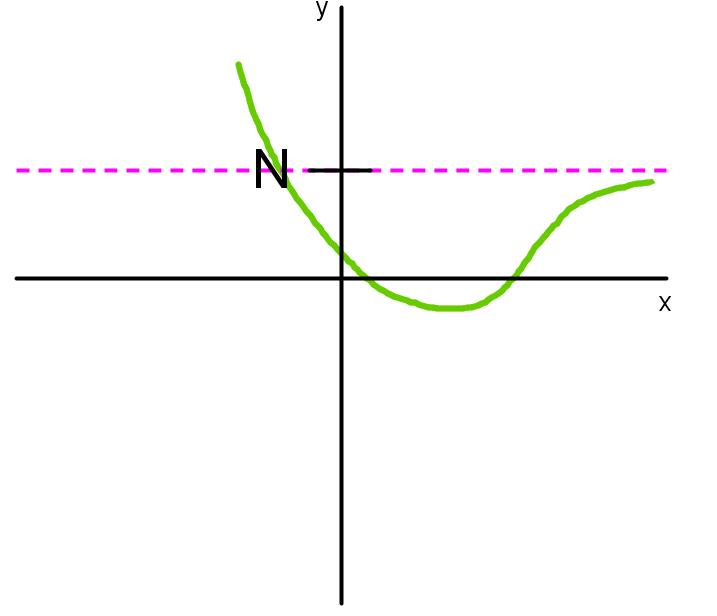
                horizontal asymptote in limit notation positive infinity 2

                or

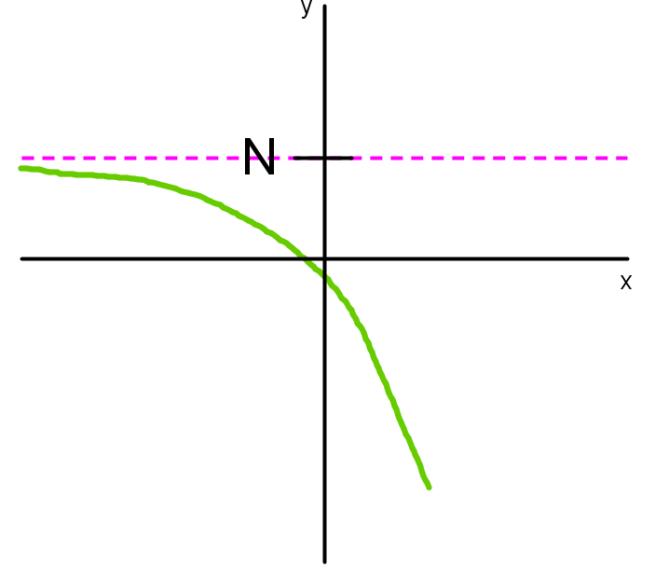
                horizontal asymptote in limit notation negative infinity 2