Volumes of solid with known cross-sections

Introduction

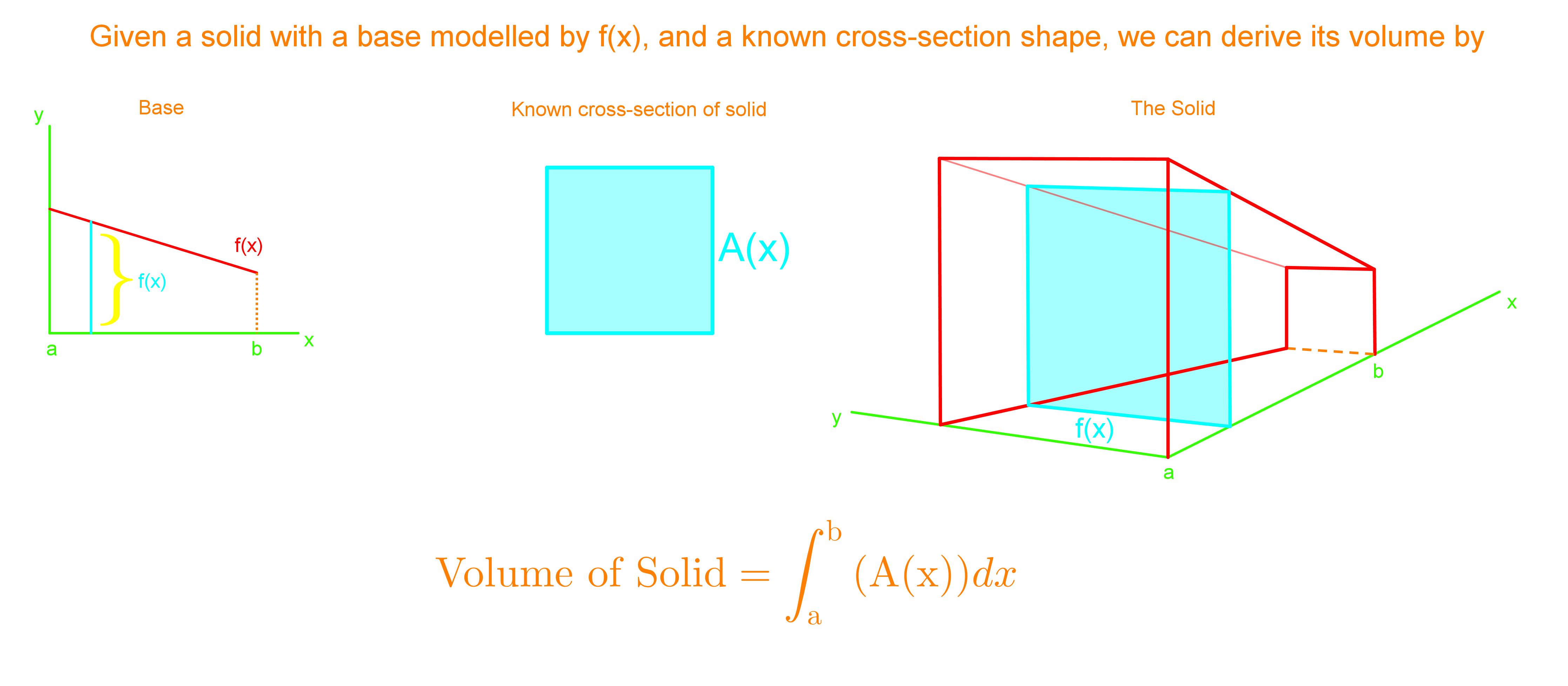
Finding the volume of solids with known cross-sections is a fundamental concept in calculus and geometry. The introduction video provides a crucial foundation for understanding this method, which involves integrating cross-sectional areas to calculate volume. This technique is particularly useful when dealing with complex shapes that may not have simple formulas for volume calculation. By slicing the solid into thin cross-sections and summing up their areas, we can approximate the total volume with increasing accuracy as the slices become thinner. The process relies on the principle of integration, where we accumulate infinitesimally small pieces to obtain the whole. This method is applicable to a wide range of solids, from simple cylinders to more intricate shapes like vases or irregular objects. Understanding cross sections of solids and their relationship to volume calculation is essential for students in mathematics, engineering, and physics, as it forms the basis for more advanced concepts in multivariable calculus and solid geometry.

Understanding Cross-Sections

Cross-sections are a fundamental concept in solid geometry, providing valuable insights into the internal structure of three-dimensional objects. To understand cross-sections better, let's use a simple analogy from everyday life: imagine a book. When you open a book and look at its pages, you're essentially viewing a series of cross-sections of the entire book. Each page represents a slice or a cross-section of the book as a whole.

In the world of solid geometry, cross-sections are obtained by cutting through a solid object with a plane. This process is similar to slicing through a loaf of bread. Each slice reveals the internal structure of the loaf at that particular point. In geometry, these "slices" are called cross-sections, and they provide valuable information about the shape and composition of the solid object.

One of the most fascinating aspects of cross-sections is their variability. Depending on where and how you make the cut through a solid, you can obtain cross-sections of different shapes and sizes. This variability is crucial in understanding the complex nature of three-dimensional objects. For instance, if you slice a cone at different angles and positions, you might get circles, ellipses, or even triangles as cross-sections.

Common cross-section shapes include squares, circles, rectangles, and trapezoids, among others. A cube, for example, will always have a square cross-section if cut parallel to one of its faces. However, if you cut it diagonally, you might get a rectangle or even a hexagon. A sphere, on the other hand, will always have a circular cross-section, regardless of where you make the cut.

The concept of cross-sectional area is particularly important in various fields, including engineering, architecture, and physics. It refers to the area of the surface exposed when a solid object is cut by a plane. This measurement is crucial for calculating volumes, understanding fluid dynamics, and designing structures.

In mathematics and engineering, the study of cross-sections helps in visualizing and analyzing complex three-dimensional objects. By examining cross-sections at different points, we can gain a comprehensive understanding of an object's internal structure and composition. This technique is widely used in medical imaging, where CT scans and MRIs create cross-sectional images of the human body for diagnostic purposes.

Understanding cross-sections also plays a vital role in manufacturing and construction. Engineers and architects often use cross-sectional drawings to illustrate the internal details of buildings, machines, and other structures. These drawings provide essential information about materials, dimensions, and the arrangement of components within a larger system.

In conclusion, cross-sections are a powerful tool for understanding and analyzing three-dimensional objects. By slicing through solids, we can reveal their internal structure, measure cross-sectional areas, and gain insights into their overall composition. Whether you're studying geometry, working in engineering, or simply curious about the world around you, understanding cross-sections opens up a new dimension of spatial awareness and analytical thinking.

The Concept of Stacking Cross-Sections

The idea of stacking cross-sections to form a solid is a fundamental concept in calculus, particularly in the realm of integration and volume calculation. This approach, beautifully demonstrated with book pages in the video, provides a tangible way to understand how three-dimensional objects can be constructed and analyzed mathematically.

Imagine a book as a solid object. Each page represents a thin cross-section of this solid. When we stack these pages together, we recreate the entire volume of the book. This simple analogy serves as a powerful visualization tool for understanding more complex mathematical concepts.

In calculus, this concept directly relates to integration. Integration is essentially the process of summing up infinitely many infinitesimally small pieces to calculate areas, volumes, or other quantities. When we think about calculating the volume of an irregularly shaped object, we can apply this stacking method conceptually.

Here's how it works: We slice the object into very thin cross-sections perpendicular to one axis. Each of these cross-sections has a specific area that may vary depending on its position along the axis. By "adding up" all these cross-sectional areas along the length of the object, we can determine its volume. This is precisely what integration does in calculus.

The transition from discrete stacking (like book pages) to continuous integration for smooth solids is a crucial step in understanding this concept. With book pages, we have a finite number of discrete cross-sections. However, for a smooth solid, we need to consider an infinite number of infinitesimally thin cross-sections.

This is where the power of calculus comes into play. Instead of manually adding up a finite number of cross-sectional areas, we use integration to sum up an infinite number of infinitesimally thin slices. The integral symbol () itself visually represents this idea of summing up many small pieces.

In mathematical terms, if we have a function f(x) that describes the cross-sectional area at any point x along the axis, the volume V of the solid between points a and b is given by the definite integral formula: V = [a to b] f(x) dx. This formula essentially says, "Add up all the cross-sectional areas from point a to point b."

The beauty of this approach is its versatility. It can be applied to a wide range of shapes and solids, from simple cylinders to complex, irregularly shaped objects. For instance, calculating the volume of a cone becomes straightforward when you consider it as a stack of circular cross-sections, each with a different radius depending on its height.

This method also extends to more advanced applications in physics and engineering. For example, in fluid dynamics, engineers might use this concept to calculate the flow rate of a liquid through an irregularly shaped pipe. In computer graphics, 3D modeling often involves constructing complex shapes from simpler cross-sections.

Understanding the transition from discrete to continuous is crucial in grasping the full power of calculus. While we can approximate volumes using a finite number of cross-sections (like the pages of a book), true mathematical precision comes from the limit as the number of sections approaches infinity and their thickness approaches zero. This limit process is at the heart of integral calculus.

In conclusion, the concept of stacking cross-sections to form a solid is not just a mathematical trick; it's a powerful way of visualizing and understanding three-dimensional objects. It bridges the gap between tangible, physical objects (like a book) and abstract mathematical concepts (like integration). By mastering this concept, students of calculus gain a deeper appreciation for the practical applications of integration in real-world problem-solving, from engineering to physics and beyond.

The Volume Formula for Solids with Known Cross-Sections

Understanding how to calculate the volume of a solid using cross-sections is a fundamental concept in calculus and geometry. The formula V = [a to b] A(x) dx provides a powerful tool for determining the volume of complex shapes. In this section, we'll break down this formula and provide a step-by-step guide on how to apply it effectively.

Breaking Down the Formula

The volume formula for solids with known cross-sections is expressed as:

V = [a to b] A(x) dx

Let's examine each component:

• V: This represents the volume of the solid.
• : The integral symbol, indicating we're summing up infinitesimal slices of the solid.
• [a to b]: These are the bounds of integration, representing the start and end points of the solid along the axis of integration.
• A(x): This function describes the area of the cross-section at any point x along the axis of integration.
• dx: This represents an infinitesimal slice of the solid along the x-axis.

Understanding the Cross-Sectional Area Function

The cross-sectional area function A(x) is crucial in this formula. It describes how the area of the solid's cross-section changes as you move along the axis of integration. This function can take various forms depending on the shape of the solid. For example:

• For a cylinder, A(x) would be constant (πr²).
• For a cone, A(x) might be a quadratic function like πr²(h-x)²/h², where h is the height of the cone.
• For more complex shapes, A(x) could be a more intricate function.

Step-by-Step Guide to Applying the Formula

1. Identify the solid and its orientation: Determine the shape of the solid and choose an appropriate axis of integration.
2. Determine the bounds of integration: Identify the start (a) and end (b) points of the solid along your chosen axis.
3. Find the cross-sectional area function: This is often the most challenging step. Analyze how the cross-sectional area changes along the axis of integration and express it as a function A(x).
4. Set up the integral: Write out the full integral expression: V = [a to b] A(x) dx.
5. Evaluate the integral: Use integration techniques to solve the integral. This may involve substitution, integration by parts, or other methods depending on the complexity of A(x).
6. Simplify and interpret: Simplify your result and ensure it makes sense in the context of the problem.

The Importance of Correctly Identifying A(x)

The accuracy of your volume calculation heavily depends on correctly identifying the cross-sectional area function A(x). This function is the heart of the formula and directly represents the shape of your solid. Mistakes in determining A(x) will lead to incorrect volume calculations. Here are some tips for identifying A(x):

• Visualize cross-sections perpendicular to your axis of integration.
• Consider how these cross-sections change as you move along the axis.
• Use geometric formulas for areas of common shapes (circles, squares, triangles) that might appear in cross-sections.
• For complex solids, you may need to break the cross-section into simpler shapes and sum

  Examples of Solids with Known Cross-Sections

  Understanding the cross-sections of various solids is crucial for calculating their volumes using integration techniques. Let's explore several examples of solids with known cross-sections and how they change along the axis of integration.

  1. Pyramid

  A pyramid is a classic example of a solid with varying cross-sections. Consider a square pyramid with a base side length of 10 units and a height of 12 units.

  • Cross-section shape: Square
  • Change along axis: The area of the square cross-sections decreases as we move from the base to the apex
  • Cross-sectional area at height h: A(h) = (10 - 10h/12)²

  2. Cone

  A cone is another common solid with circular cross-sections. Let's consider a cone with a base radius of 5 units and a height of 8 units.

  • Cross-section shape: Circle
  • Change along axis: The area of the circular cross-sections decreases as we move from the base to the apex
  • Cross-sectional area at height h: A(h) = π(5 - 5h/8)²

  3. Sphere

  A sphere has circular cross-sections that vary in size. Consider a sphere with a radius of 6 units.

  • Cross-section shape: Circle
  • Change along axis: The area of the circular cross-sections increases from the poles to the equator, then decreases
  • Cross-sectional area at height h: A(h) = π(6² - h²)

  4. Ellipsoid

  An ellipsoid has elliptical cross-sections that vary in size and shape. Consider an ellipsoid with semi-axes a = 4, b = 3, and c = 2 units.

  • Cross-section shape: Ellipse
  • Change along axis: The area and shape of the elliptical cross-sections vary along all axes
  • Cross-sectional area perpendicular to z-axis at height h: A(h) = πab(1 - h²/c²)

  5. Torus

  A torus (donut shape) has circular cross-sections that vary in position. Consider a torus with major radius R = 5 units and minor radius r = 2 units.

  • Cross-section shape: Circle
  • Change along axis: The position of the circular cross-sections changes as we rotate around the central axis
  • Cross-sectional area: A = πr² (constant)

  Worked-out Problem: Volume of a Cone

  Let's calculate the volume of the cone described earlier (base radius 5 units, height 8 units) using integration.

  1. Set up the integral: V = A(h) dh
  2. Substitute the cross-sectional area function: V = π(5 - 5h/8)² dh
  3. Expand the integrand: V = π(25 - 25h/4 + 25h²/64) dh
  4. Integrate: V = π[25h - 25h²/8 + 25h³/192]
  5. Evaluate the limits: V

     Applications and Real-World Examples

     Calculating volumes using cross-sections is a powerful technique with numerous practical applications across various industries. This method, which involves analyzing the cross-sectional areas of solids at different points, proves invaluable in engineering, architecture, and manufacturing. Let's explore some real-world examples where cross-sections of solids play a crucial role in volume calculations.

     In engineering, the use of cross-sections for volume calculations is particularly prominent in the design and construction of storage tanks. Engineers must accurately determine the capacity of these tanks to ensure they meet specific requirements. For instance, when designing a conical or cylindrical water tower, engineers use cross-sectional analysis to calculate the exact volume it can hold. This information is critical for urban planning and water supply management.

     The construction industry heavily relies on cross-sectional volume calculations for estimating material volumes. When excavating for foundations or landscaping projects, contractors need to know the volume of soil to be removed or added. By taking cross-sections of the land at regular intervals, they can accurately calculate the volume of earth to be moved, helping in project planning and cost estimation.

     In architecture, cross-sectional volume calculations are essential for designing energy-efficient buildings. Architects use this method to determine the volume of spaces within a structure, which is crucial for heating, ventilation, and air conditioning (HVAC) system design. By accurately calculating the volume of rooms and corridors, they can ensure optimal air circulation and temperature control.

     The manufacturing sector also benefits greatly from cross-sectional volume calculations. In the production of irregularly shaped containers or products, such as custom bottles or automotive parts, manufacturers use this technique to determine the exact volume of materials needed. This precision helps in reducing waste and optimizing production costs.

     Another fascinating application is in the field of medical imaging. Radiologists and medical professionals use cross-sectional imaging techniques like CT scans and MRIs to calculate the volume of organs or tumors. This information is crucial for diagnosis, treatment planning, and monitoring the progression of diseases.

     In the aerospace industry, engineers use cross-sectional volume calculations to design fuel tanks for aircraft and spacecraft. The irregular shapes of these vehicles require precise volume calculations to ensure they can carry sufficient fuel for their missions while maintaining optimal weight distribution.

     Environmental scientists and hydrologists employ cross-sectional volume calculations to estimate the capacity of reservoirs and lakes. By analyzing cross-sections at various points, they can determine water storage capacity and predict how water levels might change under different conditions, which is vital for water resource management and flood control.

     In conclusion, the application of cross-sectional volume calculations extends far beyond theoretical mathematics. From designing efficient storage solutions to estimating construction materials, and from medical diagnostics to environmental management, this method proves indispensable in solving real-world problems across diverse fields. The ability to accurately calculate volumes using cross-sections of solids continues to drive innovation and precision in numerous industries, highlighting its enduring importance in practical applications.

     Conclusion

     Understanding cross-sections of solids is crucial for accurate volume calculations. This article has explored the fundamental concepts and techniques involved in using integration to determine volumes. The introduction video provided a valuable visual aid, helping to solidify the abstract ideas presented. Key points covered include the relationship between cross-sectional area and volume, the importance of selecting appropriate integration limits, and the application of various integration methods. Readers are encouraged to practice solving problems involving volumes of solids with known cross-sections, starting with simple shapes and progressing to more complex examples. By mastering these concepts, students and professionals alike can tackle a wide range of real-world applications in fields such as engineering, physics, and mathematics. Remember, the ability to visualize and analyze cross-sections is a powerful tool in volume calculation, opening doors to advanced problem-solving in three-dimensional space.

  FAQs

  1. What is the cross section of a 3D object?

     A cross section of a 3D object is the shape that results from intersecting the object with a plane. It reveals the internal structure of the object at that specific point. For example, the cross section of a cylinder cut perpendicular to its axis is a circle, while a diagonal cut might produce an ellipse.

  2. What is cross-sectional area of solids?

     The cross-sectional area of a solid is the area of the surface exposed when the solid is cut by a plane. It's crucial for volume calculations using integration. For instance, in a cone, the cross-sectional area varies depending on where the cut is made, typically forming circles of different sizes.

  3. How do you describe cross-sections?

     Cross-sections are described by their shape, size, and how they change along the axis of the solid. For example, a sphere's cross-sections are circles that vary in size, largest at the center and smallest near the poles. When describing, specify the plane of intersection and how the cross-section changes as you move through the solid.

  4. How to identify cross section?

     To identify a cross section, visualize slicing the object with a plane and observe the resulting shape. Consider the object's geometry and the angle of the cut. For complex shapes, it may help to use physical models or 3D visualization software. Practice with simple objects like cubes or cylinders before moving to more complex shapes.

  5. How are cross-sections used in volume calculations?

     Cross-sections are integral to calculating volumes of irregular solids. By integrating the cross-sectional area function A(x) over the length of the solid, we can determine its volume. The formula V = [a to b] A(x) dx sums up all infinitesimal cross-sectional areas to give the total volume. This method is particularly useful for solids where traditional volume formulas don't apply.

  Prerequisite Topics

  Understanding the volumes of solids with known cross-sections is a crucial concept in advanced mathematics, particularly in calculus and geometry. To fully grasp this topic, it's essential to have a strong foundation in several prerequisite areas. These foundational concepts not only provide the necessary tools to tackle volume calculations but also enhance your overall mathematical reasoning skills.

  One of the most critical prerequisites is the definite integral. This concept is fundamental to calculating volumes of solids, as it allows us to sum up infinitesimal slices of the solid to determine its total volume. The definite integral formula provides a powerful method for computing these volumes, especially when dealing with irregular shapes or solids with varying cross-sections.

  Another important prerequisite is an introduction to surface area of 3-dimensional shapes. This topic lays the groundwork for understanding how three-dimensional objects are analyzed and measured. By familiarizing yourself with various 3D shapes and their properties, you'll be better equipped to visualize and comprehend the solids whose volumes you'll be calculating.

  Building upon this introduction, a deeper understanding of the surface area of 3-dimensional shapes is crucial. This knowledge helps you recognize how the cross-sections of a solid relate to its overall structure and volume. It also provides insights into how changes in a solid's dimensions affect its volume, which is essential when working with known cross-sections.

  The relationship between these prerequisites and the main topic of volumes of solids with known cross-sections is intricate and multifaceted. The definite integral serves as the mathematical tool for summing up the areas of cross-sections to determine volume. Meanwhile, the understanding of 3D shapes and their surface areas provides the spatial reasoning necessary to interpret and apply the cross-sectional information effectively.

  By mastering these prerequisite topics, you'll develop a robust framework for tackling volume calculations. You'll be able to visualize how a solid is constructed from its cross-sections, understand how to set up the appropriate integrals, and interpret the results in a meaningful way. This comprehensive approach not only aids in solving specific problems but also enhances your overall mathematical intuition and problem-solving skills.

  In conclusion, the journey to understanding volumes of solids with known cross-sections is built upon these fundamental concepts. By investing time in thoroughly grasping these prerequisites, you'll find yourself well-prepared to explore more complex volumetric calculations and applications in various fields, from engineering to physics and beyond.