A polynomial function is a function in the form:
leading coefficient: "", the coefficient of the highest power of x constant term: "", the term without
degree of the polynomial function: , the highest power of
Which of the following are not polynomial functions? Explain.
Complete the chart:
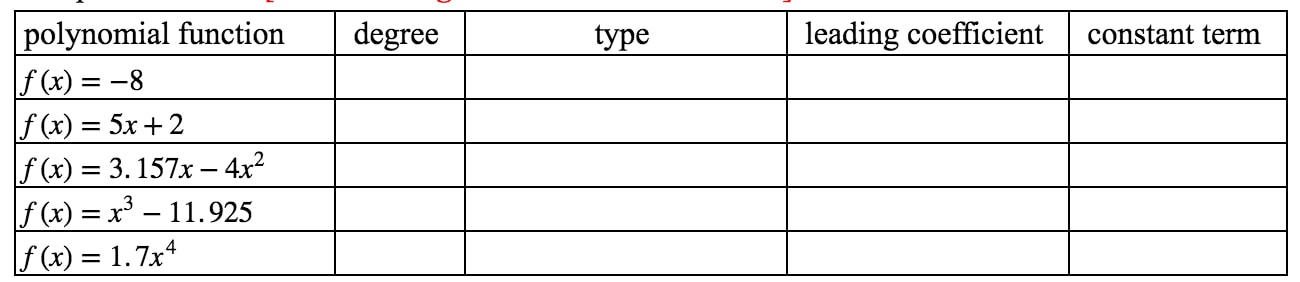



A polynomial function is a function in the form:
Welcome to the fascinating world of polynomial functions! These mathematical expressions are fundamental in algebra and have wide-ranging algebraic applications in various fields. Our introduction video serves as your gateway to understanding these powerful functions. As your virtual math tutor, I'm excited to guide you through this topic. Polynomial functions are expressions containing variables with non-negative integer exponents, combined using addition, subtraction, and multiplication. They can be as simple as a constant or as complex as a high-degree equation. The introduction video will break down the key concepts, helping you grasp the basics of polynomials, their properties, and how to work with them. You'll learn about terms, coefficients, and degrees, which are essential building blocks for more advanced mathematical concepts. By the end of this introductory section, you'll have a solid foundation to explore more complex aspects of polynomial functions. Let's embark on this mathematical journey together!
Recognizing a Polynomial Function
Which of the following are not polynomial functions? Explain.
f(x)=5x2+4x−3x−1+2
To determine whether a given function is a polynomial function, we need to understand the basic characteristics of polynomial functions. A polynomial function is an expression that consists of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. The general form of a polynomial function is:
P(x)=anxn+an−1xn−1+...+a1x+a0
where an,an−1,...,a1,a0 are coefficients and n is a non-negative integer.
Let's analyze the given function f(x)=5x2+4x−3x−1+2. We need to check each term to see if it fits the criteria of a polynomial function.
1. The first term is 5x2. Here, the coefficient is 5 (a real number) and the exponent is 2, which is a positive integer. This term fits the criteria of a polynomial function.
2. The second term is 4x. The coefficient is 4 (a real number) and the exponent is 1 (since x is the same as x1), which is a positive integer. This term also fits the criteria of a polynomial function.
3. The third term is −3x−1. The coefficient is -3 (a real number), but the exponent is -1, which is not a positive integer. This term does not fit the criteria of a polynomial function.
4. The fourth term is 2. This is a constant term with no variable, which can be considered as 2x0 where the exponent is 0 (a non-negative integer). This term fits the criteria of a polynomial function.
When determining if a function is a polynomial, the focus should be on the exponents of the variables. The coefficients can be any real number, but the exponents must be non-negative integers. In the given function f(x)=5x2+4x−3x−1+2, the term −3x−1 has an exponent of -1, which is not a positive integer.
Based on the analysis, the term −3x−1 disqualifies the function f(x)=5x2+4x−3x−1+2 from being a polynomial function. The explanation is that for a function to be considered a polynomial, all exponents of the variables must be non-negative integers. Since −1 is not a positive integer, the given function is not a polynomial function.
Q1: What is a polynomial function?
A polynomial function is an expression consisting of variables and coefficients, combined using only addition, subtraction, and multiplication operations. It's characterized by non-negative integer exponents of the variable. For example, f(x) = 2x³ + 3x² - 5x + 1 is a polynomial function.
Q2: What are examples of polynomial functions?
Examples of polynomial functions include:
- Linear: f(x) = 2x + 3
- Quadratic: g(x) = x² - 4x + 7
- Cubic: h(x) = 3x³ + 2x² - 5x + 1
- Constant: k(x) = 5
- Higher degree: p(x) = x - 2x³ + 3x - 1
Q3: How do you identify a polynomial function?
To identify a polynomial function:
1. Check if it only uses addition, subtraction, and multiplication.
2. Ensure all exponents are non-negative integers.
3. Verify that it contains only numerical coefficients and variables.
4. Confirm there are no division operations by variables.
If all these conditions are met, it's a polynomial function.
Q4: What is the degree of a polynomial function?
The degree of a polynomial function is the highest power of the variable in the polynomial. For example, in f(x) = 2x³ + 3x² - 5x + 1, the degree is 3. The degree helps determine the function's behavior and the maximum number of possible roots.
Q5: How do you solve a polynomial function?
Solving a polynomial function typically involves finding its roots or zeros. Methods include:
1. Factoring for lower-degree polynomials.
2. Using the quadratic formula for quadratic equations.
3. Applying synthetic division or the rational root theorem.
4. Using graphing calculators or computer software for higher-degree polynomials.
The specific method depends on the polynomial's degree and complexity.
Mastering polynomial functions requires a solid foundation in several key mathematical concepts. One of the most fundamental skills is solving two-step linear equations using addition and subtraction. This ability is crucial because it forms the basis for manipulating more complex polynomial equations. Understanding these basic operations helps students grasp how to isolate variables and solve for unknowns in polynomial functions.
Another critical prerequisite is solving polynomials with unknown coefficients. This skill is directly applicable to polynomial functions, as it teaches students how to work with variables in different positions within an equation. Recognizing and manipulating coefficients is essential when analyzing and solving polynomial functions of various degrees.
Equally important is the ability to factor polynomials, particularly those in the form x^2 + bx + c. Factoring is a fundamental technique used to simplify and solve polynomial equations. It allows students to find the roots of polynomial functions and understand their behavior. This skill is indispensable when graphing polynomial functions and determining their characteristics.
Lastly, determining the equation of a polynomial function is a crucial skill that ties all these concepts together. This ability requires students to synthesize their understanding of polynomial structure, coefficients, and factoring to construct and analyze polynomial functions from given information.
Each of these prerequisite topics builds upon the others, creating a comprehensive understanding of polynomial functions. The ability to perform addition, subtraction, and multiplication operations forms the foundation for manipulating polynomial expressions. Understanding coefficients in polynomials is essential for recognizing the structure and behavior of different polynomial functions. Familiarity with the standard form of polynomials enables students to quickly identify the degree and leading terms of a function. Finally, the skill of polynomial function classification allows for a deeper analysis of function behavior and characteristics.
By mastering these prerequisite topics, students will be well-equipped to tackle more advanced concepts in polynomial functions. They will be able to graph, analyze, and solve complex polynomial equations with confidence. Moreover, this foundational knowledge will prove invaluable in higher-level mathematics courses and real-world applications where polynomial functions are frequently encountered.
