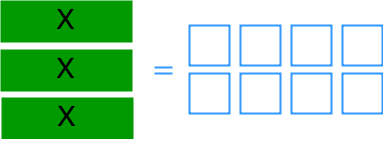
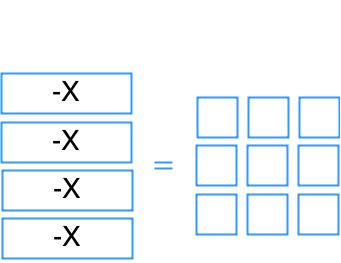
Mastering One-Step Linear Equations: ax = b and x/a = b Unlock the power of one-step linear equations! Learn to solve ax = b and x/a = b with confidence. Gain essential algebra skills through clear explanations, step-by-step solutions, and real-world applications.
Free to Join!
Easily See Your Progress
 We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.
We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.Make Use of Our Learning Aids
Earn Achievements as You Learn
 Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.
Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.Create and Customize Your Avatar
 Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Introduction to One-Step Linear Equations
One-step linear equations are fundamental in algebra, serving as a gateway to more complex mathematical concepts. Our introduction video provides a crucial foundation for understanding these equations, making it an essential starting point for learners. This article delves into two primary forms of one-step linear equations: ax = b and x/a = b. These equations are called "one-step" because they require only a single operation to solve for the unknown variable. The ax = b form involves multiplication or division to isolate x, while x/a = b requires multiplication to eliminate the fraction. Mastering these basic equations is vital for progressing in algebra and tackling more advanced problems. By understanding the principles behind one-step linear equations, students develop critical thinking skills and lay the groundwork for solving more complex mathematical challenges. The simplicity of these equations makes them an ideal starting point for beginners in algebra.
What is the equation represented by each diagram?
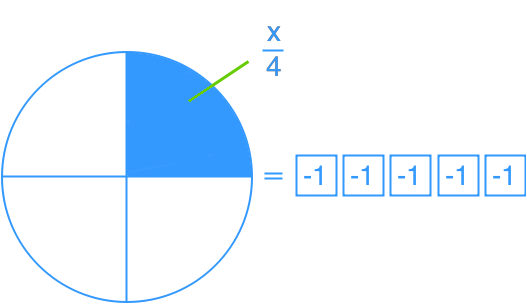
Step 1: Understanding the Diagram
To solve the equation represented by the diagram, we first need to understand what each part of the diagram represents. The large circle in the diagram represents an unknown amount, which we typically denote as a variable, usually "x". This unknown amount is divided into four equal parts, indicating that the large circle is divided by 4.
Step 2: Identifying the Variable
Since the large circle represents an unknown amount, we can call this unknown amount "x". Therefore, the division of this circle into four parts can be represented as x/4. This is the left side of our equation.
Step 3: Understanding the Algebra Tiles
The diagram also includes five white algebra tiles. In algebra, white tiles typically represent negative values, while colored tiles represent positive values. Each white tile represents -1. Since there are five white tiles, this part of the diagram represents -5. This is the right side of our equation.
Step 4: Formulating the Equation
Now that we have identified both parts of the diagram, we can formulate the equation. The left side of the equation is x/4, and the right side of the equation is -5. Therefore, the equation represented by the diagram is:
x/4 = -5
Step 5: Solving the Equation
To solve the equation x/4 = -5, we need to isolate the variable x. This involves undoing the division by 4. To do this, we multiply both sides of the equation by 4. This step ensures that we maintain the equality of the equation.
Multiplying both sides by 4, we get:
4 * (x/4) = 4 * (-5)
The 4s on the left side cancel out, leaving us with:
x = -20
Step 6: Verifying the Solution
To ensure our solution is correct, we can verify it by substituting x = -20 back into the original equation. If the left side equals the right side, our solution is correct.
Substituting x = -20 into x/4 = -5, we get:
-20/4 = -5
Since -20 divided by 4 is indeed -5, our solution is verified.
Conclusion
By following these steps, we have successfully identified and solved the equation represented by the diagram. The key steps involved understanding the diagram, identifying the variable, formulating the equation, solving the equation, and verifying the solution. This method ensures a clear and accurate solution to one-step linear equations.
Here are some frequently asked questions about one-step linear equations:
1. What is a linear equation with one example?
A linear equation is an equation where the variable has an exponent of 1. For example, 2x + 5 = 13 is a linear equation. The variable x is raised to the first power, and the equation forms a straight line when graphed.
2. How to solve step equations?
To solve one-step equations, follow these steps: 1) Identify the operation (addition, subtraction, multiplication, or division). 2) Perform the inverse operation on both sides of the equation. 3) Simplify to isolate the variable. For example, to solve x + 3 = 7, subtract 3 from both sides: x = 7 - 3 = 4.
3. How do you solve linear equations 1?
To solve linear equations with one variable: 1) Isolate the variable on one side of the equation. 2) Use inverse operations to remove constants or coefficients. 3) Simplify the equation. 4) Check your answer by substituting it back into the original equation.
4. What are simple linear equations?
Simple linear equations are equations that can be solved in one step. They typically involve one operation, such as addition, subtraction, multiplication, or division. Examples include x + 5 = 12, 3y = 15, or z/4 = 6.
5. How to Solve One-Step Equations?
To solve one-step equations: 1) Identify the operation being performed on the variable. 2) Perform the inverse operation on both sides of the equation. 3) Simplify to find the value of the variable. For example, to solve 3x = 12, divide both sides by 3: x = 12 ÷ 3 = 4.
Understanding the foundations of algebra is crucial when tackling one-step linear equations. Before diving into solving equations like ax = b or x/a = b, it's essential to grasp several key concepts that form the building blocks of algebraic problem-solving.
One of the fundamental skills required is solving linear equations using multiplication and division. This forms the core of manipulating one-step equations, allowing you to isolate the variable efficiently. Closely related to this is the concept of inverse operations in equations, which is crucial for maintaining equality while solving for the unknown.
When working with equations, it's important to understand the role of coefficients in equations. This knowledge helps in interpreting the 'a' in ax = b and recognizing its impact on the solution. Additionally, being familiar with the order of operations in equations ensures that you approach the problem-solving process systematically.
Real-world applications often require translating word problems into equations. This skill is invaluable when you encounter practical scenarios that need to be modeled as one-step linear equations. Similarly, solving for variables in context, such as in distance-time problems, reinforces the practical utility of these equations.
As you progress, you'll find that checking solutions in equations becomes an essential part of the problem-solving process. This verification step ensures the accuracy of your work and deepens your understanding of equation properties.
By mastering these prerequisite topics, you'll build a strong foundation for tackling one-step linear equations. Each concept contributes to your overall comprehension, making the process of solving ax = b or x/a = b more intuitive and manageable. Remember, algebra is a step-by-step journey, and each prerequisite topic serves as a crucial stepping stone towards mastery of more complex algebraic concepts.