Sign In
Sign Up
flag-us

Real-Life Examples and Applications of Diffraction
Discover how diffraction shapes our world, from rainbow effects on CDs to advanced technologies in telecommunications and medical imaging. Explore everyday examples and cutting-edge applications.

Now Playing:Application of diffraction – Example 0a
Intros
  1. Application of Diffraction
  2. Application of Diffraction
    Introduction to applications of refraction
  3. Application of Diffraction
    Hologram and Resolving Power of lenses
Examples
  1. A good diffraction grating has 2.6 x 103 lines per centimeters. What is the distance between two lines in the grating?
    Fundamentals of light
    Jump to:NotesConceptFAQsPrerequisites
    Notes

    In this lesson, we will learn:

    • Transmission Gratings
    • Reflecting Gratings
    • Holograms
    • Resolving Power of lenses

    Notes:

    Transmission Gratings

    • As narrow beam of light passes through a narrow slit, it shows wave light interference patterns. Light and dark bands are formed on the screen but they are kind of blended. In order to have more defined spots we use diffraction grating. A diffraction grating includes number of wholes through which we will be able to see bright spots that are separated by dark bands more clearly.

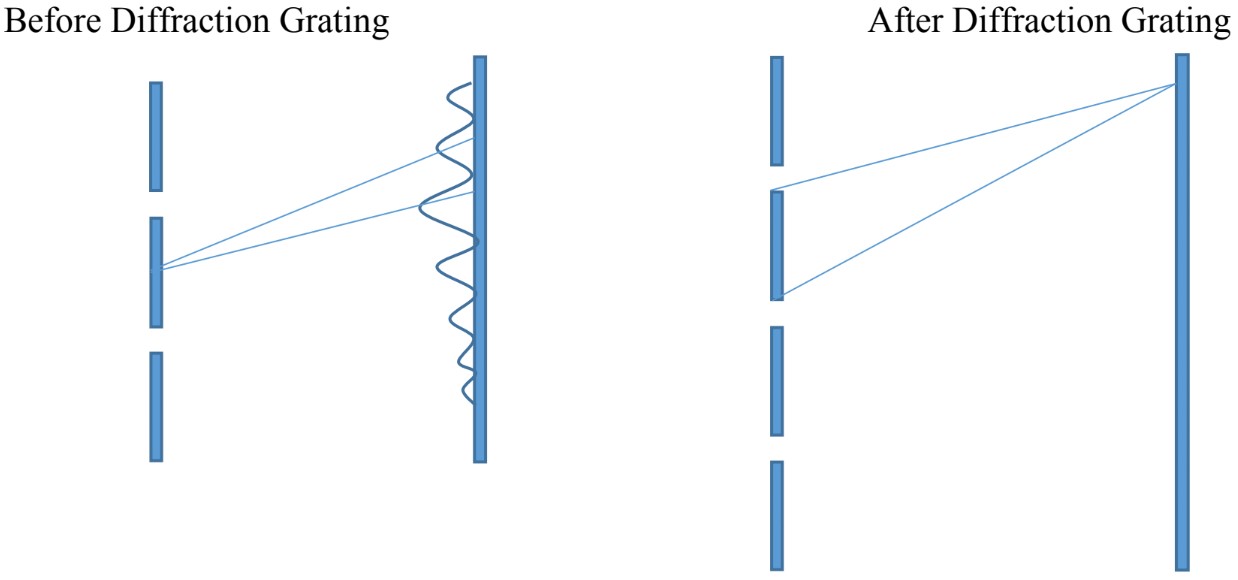
    Mirrors
    • Diffraction Gratings
    • A diffraction grating is a device that transmits or reflects light and forms an interference pattern in the same way that a double slit does.
    • Diffraction gratings are made by scattering very fine lines with a diamond point on glass.
    • The space between the scattered lines act like slits
    • Same equation as double-slit interference holds for a diffraction grating, where d is the distance between the lines.
      Note: instead of measuring the distance from the central band to the first band, the angle θ \theta is calculated. XX is so smaller than LL, therefore, the distance to the screen is almost equal to the perpendicular distance LL, so the ratio of x/Lx/L can be replaced by sin θ \theta

      • Sinθ=x/L Sin \theta = x/L
      ⇒ Sinθ=λ/d ⇒ λ=d Sinθ \Rightarrow \,Sin \theta = \lambda/d \, \Rightarrow \, \lambda = d \, Sin \theta

      x/L=λ/d x/L = \lambda/d

      λ \lambda: Light wavelengt
      d d: Distance between two line
      θ \theta : The angle between first order of bright band

    Reflecting Gratings

    • They are produced by scribing fine lines on metallic or reflected glass surface.
    • Incoming light will be separated into different wavelengths
    • Different colors are separated
    • Refraction grating and interference produce the similar patterns

    Holograms

    • A form of Photography that produces a three-dimensional image
    • (credit cards holograms)
    • A coherent beam of light (laser light) is passed through a mirror and is divided into two beams
      1. The reference beam
      2. The object beam
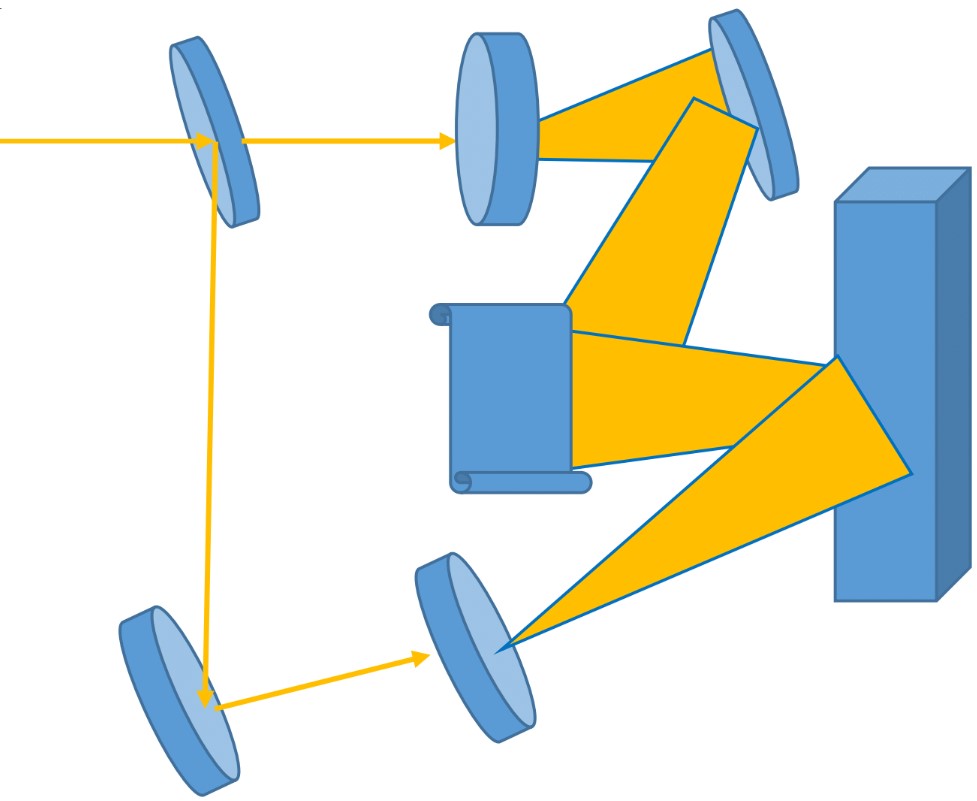
    Mirrors

    • The object beam is reflected from a mirror to illuminate the object
    • The beam in turn reflects from the object into photographic film
    • The reference beam is first reflected from a mirror, then it is spread by a lens and is directed over the object beam on the film
    • Intensity and relative phase of the light is recorded by interference pattern
    • When the film is developed the result is a hologram of the object

    Resolving Power of lenses

    • As light enters the lens of a telescope it passes through a circular hole, light gets diffracted.
    • Diffraction limits the ability of a lens to distinguish two closely spaced object.
    • The objective lens of a microscope cannot be enlarged, but the wavelength of light can be reduced.
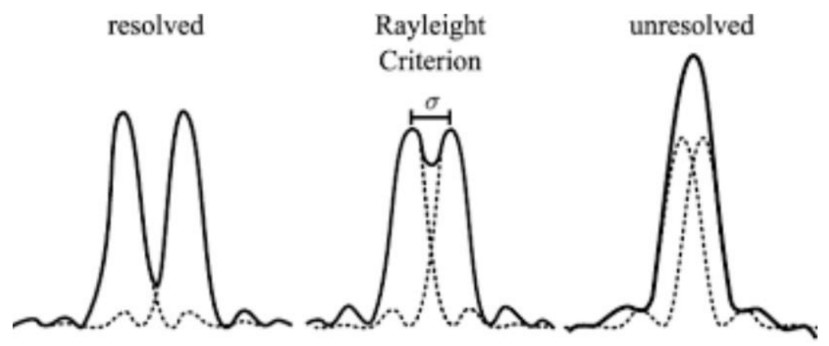
    Mirrors

    • Light coming from the stars will appear to be spread out, image looks blurry.
    • If the central bright band of one star falls on the first dark band of the second one, then the two stars are seen separately and clearly.
    • Increasing the size of the lens would affect the resolving power of the telescope, the Rayleigh criterion.
    Concept

    Introduction to Diffraction Applications

    Welcome to our exploration of diffraction applications! Diffraction, a fascinating phenomenon in wave physics, has numerous practical uses in our everyday lives. In our introduction video, we'll dive into key concepts like transmission grating, reflecting grating, holograms, and the resolving power of lenses. These applications showcase how diffraction impacts various fields, from optics to telecommunications. The video serves as an excellent starting point to understand how light behaves when it encounters obstacles or passes through small openings. By watching, you'll gain insights into how diffraction principles are used in creating spectacular visual effects, improving optical instruments, and even in securing documents. Whether you're a curious student or an aspiring scientist, this overview will provide a solid foundation for understanding the wide-ranging applications of diffraction in modern technology and scientific research. Let's embark on this illuminating journey together!

    FAQs

    1. What are real-life examples of diffraction?

      Real-life examples of diffraction include:

      • The rainbow-like effect on CD/DVD surfaces
      • Colorful patterns on soap bubbles
      • Iridescent colors on butterfly wings
      • The spread of ocean waves around obstacles
      • The bending of sound waves around corners

    2. How is diffraction used in everyday life?

      Diffraction is used in various everyday applications, including:

      • Optical fiber communications for internet and phone services
      • Security holograms on credit cards and banknotes
      • Spectroscopy for chemical analysis in laboratories
      • X-ray crystallography in medical imaging
      • Noise-canceling headphones that use sound wave interference

    3. What is an example of diffraction of sound in everyday life?

      A common example of sound diffraction is hearing someone speak from around a corner or through a doorway. Sound waves bend around obstacles, allowing us to hear sounds even when there's no direct line of sight to the source. This principle is also used in the design of concert halls and auditoriums to ensure optimal sound distribution.

    4. What is an example of diffraction grating in real life?

      A real-life example of diffraction grating is the iridescent surface of a CD or DVD. When light hits the closely spaced tracks on the disc's surface, it diffracts into its component colors, creating a rainbow-like effect. This principle is also used in spectroscopes for scientific analysis and in some types of solar panels to improve light absorption efficiency.

    5. How does diffraction affect telescope and microscope performance?

      Diffraction affects the resolving power of telescopes and microscopes, limiting their ability to distinguish between closely spaced objects. This is known as the diffraction limit. To improve performance, scientists use larger apertures in telescopes and shorter wavelengths of light (or electron beams) in microscopes. Advanced techniques like adaptive optics and super-resolution microscopy help overcome these limitations, allowing for clearer and more detailed observations.

    Prerequisites

    Understanding the application of diffraction requires a solid foundation in key physics concepts. One crucial prerequisite topic is diffraction and interference of light. This fundamental concept is essential for grasping how diffraction applies to various real-world scenarios and technological advancements.

    Diffraction, the bending of waves around obstacles or through openings, is a phenomenon that plays a significant role in many applications. To fully appreciate these applications, students must first comprehend the principles of diffraction and how it relates to light interference patterns. This understanding forms the basis for exploring more complex applications in fields such as optics, telecommunications, and medical imaging.

    When studying the diffraction and interference of light, students learn about wave properties, wavelength, and how light behaves when encountering obstacles or passing through small apertures. These concepts directly translate to practical applications of diffraction. For instance, in optical systems, diffraction limits the resolution of microscopes and telescopes, making it crucial for designers to account for these effects.

    Moreover, the study of light interference patterns provides insights into how diffraction gratings work. These gratings are used in spectroscopy, allowing scientists to analyze the composition of distant stars or identify chemical compounds. Without a solid grasp of the prerequisite topic, students might struggle to understand how these applications leverage the principles of diffraction.

    In the field of telecommunications, diffraction plays a vital role in antenna design and signal propagation. Engineers must consider how radio waves diffract around buildings and terrain to optimize network coverage. This application directly builds upon the foundational knowledge of how waves behave when encountering obstacles, a key aspect of the diffraction and interference of light topic.

    Medical imaging techniques, such as X-ray crystallography, also rely heavily on diffraction principles. By understanding how X-rays diffract through crystal structures, scientists can determine the atomic and molecular structure of materials. This application demonstrates the far-reaching implications of mastering the prerequisite concepts related to diffraction.

    In conclusion, the application of diffraction in various fields becomes much more accessible and comprehensible when students have a strong foundation in the prerequisite topic of diffraction and interference of light. This knowledge not only enhances understanding but also fosters creativity in applying these principles to solve real-world problems and drive technological innovation.