Diffraction and interference of light

In this lesson, we will learn:

• Young’s two-slit experiment and diffraction of light
• Interference fringes
• Coherent waves
• Diffraction of white light
• Measuring the wavelength of light wave
• Single- slit diffraction

Notes:

Young’s two-slit experiment and diffraction of light

A directed beam of light at two closely spaced narrow slits in a barrier gets diffracted and rays from two slits overlap. A pattern of bright and and dark bands can be seen on the screen.



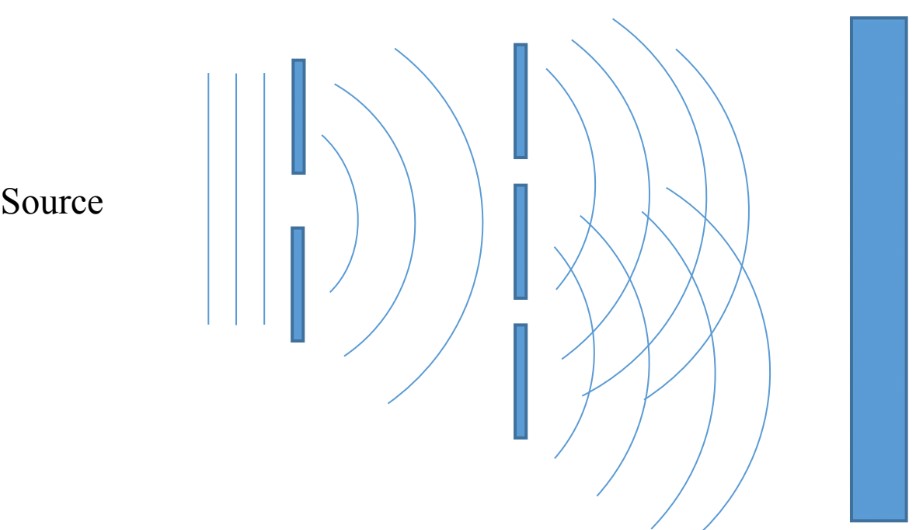
Interference Fringes

The bright and dark bands that can be seen on the screen as the result of light interference. The bands are the result of constructive (crests overlap) and destructive (crests meet troughs) interference of light waves form the two slits.

Coherent Waves

A narrow slit is placed in front of a monochromatic light (light with only one wavelength) produces coherent waves. Crests reach the same point at the same time as do the troughs.
Diffraction of monochromatic light results in a bright central band on the screen as the result of constructive interference, other bright bands are placed on either sides. Between the bright bands dark areas are located as the result of destructive interference.

Diffraction of white light

When white light is used in a double-slit experiment, diffraction causes the appearance of colored spectra instead of bright and dark bands.

Measuring the wavelength of light wave

The wavelength of light waves using double-slit interference can be measured using:

$\large \lambda = \frac{xd}{L}$

$x =$distance between the central band and the first bright
$L =$ distance between the slits and the screen
$d =$ distance between two slits
$\lambda =$ wavelength

Single- slit diffraction

• In single-slit diffraction the x is the distance between the central bright band and the first dark band
• $x =$ $\large \frac{\lambda}{2}$
• $L = \frac{w}{2},$ (w is the width of the slit)
• $\frac{X}{L}$ $\large \frac{\lambda / 2} {w / 2} = \frac{\lambda}{w} \Rightarrow x = \frac{\lambda L} {w}$ (distance between the central bright band the and first dark band)