1. Home
  2. >Multivariable Calculus
  3. >Multiple Integral Applications
Help

Change in variables

All in One Place

Everything you need for JC, LC, and college level maths and science classes.

Learn with Ease

We’ve mastered the national curriculum so that you can revise with confidence.

Instant Help

24/7 access to the best tips, walkthroughs, and practice exercises available.

0/3
?
Intros
Lessons
  1. Change in Variables Overview:
  2. Transformations
    • Transformation = change one variable to another
    • Similar to u-substitution in integral calculus
    • xyxy-coordinate →\to uvuv-coordinate
    • An Example of Change in Variable of Regions
  3. Jacobian of a Transformation
    • Definition of Jacobian
    • Determinant of a 2 x 2 matrix
    • Deals with derivatives
  4. Change of Variables for a Double Integral
    • All xx's & yy's become uu's & vv's
    • Extra term is absolute value of Jacobian
    • An Example
0/6
?
Examples
Lessons
  1. Finding the Transformations
    Suppose we have  R\, R, where  R \, R \, is the region bounded by  y=x+2,y=−x\, y = x + 2, y = -x , and  y=x2\, y = \frac{x}{2} . Use the transformation  x=13u−13v  \, x = \frac{1}{3}u - \frac{1}{3}v \, and  y=13u+13v  \, y = \frac{1}{3}u + \frac{1}{3}v \, to determine the new region.
    1. Suppose we have  R\, R, where  R \, R \, is the region bounded by  y=1x,y=2x,x=2,x=4\, y = \frac{1}{x}, y = \frac{2}{x}, x = 2, x = 4 . Use the transformation  x=2u  \, x = 2u \, and  y=vu  \, y = \frac{v}{u} \, to determine the new region.
      1. Finding the Jacobian
        Given that the transformations are  x=2u+4v2  \, x = 2u + 4v^{2} \, and  y=u2−4v \, y = u^{2} - 4v , find the Jacobian.
        1. Given that the transformations are  x=u3v5 \, x = u^{3}v^{5} \, and  y=uv \, y = \frac{u}{v} , find the Jacobian.
          1. Changing the Variables & Integrating
            Evaluate  ∫∫Rx−ydA \, \int\int_{R} x - ydA \, where  R  \,R \, is the region bounded by  x24+y29=1  \, \frac{x^{2}}{4} + \frac{y^{2}}{9} = 1 \, using the transformation  x=2v,  \, x = 2v, \, and  y=3v \, y = 3v .
            1. Evaluate  ∫∫R2xydA \, \int\int_{R} 2xydA \, where  R  \,R \, is the region bounded by  xy=2,xy=4,x=2,x=4  \, xy = 2, xy = 4, x = 2, x = 4\, using the transformation  x=2u,  \, x = 2u, \, and  y=vu \, y = \frac{v}{u} .
              Topic Notes
              ?
              Notes:

              Transformations

              Recall that in Integral Calculus, we can change the variable xx to uu of an integral using u-substitution. In other words, we can change from

              ∫f(x)dx→∫f(u)du \int f(x)dx \to \int f(u)du

              We would like to do something similar like this with double integrals.

              Transformations is about changing from one variable to another. We will first start by transforming regions.


              Jacobian of a Transformation

              The Jacobian of a transformation x=g(u,v)x=g(u,v) & y=h(u,v)y=h(u,v) is the following:

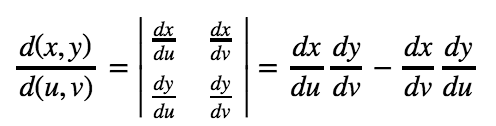
              jacobian of a transformation

              It is the determinant of a 2 x 2 matrix.

              Change of Variables for a Double Integral

              Suppose we want to integrate the function f(x,y)f(x,y) in the region RR under the transformation x=g(u,v)x=g(u,v) & y=h(u,v)y=h(u,v). Then the integral will now become:

              ∫∫Rf(x,y)dA=∫∫Sf(g(u,v),h(u,v))∣d(x,y)d(u,v)∣dudv\int \int_R f(x,y)dA = \int \int_S f(g(u,v), h(u,v)) \left| \frac{d(x,y)}{d(u,v)}\right| du dv