Using the pythagorean relationship

Introduction to Using the Pythagorean Relationship

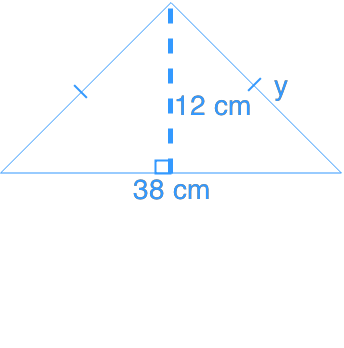
Welcome to our exploration of the Pythagorean theorem and its fascinating applications in compound polygons! This fundamental principle, a cornerstone of geometry, states that in a right-angled triangle, the square of the hypotenuse equals the sum of squares of the other two sides. It's not just a mathematical curiosity; it's a powerful tool used in various real-world scenarios. As we delve into compound polygons, you'll see how this theorem becomes even more versatile. Our introduction video is a great starting point, offering clear visuals and examples to help you grasp these concepts. Remember, understanding the Pythagorean theorem is key to solving complex geometric problems. Whether you're calculating distances, designing structures, or analyzing shapes, this principle will be your trusted companion. So, let's embark on this mathematical journey together, unraveling the mysteries of right-angled triangles and their role in more complex shapes. Get ready to see geometry in a whole new light!

Understanding the Pythagorean Theorem

Introduction to the Pythagorean Theorem

The Pythagorean theorem is a fundamental principle in geometry that has been captivating mathematicians and students alike for centuries. This theorem, named after the ancient Greek mathematician Pythagoras, establishes a crucial relationship between the sides of a right-angled triangle. At its core, the Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of squares of the other two sides.

The Formula: a² + b² = c²

The Pythagorean theorem is elegantly expressed by the formula a² + b² = c², where:

• a and b represent the lengths of the two shorter sides of the right-angled triangle (also known as the legs or catheti)
• c represents the length of the hypotenuse (the longest side of the triangle)

This simple yet powerful equation allows us to calculate the length of any side of a right-angled triangle when we know the lengths of the other two sides.

Historical Background

While the theorem bears Pythagoras' name, evidence suggests that the concept was known to several ancient civilizations before him. Babylonian mathematicians used it in practical applications as early as 1900 BCE. However, Pythagoras, who lived in the 6th century BCE, is credited with providing the first known mathematical proof of the theorem. This achievement revolutionized geometry and laid the groundwork for numerous mathematical developments that followed.

Pythagoras' Proof Using Squares and Triangles

Pythagoras' original proof, while ingenious, has been lost to time. However, one of the most elegant proofs attributed to him involves the use of squares and triangles. This proof demonstrates the theorem visually:

1. Start with a square with side length (a + b).
2. Inside this square, construct four congruent right-angled triangles, each with sides a, b, and c.
3. The remaining area in the center forms a square with side length c (the hypotenuse).
4. The area of the large square can be calculated in two ways:
   • (a + b)² = a² + 2ab + b²
   • 4(½ab) + c² = 2ab + c²
5. Equating these expressions and simplifying leads to a² + b² = c²

Practical Examples

Let's consider a few examples to illustrate the application of the Pythagorean theorem:

1. Finding the hypotenuse: In a right-angled triangle with sides 3 and 4 units long, we can find the hypotenuse:
   3² + 4² = c²
   9 + 16 = c²
   25 = c²
   c = 5 units
2. Determining if a triangle is right-angled: For a triangle with sides 5, 12, and 13 units:
   5² + 12² = 13²
   25 + 144 = 169
   169 = 169 (This equality confirms it's a right-angled triangle)

Applications in the Real World

The Pythagorean theorem finds applications in various fields beyond pure mathematics:

• Architecture and Construction: Ensuring buildings and structures are square and level
• Navigation: Calculating distances and plotting courses

Applying the Pythagorean Theorem

The Pythagorean theorem is a fundamental principle in geometry that relates the lengths of the right triangle sides. This theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of squares of the other two sides. Let's explore how to apply this theorem step-by-step to solve various problems.

Step 1: Understanding the Formula

The Pythagorean theorem is expressed as a² + b² = c², where:

• a and b are the lengths of the two shorter sides (legs) of the right triangle
• c is the length of the hypotenuse (the longest side)

Step 2: Solving for the Hypotenuse

To find the length of the hypotenuse when you know the lengths of the other two sides:

1. Square the length of each known side
2. Add these squared values
3. Calculate the square root of the sum

Example: If a = 3 and b = 4, then:

c² = 3² + 4² = 9 + 16 = 25

c = 25 = 5

Step 3: Solving for a Leg

To find the length of one of the shorter sides when you know the hypotenuse and the other side:

1. Rearrange the formula to a² = c² - b²
2. Substitute the known values
3. Solve for the unknown side

Example: If c = 5 and b = 4, then:

a² = 5² - 4² = 25 - 16 = 9

a = 9 = 3

Step 4: Practical Applications

The practical applications of Pythagorean theorem have numerous real-world applications. Here are some examples:

• Construction: Calculating the length of a roof rafter
• Navigation: Determining the shortest distance between two points
• Sports: Measuring field dimensions or calculating trajectories

Step 5: Solving a Practical Problem

Let's solve a real-world problem using the Pythagorean theorem:

A ladder needs to reach a window 12 feet above the ground. The base of the ladder must be placed 5 feet away from the building for safety. How long should the ladder be?

1. Identify the known sides: a = 12 feet (height), b = 5 feet (distance from wall)
2. Use the formula: c² = a² + b²
3. Substitute the values: c² = 12² + 5²
4. Calculate: c² = 144 + 25 = 169
5. Solve for c: c = 169 13 feet

Step 6: Verifying Solutions

Always check your answers by plugging them back into the original equation. This helps ensure accuracy and reinforces understanding of the theorem.

Step 7: Practice with Various Scenarios

To master the application of the Pythagorean theorem, practice with different scenarios involving right triangle sides:

• Construction: Calculating the length of a roof rafter
• Navigation: Determining the shortest distance between two points
• Sports: Measuring field dimensions or calculating trajectories

Pythagorean Triples

Pythagorean triples are a fascinating concept in mathematics that have intrigued mathematicians and enthusiasts for centuries. These special sets of three positive integers have a unique property that makes them invaluable in geometry and various practical applications. At their core, Pythagorean triples are sets of numbers that satisfy the Pythagorean theorem, which states that in a right-angled triangle, the square of the length of the hypotenuse (the longest side) is equal to the sum of squares of the other two sides.

The most well-known Pythagorean triple is 3-4-5. In this case, 3² + 4² = 5², or 9 + 16 = 25. This triple corresponds to a right-angled triangle with sides of lengths 3, 4, and 5 units. Another common example is the 5-12-13 triple, where 5² + 12² = 13², or 25 + 144 = 169. These are just two examples of an infinite set of Pythagorean triples that exist.

To verify if a set of numbers is a Pythagorean triple, one simply needs to apply the Pythagorean theorem. Let's take the triple 8-15-17 as an example. We can verify this by calculating: 8² + 15² = 64 + 225 = 289, which is indeed equal to 17² (289). This confirmation process is straightforward and can be applied to any set of three positive integers to determine if they form a Pythagorean triple.

The significance of Pythagorean triples in mathematics cannot be overstated. They provide a concrete, integer-based representation of the Pythagorean theorem, making it more accessible and applicable in various fields. In number theory, the study of Pythagorean triples has led to important discoveries and continues to be an area of active research. These triples also serve as a bridge between algebra and geometry, demonstrating how abstract mathematical concepts can have tangible geometric representations.

In practical applications, Pythagorean triples find use in numerous areas. In construction and carpentry, the 3-4-5 triple is often used to ensure right angles in building structures. Surveyors and engineers utilize these triples for accurate measurements and in creating precise right angles in large-scale projects. In computer graphics and game development, Pythagorean triples are employed to create realistic 3D environments and calculate distances efficiently.

The concept of Pythagorean triples extends beyond basic mathematics and finds applications in advanced fields such as cryptography and computer science. The properties of these triples are used in certain encryption algorithms and in solving complex computational problems. Additionally, the study of Pythagorean triples has led to the development of more advanced mathematical concepts, including elliptic curve theory, which has significant implications in modern cryptography.

Understanding Pythagorean triples also provides a gateway to exploring more complex mathematical ideas. For instance, the search for patterns in generating these triples has led to the discovery of formulas that can produce an infinite number of such triples. This exploration touches on aspects of number theory, algebra, and even calculus, making Pythagorean triples a rich topic for mathematical investigation at various levels of education.

In conclusion, Pythagorean triples represent a perfect blend of simplicity and profound mathematical significance. From their basic definition as sets of numbers satisfying a² + b² = c² to their wide-ranging applications in mathematics and real-world scenarios, these triples continue to fascinate and inspire. Whether used in practical measurements, theoretical mathematics, or as a teaching tool, Pythagorean triples remain a fundamental and intriguing concept in the world of mathematics, bridging ancient wisdom with modern applications.

Applying the Pythagorean Theorem to Compound Polygons

The Pythagorean theorem is a fundamental principle in geometry, typically used for right triangles. However, its application extends far beyond simple triangles, proving invaluable when dealing with complex geometric shapes and compound polygons. In this section, we'll explore how to leverage the Pythagorean theorem in more intricate scenarios, providing step-by-step solutions to problems involving multiple right triangles within larger shapes.

When working with compound polygons, the key is to identify the right triangles within the larger shape. These triangles may not be immediately apparent, but breaking down the complex shape into simpler components is crucial for problem-solving. Let's consider some examples to illustrate this process.

Example 1: L-shaped polygon
Imagine an L-shaped polygon with dimensions 3 units, 4 units, and 5 units for the outer edges, and 1 unit for the inner corner. To find the diagonal distance across this shape, we need to: 1. Identify the right triangle formed by the diagonal and the outer edges. 2. Calculate the lengths of the legs of this larger right triangle using the Pythagorean theorem on smaller right triangles within the shape. 3. Apply the Pythagorean theorem to the larger triangle to find the diagonal.

Step-by-step solution: 1. The diagonal forms a right triangle with legs of (3+1) and (4+1) units. 2. Calculate the hypotenuse of the smaller right triangle (3-4-5): 5² = 3² + 4² (25 = 9 + 16) 3. Now we have a larger right triangle with legs 4 and 5 units. 4. Apply the Pythagorean theorem: d² = 4² + 5² = 16 + 25 = 41 5. The diagonal length is 41 6.40 units

Example 2: Trapezoid with a perpendicular bisector
Consider a trapezoid with parallel sides of 8 and 12 units, a height of 5 units, and a perpendicular bisector from the longer parallel side to the opposite side. To find the length of this bisector: 1. Identify the right triangles formed by the bisector. 2. Use the Pythagorean theorem to find the length of half the difference between parallel sides. 3. Apply the theorem again to calculate the bisector length.

Step-by-step solution: 1. The difference between parallel sides is 4 units, so each right triangle has a base of 2 units. 2. Use the Pythagorean theorem for the right triangle formed by half the trapezoid: x² = 2² + 5² = 4 + 25 = 29 3. The length of the perpendicular bisector is 29 5.39 units

Example 3: Irregular hexagon
Imagine an irregular hexagon formed by combining a rectangle and two equilateral triangles on opposite sides. If the rectangle is 6 units by 8 units and the equilateral triangles have sides of 4 units, find the distance between the top and bottom vertices of the hexagon. 1. Break down the shape into right triangles. 2. Calculate the height of the equilateral triangles. 3. Sum the heights and apply the Pythagorean theorem to find the total distance.

Step-by-step solution: 1. For each equilateral triangle, form a right triangle by drawing a perpendicular bisector. 2. Calculate the height of each equilateral triangle: h² = 4² - 2² = 16 - 4 = 12, so h = 12 3.46 units 3. Total vertical distance = 6 + (2 × 3.46) = 12.

Real-World Applications of the Pythagorean Theorem

The Pythagorean theorem, a fundamental principle in mathematics, has numerous real-world applications that extend far beyond the classroom. This ancient mathematical concept, which states that the square of the hypotenuse of a right triangle is equal to the sum of the squares of the other two sides, plays a crucial role in various fields such as construction, navigation, and sports. Understanding how this theorem is applied in everyday situations can enhance problem-solving skills and decision-making abilities.

In the construction industry, the Pythagorean theorem is an indispensable tool for ensuring structural integrity and accuracy. Builders and architects frequently use this principle to calculate distances, determine square footage, and create precise right angles. For instance, when framing a house, contractors employ the 3-4-5 triangle method, a practical application of the theorem, to ensure walls are perfectly square. This technique involves measuring 3 units along one side, 4 units along the perpendicular side, and confirming that the diagonal measures exactly 5 units, thus forming a right triangle. This simple yet effective method helps maintain the structural soundness of buildings and improves overall construction quality.

Navigation, both on land and at sea, heavily relies on the Pythagorean theorem. In maritime navigation, sailors use this principle to calculate distances between two points on a map or chart. By treating the longitude and latitude differences as the two sides of a right triangle, navigators can determine the shortest distance between two locations, which is essential for efficient route planning and fuel conservation. Similarly, in aviation, pilots utilize the theorem to calculate flight paths and distances, ensuring safe and efficient air travel. GPS systems also incorporate the Pythagorean theorem in their algorithms to provide accurate location data and distance calculations for users worldwide.

The world of sports offers numerous examples of the Pythagorean theorem in action. In baseball, groundskeepers use the theorem to accurately lay out the diamond shape of the field. The distance from home plate to first base (90 feet) and from home plate to third base (90 feet) forms two sides of a right triangle, with the foul lines representing the hypotenuse. In soccer, the theorem helps determine the optimal placement of corner flags and ensures the field's dimensions are correct. Even in sports like golf, understanding the Pythagorean relationship can help players calculate distances and choose the right club for their shots.

Beyond these specific applications, the Pythagorean theorem serves as a powerful tool for everyday problem-solving and decision-making. For example, when rearranging furniture or planning home improvements, individuals can use the theorem to calculate room dimensions, determine the size of carpets or tiles needed, or even figure out if a large piece of furniture will fit through a doorway diagonally. In outdoor activities like hiking or landscaping, the theorem can help estimate distances or plan efficient paths. Understanding this mathematical principle allows people to approach spatial problems with greater confidence and accuracy.

The Pythagorean theorem's versatility extends to fields like computer graphics, where it's used to calculate distances between points in 2D and 3D spaces, crucial for rendering realistic images and animations. In physics and engineering, the theorem is fundamental in vector calculations, force analysis, and electrical circuit design. Even in fields like finance, the concept finds application in risk assessment models and portfolio diversification strategies. By recognizing the widespread applicability of this theorem, individuals can develop a more analytical approach to problem-solving in various aspects of life, from DIY projects to professional endeavors.

Conclusion

In this article, we've explored the fundamental principles of the Pythagorean theorem and its crucial role in geometry and problem-solving. We began with an introductory video that laid the groundwork for understanding this mathematical concept. The Pythagorean theorem's application extends far beyond simple right-angled triangles, proving invaluable in various real-world scenarios. We've seen how it can be used to calculate distances, determine unknown side lengths, and solve complex geometric problems. Remember, mastering the Pythagorean relationship is essential for advancing in mathematics and related fields. To solidify your understanding, we encourage you to practice applying the theorem to diverse problems. Try solving exercises that involve different triangle orientations and real-life applications. The more you work with the Pythagorean theorem, the more intuitive it will become. Don't hesitate to revisit the introductory video if you need a refresher, and keep exploring this fascinating mathematical principle to enhance your problem-solving skills. Remember, mastering the Pythagorean relationship is essential for advancing in mathematics and related fields.