Sign In
Try for Free
 flag-us

Surface area and volume of cones

Get the most by viewing this topic in your current grade. Pick your course now.

Now Playing:Surface area and volume of cones – Example 1a
Examples
  1. Find the surface area and the volume of the following cones:

    1. Surface area and volume of cones


    2. Surface area and volume of half spheres and cones

Surface area and volume of cones
Jump to:NotesPrerequisitesRelated
Notes
We just learned how to calculate the surface area and volume of pyramids and cylinders in the previous lessons. Now, we will look at cones - objects that have circle bases like cylinders and pointy tops like pyramids. Just like pyramids and cylinders, there are formulas for surface area and volume of cones. We will also try out some questions on composite solids that consist of cones too.

What is a cone?

Before we deal with calculations for the surface area and volume of cones, let's understand what a cone is first.

A cone is one of many geometric shapes. It has a flat surface on one of its ends and it comes to a point on the other end. The cones that we will be discussing in this section are right circular cones. What exactly is that? It's a cone that has a circle on its flat end, and a point that is 90 degrees from the center of the circle.

As we will be dealing a circle, be prepared to use pi and radius in our calculations. Take a moment to review this if you need before proceeding.

How to find the surface area of a cone

The surface area of a cone calculates the area surrounding the cone, and the surface of the circular base. We can easily find it with the following formula:

Surface  area=πr2+πrsSurface\;area = \pi r^2+ \pi rs

How would this work in a math problem?

Question:

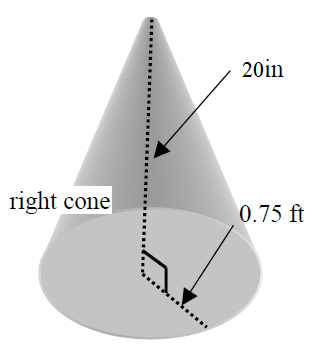
Find the surface area of the following cone.

Solution:

finding surface area and volume of a cone
a right cone

Surface area of cone

Surface  area=πr2+πrsSurface\;area = \pi r^2+ \pi rs

From the graph, we know the height (h) = 20in, radius (r) = 0.75ft. We need to find the slant (s) to calculate the surface area using the Pythagorean Theorem. But in order for us to do proper calculations, we'll have to convert the (h) and (s) into the same unit (inches) first. You can notice that one of the measurements is in feet, and one of them is in inches. To convert it, you'll get the following:

0.75ft=9m0.75ft = 9m

So we have height (h) = 20in, radius (r) = 9in. The slant is actually the hypotenuse of the right angle inside the cone. To find its length, we can now apply the Pythagorean Theorem:

S=92+202S=\sqrt{9^2+20^2}

S≈21.9inS\approx 21.9in

Lastly, we can plug in the numbers into the formula:

SA=π(9)2+π(9)(21.9)SA= \pi (9)^2+ \pi (9)(21.9)

SA≈875in2SA\approx 875in^2

The cone surface area is roughly 875in2875in^2

finding surface area of a cone
steps of finding surface area of a cone

How to find the volume of a cone

So how do we find the volume of a cone? The cone volume is a measurement of how much space there is inside a cone. There is a volume of a cone formula to help you find this:

Volume=13×(area  of  base)×hVolume= \frac{1}{3} \times (area\;of\;base) \times h

Let's try it out on an example. It's the same cone as the previous walkthrough, so you should be quite familiar with it already.

Question

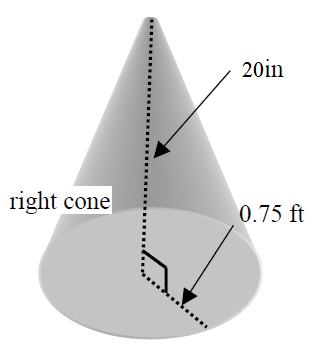
Find the volume of the following cone:

finding surface area and volume of a cone
a right cone

Surface area of cone

Solution

Volume of cone

Volume=13×(area  of  base)×hVolume= \frac{1}{3} \times (area\;of\;base) \times h

First, we need to calculate the area of the cone's base

Area  of  base=πr2Area\;of\;base=\pi r^2

Area  of  base=π(9)2Area\;of\;base=\pi (9)^2

Now, we can plug apply the numbers into the formula for volume of a cone

Volume=13×(π(9)2)×(20)Volume= \frac{1}{3} \times (\pi (9)^2) \times (20)

Volume≈1696.5in3Volume \approx 1696.5 in^3

finding volume of a cone
steps of finding volume of a cone

In this example, we didn't have to find an unknown for the volume, but there may be times where you'll be required to do so, such as when the slant is given, but we don't know the height. Always work on finding the unknowns you'll need for the different formulas first, whether it's for the volume or surface area of a cone.

You can take a look at more examples that illustrate a cone and its volume and surface area with this online click and drag cone resizer. This cone calculator can also help you double check your calculations.


equations of area,surface area,and volume of cones