What is slope?
The slope of a line is essentially how angled away from the horizontal a straight line is. In a chart such as the one below, the blue line is a straight line. Making use of the graph of a line, we can find its slope.
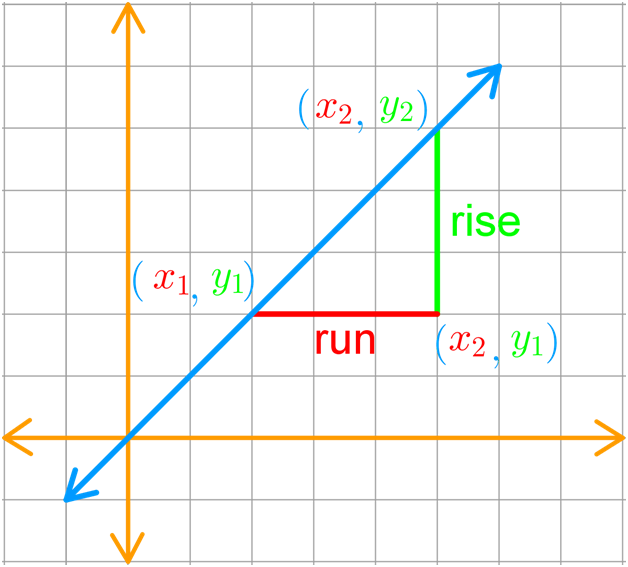
In order to find the slope of a line we require two points from the straight line. When you've got them, you can then put them into the slope formula to solve for the slope.
Formula for slope
The slope formula is quite simple and is as follows:
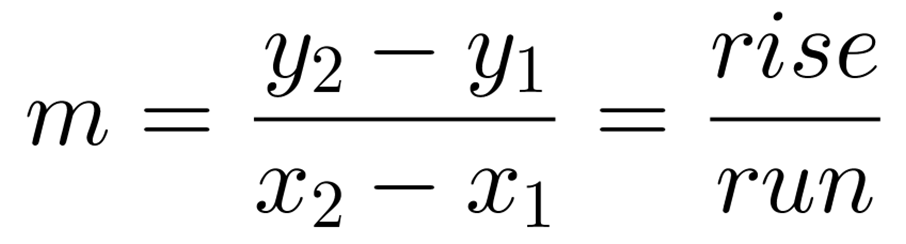
A slope definition is just rise over run. m denotes the slope, whereas the subscripts of 2 and 1 in the above 's and 's are used to differentiate between a point number "one" and a point number "two". There is no rule that indicates which point you should designate as your first point or your second point. As long as you subtract the values in the same order, with the 's on the top and the 's on the bottom, you'll get the same answer.
To prove this, we can take a look at the following:
If we take a point x1 and then subtract it by x2, it is the same as if we were to take -x2 and add it to x1. In the end, the two minus signs will cancel out, which shows that the order of the two points does not matter. The same can be done for y1 and y2. You will get the same answer for the slope no matter which point you use first and second, as long as you keep the points you designate as first and second consistent.
What does the slope mean?
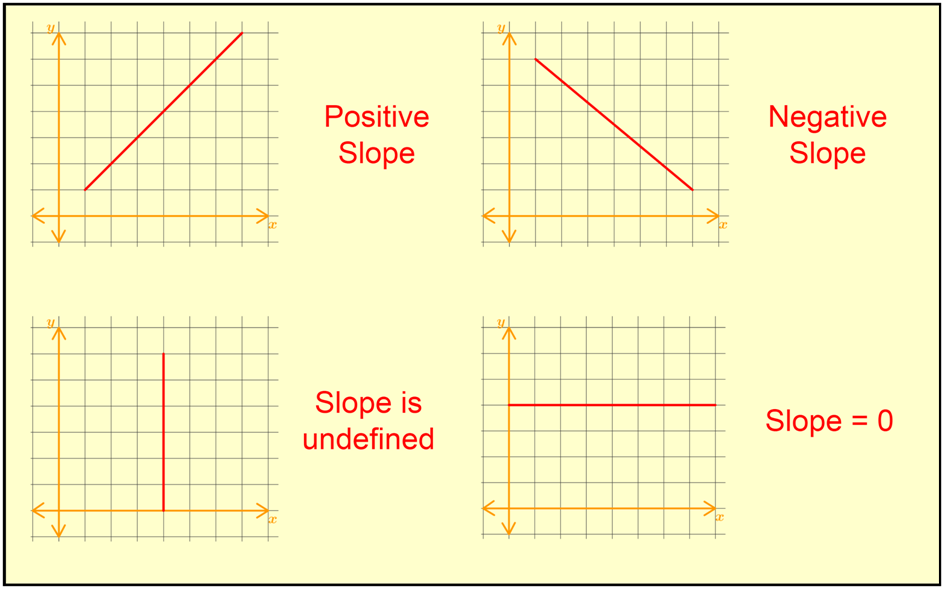
When a slope is positive, it indicates that a graph is increasing as the line progresses. If you get a negative result for a slope, it means that the line is trending downwards, and thus decreasing. This is relationship between the slope and the line that holds true at all times, so it can be useful when you need to double check your work. If you have a positive answer for a slope, look to see if the graph given is increasing. If not, you'll know that you must have made a mistake somewhere and should double check your answer.
How to find the slope of a line
What happens when a line is neither increasing nor decreasing?
For example, if the equation of the line is: , the equation will look like a horizontal line that is parallel to the -axis. There is no run, or rise.
In this case, you'll find that the slope is not positive or negative. The slope is actually zero! To test this, try it out on two points on the graph, and plug it into the slope formula.
For example,
Point 1:
Point 2:
The zero indicates that the graph is horizontal, and therefore the slope is neither increasing nor decreasing.
Now take the case of a vertical line. This is when a graph is, for example, . Would you say that the graph is increasing, or is it decreasing? In actual fact, a vertical line has no slope.
Let's look at this through an example using as our line, and the slope equation.
Point 1:
Point 2:
As we cannot divide by zero, the slope cannot be found. Thus, all vertical lines have no slope and this is true for all equations of vertical lines. You'll end up dividing by zero whenever you see an equation that looks like some number, as that's the equation of a vertical line.
A point to take note of is that a vertical line and horizontal line's slope does not mean the same thing. Having a slope of zero does not equal having no slope. Instead, horizontal lines do have a slope, and that is why when you calculate their slope with the slope equation, you'll get a number: zero.
If you're stuck on a problem or want to double check your work, feel free to reference this online slope calculator that makes use of the slope equation.