Rational zero theorem

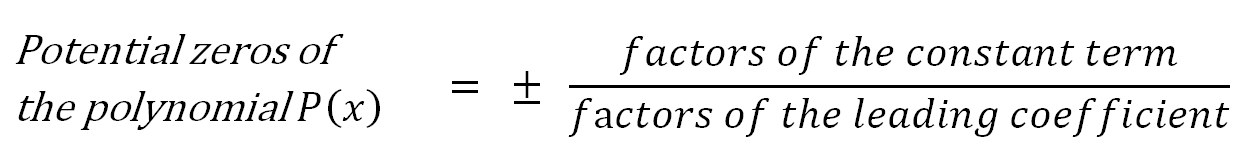
Introduction to the Rational Zero Theorem

The rational zero theorem, also known as the rational zero test or rational root test, is a fundamental concept in algebra that helps in finding zeros of polynomial functions. This powerful theorem provides a systematic approach to identifying potential rational roots of a polynomial equation. Our introduction video offers a comprehensive overview of this essential mathematical tool, making it easier for students to grasp its significance and application. By understanding the rational zero theorem, learners can efficiently narrow down the possible solutions of complex polynomial equations, saving time and effort in problem-solving. This theorem is particularly useful in higher-level mathematics and various scientific fields where polynomial functions play a crucial role. As we delve deeper into the rational zero theorem, you'll discover how it simplifies the process of finding roots and enhances your overall understanding of polynomial behavior.

Understanding the Rational Zero Theorem

The Rational Zero Theorem is a fundamental concept in algebra that provides a powerful method for finding potential zeros of polynomial equations. This theorem is particularly useful when dealing with polynomials that have integer coefficients. To fully grasp the importance of this theorem, we must first understand what rational roots are and why they play a crucial role in polynomial functions.

Rational zeros, also known as rational roots, are solutions to a polynomial equation that can be expressed as fractions (p/q) where p and q are integers, and q is not equal to zero. These zeros are significant because they represent the x-intercepts of the polynomial function's graph, which are points where the function crosses the x-axis.

The Rational Zero Theorem helps narrow down the possible rational zeros of a polynomial function, making the process of finding these zeros much more efficient. Instead of testing an infinite number of possibilities, the theorem provides a finite list of potential rational zeros to check.

The Rational Zero Theorem formula states that if a polynomial equation has integer coefficients, then any rational zero of the polynomial will be of the form ±p/q, where:

• p is a factor of the constant term (the term without a variable)
• q is a factor of the leading coefficient (the coefficient of the highest degree term)

To apply the Rational Zero Theorem, follow these steps:

1. Identify the constant term and find all its factors (including both positive and negative).
2. Identify the leading coefficient and find all its factors (positive only).
3. Create all possible fractions by dividing each factor of the constant term by each factor of the leading coefficient.
4. Test these potential zeros in the original polynomial equation.

For example, consider the polynomial function f(x) = 2x³ - 5x² - 14x + 8. The constant term is 8, and its factors are ±1, ±2, ±4, and ±8. The leading coefficient is 2, with factors 1 and 2. The potential rational zeros are:

±1/1, ±2/1, ±4/1, ±8/1, ±1/2, ±2/2, ±4/2, ±8/2

By testing these values, we can find the actual zeros of the polynomial function more efficiently than by using trial and error or other methods.

It's important to note that the Rational Zero Theorem only provides potential zeros. Not all of these candidates will necessarily be actual zeros of the polynomial. Additionally, the theorem does not account for irrational or complex zeros, which may also exist for some polynomial functions.

Understanding and applying the Rational Zero Theorem is crucial for students and professionals working with polynomial functions. It simplifies the process of finding zeros, which is essential for various applications in mathematics, physics, engineering, and other fields where polynomial models are used to describe real-world phenomena.

In conclusion, the Rational Zero Theorem is a powerful tool in the study of polynomial functions. By providing a systematic approach to identifying potential rational zeros, it significantly reduces the complexity of solving polynomial equations. Mastering this theorem enhances one's ability to analyze and work with polynomial functions efficiently, making it an indispensable skill in advanced mathematics and its applications.

Application of the Rational Zero Theorem

The Rational Zero Theorem is a powerful tool in algebra for finding the zeros of polynomial functions. This step-by-step guide will walk you through the process of applying this theorem, helping you efficiently identify potential rational zeros and solve polynomial equations.

Step 1: Identify the polynomial function
Begin by ensuring your polynomial is in standard form: a_nxⁿ + a_n-1x^n-1 + ... + a₁x + a₀ = 0, where a_n 0.

Step 2: List potential rational zeros
The Rational Zero Theorem states that if a polynomial equation with integer coefficients has a rational solution, it will be in the form ±p/q, where p is a factor of the constant term (a₀) and q is a factor of the leading coefficient (a_n). To list potential zeros:

• Find all factors of the constant term (p)
• Find all factors of the leading coefficient (q)
• Create fractions ±p/q for all combinations

Step 3: Test potential zeros
Use synthetic division or direct substitution to test each potential zero. This is where the trial and error aspect comes into play. However, you can make this process more efficient by:

• Starting with simpler fractions (e.g., ±1, ±2) before moving to more complex ones
• Using the Descartes' Rule of Signs to determine the number of positive and negative real zeros
• Applying the Intermediate Value Theorem to narrow down the range of potential zeros

Step 4: Factor the polynomial
As you find zeros, factor them out of the original polynomial. This reduces the degree of the polynomial, making subsequent steps easier.

Step 5: Repeat or conclude
Continue testing remaining potential zeros on the reduced polynomial until you've found all rational zeros or exhausted the list of potential zeros.

Example:
Let's apply the Rational Zero Theorem to the polynomial f(x) = 2x³ - 5x² - 14x + 8 = 0

Step 1: The polynomial is already in standard form.
Step 2: List potential zeros
Factors of constant term (8): ±1, ±2, ±4, ±8
Factors of leading coefficient (2): ±1, ±2
Potential zeros: ±1, ±2, ±4, ±8, ±1/2, ±1/4

Step 3: Test potential zeros
Starting with simpler options, let's test x = 1:
f(1) = 2(1)³ - 5(1)² - 14(1) + 8 = 2 - 5 - 14 + 8 = -9 0

Let's try x = 2:
f(2) = 2(2)³ - 5(2)² - 14(2) + 8 = 16 - 20 - 28 + 8 = -24 0

Testing x = -1:
f(-1) = 2(-1)³ - 5(-1)² - 14(-1)

Relationship with Polynomial Factoring

The Rational Zero Theorem plays a crucial role in polynomial factoring, providing a powerful tool for finding potential zeros of a polynomial equation. This theorem is intimately connected with polynomial long division, and synthetic division, forming a cohesive approach to solving complex polynomial problems.

At its core, the Rational Zero Theorem states that if a polynomial equation with integer coefficients has a rational solution, it will be in the form of ±p/q, where p is a factor of the constant term and q is a factor of the leading coefficient. This theorem narrows down the possible rational zeros to a finite list, significantly simplifying the factoring process.

When applied to polynomial factoring, the Rational Zero Theorem offers a systematic method for identifying potential factors. By testing the potential rational zeros, we can find actual zeros of the polynomial. Each zero corresponds to a linear factor of the polynomial, allowing us to break down the polynomial into smaller, more manageable pieces.

For example, consider the polynomial P(x) = 2x³ - 5x² - 14x + 8. The potential rational zeros according to the theorem are ±1, ±2, ±4, ±8, ±1/2, and ±1/4. By testing these values, we might find that x = 4 is a zero. This means (x - 4) is a factor of the polynomial, helping us progress in the factoring process.

The connection between the Rational Zero Theorem and polynomial long division becomes evident when we use the theorem to find a zero and then employ long division to factor out the corresponding linear term. Once we identify a zero, we can use polynomial long division to divide the original polynomial by (x - r), where r is the identified zero. This process not only confirms the zero but also produces the remaining factor of the polynomial.

Synthetic division, a shortcut method for polynomial division when dividing by a linear factor, further streamlines this process. It's particularly useful when working with the Rational Zero Theorem, as we often need to test multiple potential zeros. Synthetic division allows for quick evaluation of the polynomial at these potential zero points, making the overall factoring process more efficient.

To illustrate, let's continue with our example P(x) = 2x³ - 5x² - 14x + 8. Having found that 4 is a zero, we can use synthetic division to divide by (x - 4):

2 | -5 -14 8
8 12 -8
2 3 -2 0

This confirms that 4 is indeed a zero and gives us the remaining quadratic factor: 2x² + 3x - 2. We can further factor this quadratic to complete the factorization: 2x³ - 5x² - 14x + 8 = (x - 4)(2x + 4)(x - 1/2).

The synergy between the Rational Zero Theorem, polynomial factoring, and division techniques becomes particularly powerful when dealing with higher-degree polynomials. For a fourth-degree polynomial, finding one rational zero allows us to reduce it to a third-degree polynomial, making further factorization more manageable.

In practice, these concepts work together in a cyclical manner. The Rational Zero Theorem provides potential zeros, which we can test using synthetic division. When we find a zero, we use polynomial long division or synthetic division to factor out the corresponding linear term. This process continues until we've fully factored the polynomial or reduced it to a form that can't be factored further using these methods.

Understanding this relationship enhances problem-solving skills in polynomial algebra. It allows for a structured approach to factoring complex polynomials, breaking them down step-by-step into their constituent factors. This not only aids in solving polynomial problems but also in analyzing polynomial behavior, finding roots, and understanding the overall structure of polynomial functions.

In conclusion, the Rational Zero Theorem, when used in conjunction with polynomial factoring, long division, and synthetic division, forms a powerful toolkit for tackling polynomial problems. By leveraging these interconnected concepts, mathematicians and students alike can approach even the most complex polynomial problems with confidence.

Limitations and Considerations

The rational zero theorem is a powerful tool in algebra for finding potential rational roots of polynomial equations. However, it's crucial to understand its limitations and when to apply it effectively. One of the primary limitations of the rational zero theorem is that it only helps identify rational zeros, if they exist. Not all polynomials have rational zeros, and this fact has significant implications for problem-solving in mathematics.

Many students mistakenly believe that the rational zero theorem will always provide a complete solution to finding polynomial equations. This misconception can lead to frustration when dealing with polynomials that have irrational or complex zeros. For instance, the polynomial x² + 1 = 0 has no rational roots, and applying the rational zero theorem would not yield any useful results.

Another limitation is that even when a polynomial does have rational roots, the theorem only provides a list of potential candidates. It doesn't guarantee that any of these candidates are actual zeros, nor does it indicate how many zeros the polynomial might have. This means that after applying the theorem, one must still test each potential zero to determine if it's a true solution.

The rational zero theorem is most effective when dealing with polynomials that have integer coefficients and are likely to have rational roots. It's particularly useful in lower-degree polynomials where the number of potential rational zeros is manageable. However, as the degree of the polynomial increases, the list of potential zeros can become quite long, making the process of checking each candidate time-consuming.

In cases where a polynomial is known or suspected to have irrational zeros, other methods such as the intermediate value theorem, graphing techniques, or numerical approximation methods may be more appropriate. For polynomials of degree 3 or 4, specific formulas like the cubic or quartic formulas might be more efficient than the rational zero theorem.

It's also important to note that the rational zero theorem doesn't provide information about the multiplicity of zeros. A zero might appear in the list of potential candidates only once, but it could be a double or triple root of the polynomial. Additional techniques, such as synthetic division or analyzing the derivative of the polynomial, are needed to determine multiplicity.

When approaching polynomial problems, it's advisable to consider the context and nature of the problem before automatically applying the rational zero theorem. If the problem suggests or requires exact solutions and rational roots are likely, the theorem can be a valuable starting point. However, if approximate solutions are acceptable or if the polynomial coefficients are not integers, other methods might be more suitable.

In conclusion, while the rational zero theorem is a valuable tool in algebra, it's essential to recognize its limitations and use it judiciously. Understanding when to apply the theorem and when to explore alternative methods is key to efficient problem-solving in polynomial algebra. By being aware of these limitations and considerations, students and mathematicians can approach polynomial root-finding with a more comprehensive and effective strategy.

Practice Problems and Examples

Let's explore some practice problems that demonstrate the use of the rational zero theorem. We'll start with simpler examples and gradually increase the difficulty. Try to solve these problems on your own before checking the solutions!

Problem 1: Easy

Find the rational zeros of the polynomial: f(x) = x³ + 2x² - 5x - 6

Solution:

1. Identify potential rational zeros: Factors of the constant term (±1, ±2, ±3, ±6) divided by factors of the leading coefficient (±1).
2. Potential zeros: ±1, ±2, ±3, ±6
3. Use synthetic division to test each potential zero.
4. We find that 1, -2, and -3 are zeros of the polynomial.

Problem 2: Medium

Find the rational zeros of the polynomial: f(x) = 2x - 5x³ - 14x² + 23x + 6

Solution:

1. Potential rational zeros: Factors of 6 (±1, ±2, ±3, ±6) divided by factors of 2 (±1, ±2)
2. Potential zeros: ±1, ±1/2, ±2, ±3, ±3/2
3. Using synthetic division, we find that 1/2 and -3 are zeros.
4. Factor out (x - 1/2) and (x + 3) from the original polynomial.
5. The remaining quadratic factor can be solved using the quadratic formula.

Problem 3: Challenging

Find all zeros of the polynomial: f(x) = 3x + 7x - 13x³ - 17x² + 4x + 4

Solution:

1. Potential rational zeros: Factors of 4 (±1, ±2, ±4) divided by factors of 3 (±1, ±3)
2. Potential zeros: ±1, ±1/3, ±2/3, ±4/3, ±2, ±4/3
3. Using synthetic division, we find that 2/3 and -1 are rational zeros.
4. Factor out (3x - 2) and (x + 1) from the original polynomial.
5. The remaining cubic factor can be solved using additional techniques or graphing methods.

Remember, the rational zero theorem helps us identify potential rational roots, but it doesn't guarantee that all roots will be rational. In some cases, you may need to use additional methods to find irrational or complex roots.

Problem 4: Application

A rectangular garden has a length that is 2 meters longer than its width. If the area of the garden is 35 square meters, find the dimensions of the garden using the rational zero theorem.

Solution:

1. Let x be the width of the garden. Then the length is x + 2.
2. The area equation is: x(x + 2) = 35
3. Rearrange to standard form: x² + 2x - 35 = 0
4. Potential rational zeros: Factors of 35 (±1, ±5, ±7, ±35)
5. Using synthetic division, we find that 5 and -7 are zeros.

Conclusion

The rational zero theorem is a powerful tool in polynomial algebra, enabling us to find potential rational zeros of polynomial functions. This theorem significantly simplifies the process of solving polynomial equations by providing a finite list of possible rational solutions. Its importance extends beyond academic exercises, finding applications in various fields such as engineering, physics, and economics. The introductory video we discussed earlier offers a clear and concise explanation of the theorem, serving as an excellent starting point for understanding this concept. To truly master the rational zero theorem, regular practice with diverse polynomial problems is essential. We encourage you to explore related topics such as synthetic division, the factor theorem, and polynomial functions to deepen your understanding of polynomial functions. Remember, the rational zero theorem is just one piece of the puzzle in the fascinating world of polynomial algebra, and its mastery will greatly enhance your problem-solving skills in this domain.