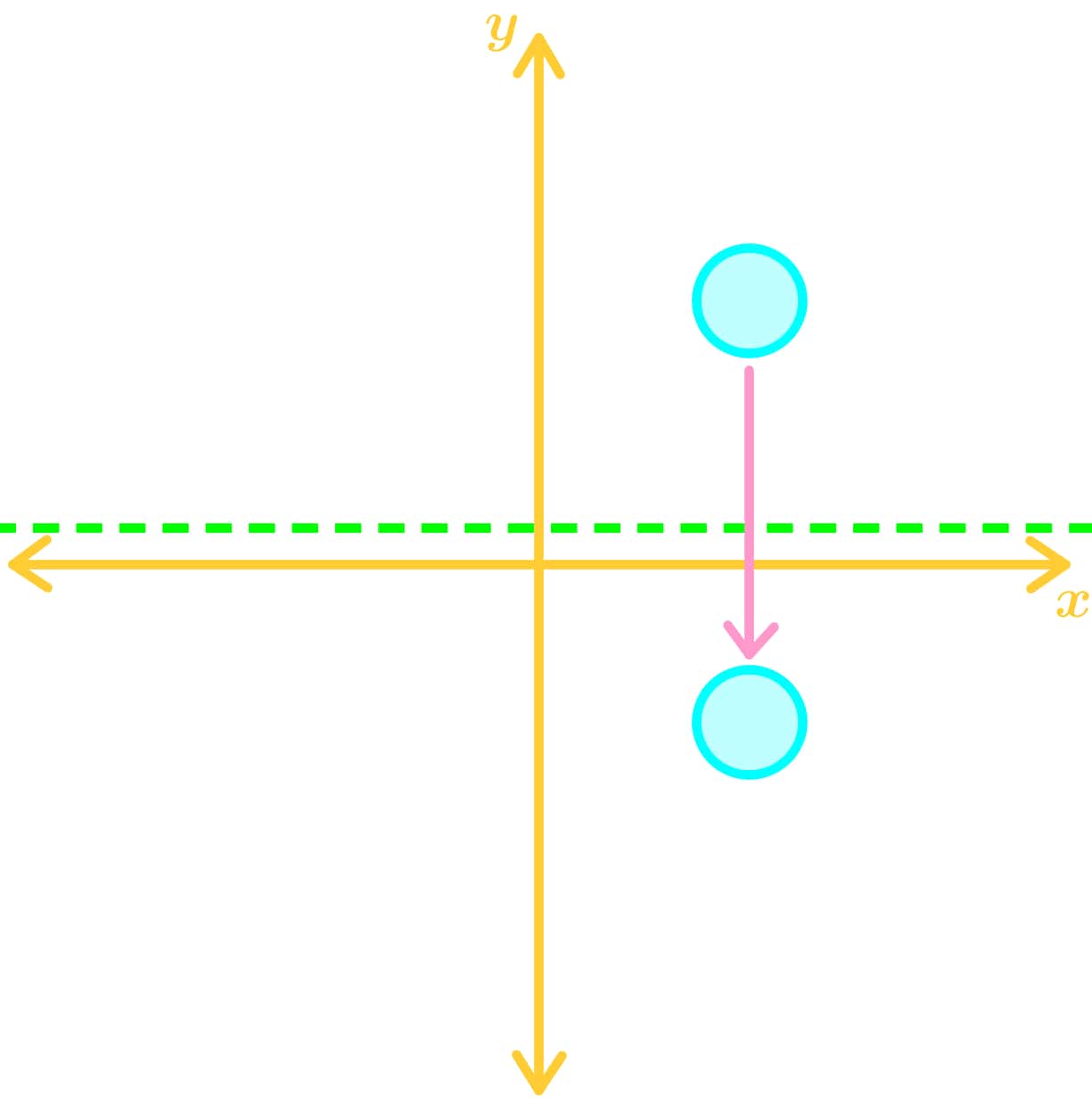
Given a picture or a description of the transformation, how do we find the transformation matrix? What we do is to take a look at the two unit vectors:
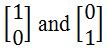
We want to ask ourselves how the transformation given in the question changes these two unit vectors.
Let's say that the unit vector
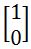 transforms from
transforms from 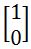 to
to 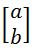 and the unit vector
and the unit vector 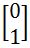 transforms from
transforms from 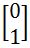 to
to 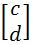 . Then we say that:
. Then we say that: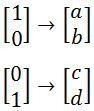
Then we combine these two column vectors into one matrix.
Hence, the transformation matrix is:
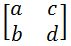

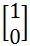 , scaled by a factor of 4
, scaled by a factor of 4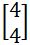 , scaled by a factor of
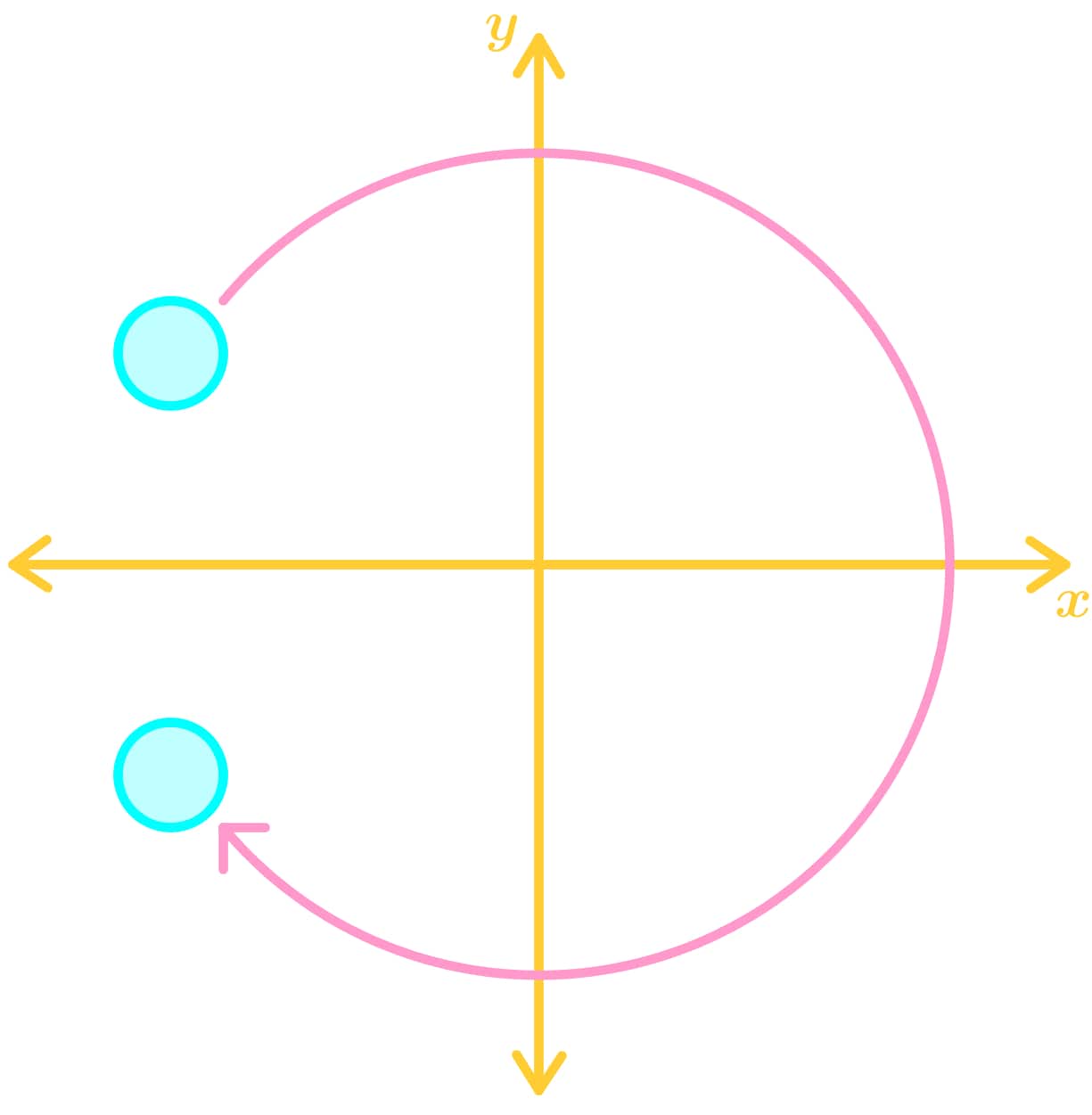
, scaled by a factor of 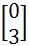 , rotated 90° counter-clockwise
, rotated 90° counter-clockwise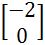 , rotated 270° clockwise
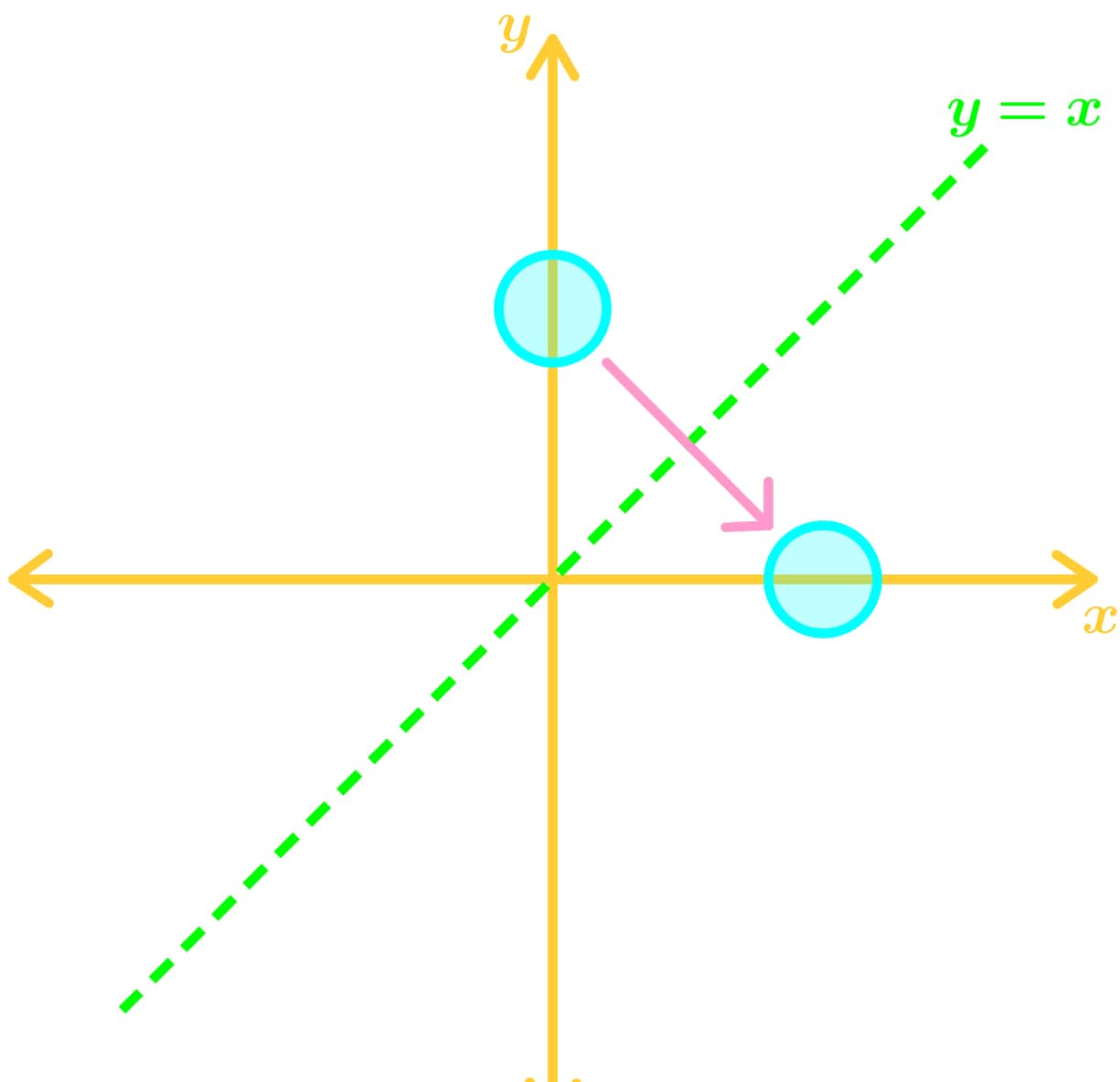
, rotated 270° clockwise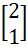 , reflected on the
, reflected on the 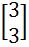 , reflected on the
, reflected on the