Theorems that are useful:
Pythagorean Theorem:
Trig ratio:
Law of sine:
Law of cosine:
Charlie leaves home for a bike ride, heading 040°T for 5km.
A camping group made a return journey from their base camp. From the camp, they first travelled 120°T for 3km. Then they travelled 210°T for 9km. Determine the direction and distance they need to travel if they want to return to the base camp now.
Melody and April go to the same school. Melody's home is 3.5km with a bearing of S16°W from school whilst April's home is 2.4km with a bearing of N42°E from school. How far away are their homes from each other?
Radar X detected an earthquake N55°E of it. 16km due east of Radar X, Radar Y detected the same earthquake N14°W of it.
A plane is sighted by Tom and Mary at bearings 028°T and 012°T respectively. If they are 2km away from each other, how high is the plane?
Consider the following diagram.
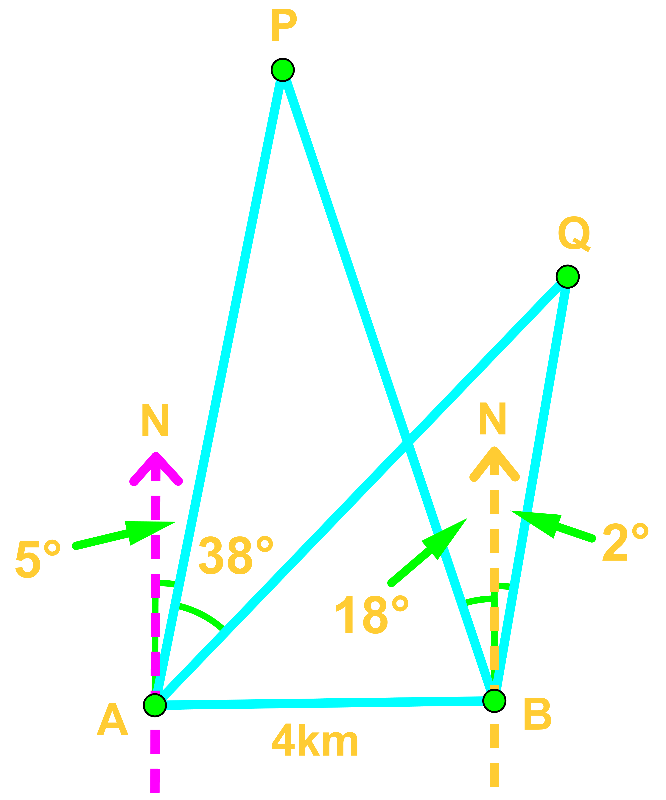
Find the distance between P and Q.