Mastering Order of Operations with Exponents: Your PEMDAS Guide Unlock the power of exponents in mathematical operations. Learn to apply PEMDAS effectively, solve complex problems, and boost your algebra skills with our comprehensive guide and practice exercises.
- Calculate:
- Calculate:
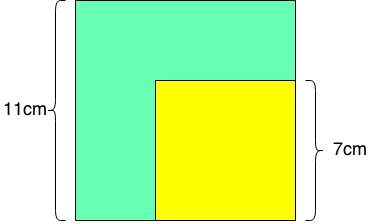
- A small yellow square is put on top of a large green square. The side length of the yellow square is 7 cm, whereas the side length of the green square is 11 cm.
Free to Join!
Easily See Your Progress
 We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.
We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.Make Use of Our Learning Aids
Earn Achievements as You Learn
 Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.
Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.Create and Customize Your Avatar
 Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Introduction to Order of Operations with Exponents
Understanding the order of operations with exponents is crucial in mathematics. Our introduction video provides a clear and concise explanation of this fundamental concept. The video demonstrates how exponents fit into the broader framework of BEDMAS (Brackets, Exponents, Division, Multiplication, Addition, Subtraction) or PEDMAS (Parentheses, Exponents, Division, Multiplication, Addition, Subtraction). These acronyms serve as essential memory aids, helping students remember the correct sequence for solving complex mathematical expressions. When dealing with exponents, it's vital to recognize their position in this hierarchy. Exponents are evaluated early in the process, immediately after brackets or parentheses. This placement emphasizes their significance in calculations. By mastering the order of operations with exponents, students gain a solid foundation for tackling more advanced mathematical problems. Remember, adhering to BEDMAS/PEDMAS ensures accurate results and prevents common errors in calculations involving solving expressions with exponents and other operations.
Calculate: 5(−2)4
Step 1: Understanding the Order of Operations
To solve the expression 5(−2)4, we need to follow the order of operations, often remembered by the acronym BEDMAS (Brackets, Exponents, Division and Multiplication, Addition and Subtraction). This means we first solve any operations inside brackets, then exponents, followed by division and multiplication (from left to right), and finally addition and subtraction (from left to right).
Step 2: Identify and Solve the Brackets
In the given expression 5(−2)4, we first look at the brackets. The brackets contain the number -2. Since there are no operations to perform inside the brackets, we move on to the next step.
Step 3: Evaluate the Exponent
Next, we handle the exponent. The expression inside the brackets is -2, and it is raised to the power of 4. We need to calculate (−2)4. When raising a negative number to an even power, the result is positive. Therefore, (−2)4=16.
Step 4: Perform the Multiplication
After evaluating the exponent, the expression simplifies to 5×16. Now, we perform the multiplication. Multiplying 5 by 16 gives us 80.
Step 5: Final Answer
After completing all the steps according to the order of operations, we find that the final answer to the expression 5(−2)4 is 80.
Here are some frequently asked questions about order of operations with exponents:
1. What is the rule for operations with exponents?
The rule for operations with exponents is to evaluate exponents before performing other operations like multiplication, division, addition, or subtraction. This is part of the PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) order of operations.
2. How to solve order of operations with exponents?
To solve order of operations with exponents:
- Solve operations within parentheses first
- Evaluate exponents
- Perform multiplication and division from left to right
- Perform addition and subtraction from left to right
3. What are the four operations with exponents?
The four main operations with exponents are:
- Multiplication of exponents with the same base: add the exponents
- Division of exponents with the same base: subtract the exponents
- Power of a power: multiply the exponents
- Power of a product: distribute the exponent to each factor
4. How to use PEMDAS with exponents?
When using PEMDAS with exponents, remember that exponents are evaluated second, right after parentheses. For example, in the expression 2 + 3², you would first calculate 3² = 9, then add 2 to get 11.
5. What to do when the exponent is outside the bracket?
When an exponent is outside a bracket, it applies to everything inside the bracket. For example, (2x)³ means (2x) * (2x) * (2x), which expands to 8x³. Always evaluate the expression inside the bracket first, then apply the exponent to the result.
Understanding the order of operations with exponents is a crucial skill in mathematics, but it's not a standalone concept. To truly master this topic, students must first grasp several fundamental concepts that serve as building blocks. Let's explore how these prerequisite topics intertwine with and support the understanding of order of operations with exponents.
At the core of this subject lies the order of operations (PEMDAS). This fundamental principle governs the sequence in which we perform mathematical operations, ensuring consistency and accuracy in calculations. When dealing with exponents, PEMDAS becomes even more critical. Exponents are typically evaluated before multiplication and division, but after parentheses. This hierarchical structure is essential when solving complex equations involving exponents.
Another crucial prerequisite is understanding the negative exponent rule. This concept is vital when dealing with expressions that include both positive and negative exponents. Knowing how to manipulate negative exponents allows students to simplify expressions and solve equations more efficiently. For instance, when faced with an expression like 2^(-3) * 2^5, understanding negative exponents is key to arriving at the correct solution.
The distributive property also plays a significant role in working with exponents. While it might seem more related to basic algebra, its application extends to exponential expressions. When dealing with expressions like (2x^3 + 4x^2)^2, the distributive property becomes invaluable. It allows students to expand such expressions correctly, maintaining the integrity of the exponents throughout the process.
Moving beyond basic operations, the concept of exponents finds practical applications in real-world scenarios. For example, compound interest calculations heavily rely on exponents. Understanding how to manipulate exponents in financial formulas is crucial for accurately calculating interest over time. This application demonstrates the practical importance of mastering order of operations with exponents.
Furthermore, the study of continuous growth and decay models introduces students to more advanced applications of exponents. These models, often used in science and economics, require a solid grasp of exponential functions and their properties. The ability to correctly apply the order of operations with exponents is fundamental in solving problems related to population growth, radioactive decay, or economic trends.
The interplay between these prerequisite topics creates a robust foundation for understanding the order of operations with exponents. Each concept builds upon the others, forming a comprehensive framework. For instance, when solving a complex problem involving compound interest, students must simultaneously apply their knowledge of PEMDAS, negative exponents, and the distributive property. This interconnectedness highlights why a strong grasp of these prerequisites is essential.
Moreover, these prerequisite topics don't just support the understanding of order of operations with exponents; they also pave the way for more advanced mathematical concepts. As students progress in their mathematical journey, they'll find that these foundational skills are repeatedly called upon. Whether it's in calculus, where exponential functions are extensively studied, or in statistics, where growth models play a crucial role, the principles learned in these prerequisite topics continue to be relevant.
It's also worth noting that mastering these prerequisites enhances problem-solving skills beyond just mathematical calculations. The logical thinking required to navigate the order of operations, for example, translates into improved analytical skills in various fields. Similarly, understanding exponential growth models can provide insights into diverse areas such as epidemiology, environmental science, and technology adoption rates.
In conclusion, the journey to mastering the order of operations with exponents is a multifaceted one, requiring a solid understanding of several interconnected concepts. From the fundamental rules of PEMDAS to the practical applications in finance and growth models, each prerequisite topic contributes uniquely to a comprehensive understanding of exponents. By recognizing the importance of these building blocks and how they relate to



