Area of triangles:$\frac{1}{2} ab$ sinC - Trigonometric Ratios and Angle Measures

Notes:

In this lesson, we will learn:

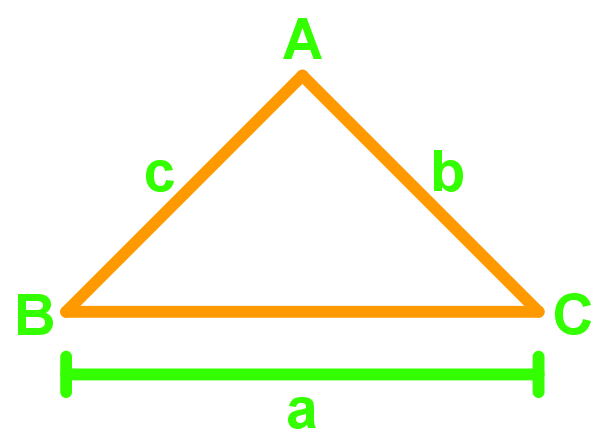
• Proof: Area of Triangle =$\frac{1}{2}ab \sin C$
• Finding the Area of a Triangle Given 2 Sides and the Angle in Between
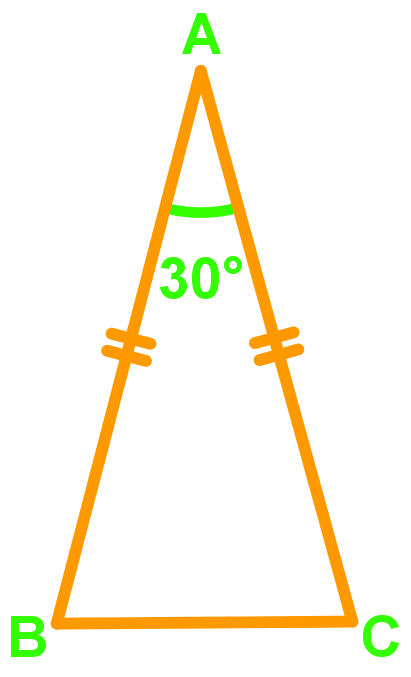
• Area of Isosceles Triangles
• Area of Equilateral Triangles
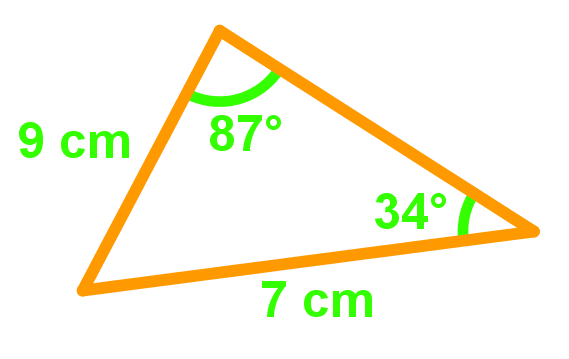
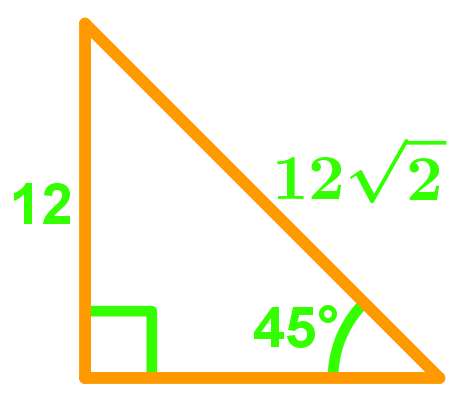
• Determining the Areas of Different Triangles

Notes:

• The base and height of a triangle must be perpendicular to each other.
• The traditional formula for the area of a triangle = $\frac{1}{2} \times base \times height$
• An isosceles triangle has two sides of equal length.
• An equilateral triangle has three sides of equal length and all the inner angles equal to 60°.
• Angles inside a triangle add up to 180°.
• Pythagorean Theorem: $a^{2}+b^{2}=c^{2}$