Sign In
Sign Up
flag-us

Rotational Kinetic Energy & Angular Momentum Explained
Dive into the fascinating world of rotational motion! Understand the principles behind spinning objects and orbiting planets. Our clear explanations and visual aids make complex concepts easy to grasp.

Now Playing:Rotational kinetic energy and angular momentum – Example 0a
Intros
  1. Rotational kinetic energy
  2. Angular momentum
Examples
  1. A bowling ball with a mass on 5.0kg and radius of 12.0cm is rolling down an inclined surface with a negligible friction without slipping. If it starts from rest at a vertical distance of 1.5m, what will be the speed of the ball when it reaches the bottom of the inclined surface?
    Rotational Vs. translational kinematics
    Jump to:NotesConceptFAQsPrerequisites
    Notes

    In this lesson, we will learn:

    • How to calculate rotational kinetic energy?
    • Definition of angular momentum
    • Newton’s 2nd law for rotation
    • The law of conservation of angular momentum

    Notes:


    Rotational Kinetic Energy

    • Objects rotating about an axis possess “Rotational Kinetic Energy”
    • Objects moving along a straight line possess “Translational Kinetic Energy”.
    • Translational kinetic energy is calculated using, 12mv2\frac{1}{2}mv^{2} , we can convert this formula to rotational kinetic energy question using the rotational motion analogues:

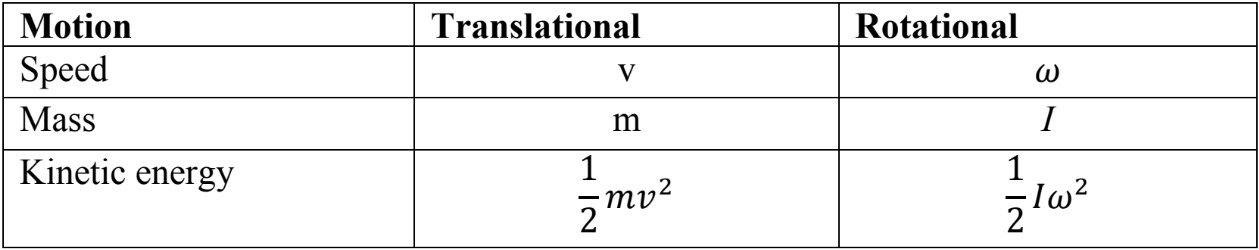
      • Rotational Kinetic Energy and Angular Momentum


      If we consider a rotating ball, every point on the ball is rotating with some speed.
      The ball is made up many tiny particles, each of mass “mm”. let’s take “rr” to be the distance of any one particle from the axis of rotation (O);

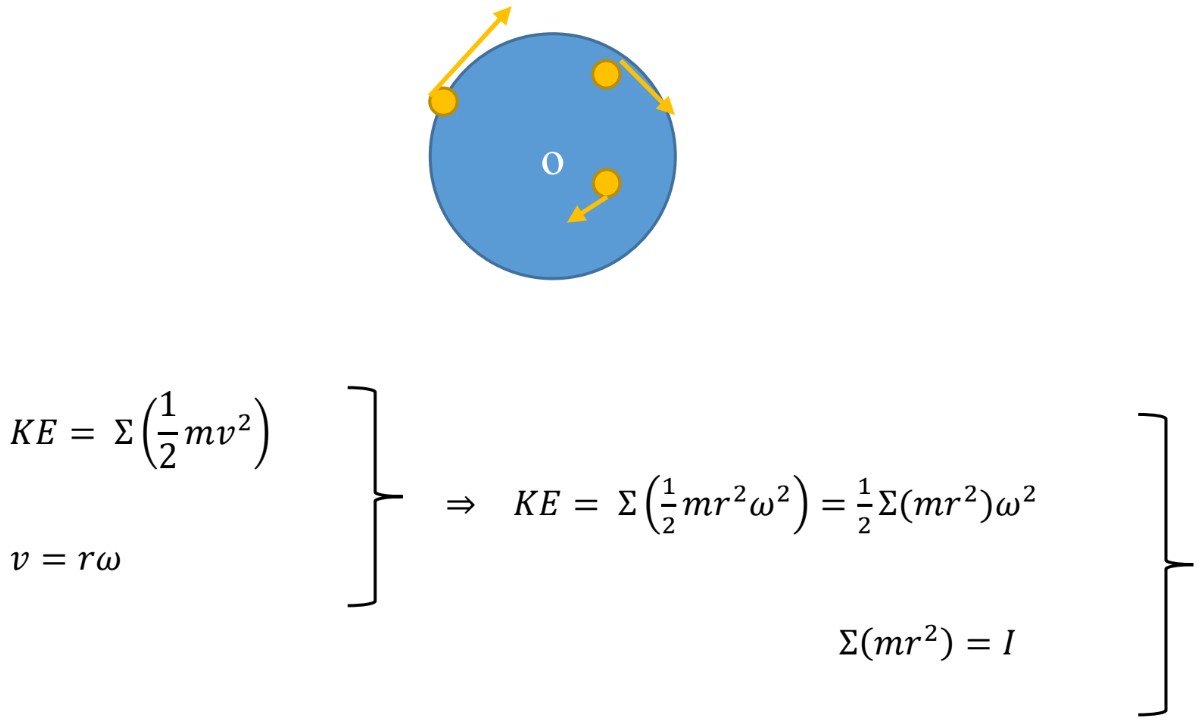
      Rotational Kinetic Energy and Angular Momentum


      ⇒ \Rightarrow \, rotational KE=12I ω2KE = \frac{1}{2}I\, \omega^{2}

    • The center of mass of a rotating object might undergo translational motion (a sphere rolling down an incline), in this case we have to consider both rotational and translational kinetic energy.


      • KE=12Mv2 + 12ICM  ω2KE = \frac{1}{2}M v^{2} \, + \, \frac{1}{2}I_{CM \;} \omega^{2}


      MM : total mass of the object
      ICMI_{CM} : moment of inertia about the axis through center of mass 
      vv : translational speed 
      ω\omega : angular speed 


      Angular Momentum
      In the previous section, we used the rotational analogue to translate the translational kinetic energy to rotational kinetic energy.
      In like manner, the linear momentum can be changed to angular momentum, using the rotational analogues;

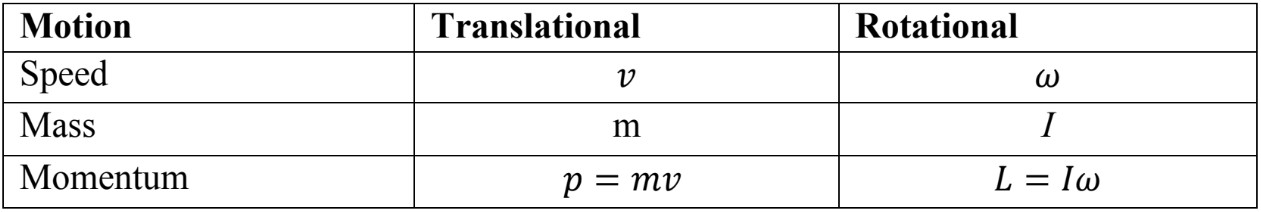
      Rotational Kinetic Energy and Angular Momentum

      LL: is the angular momentum with a standard unit of kg.m2/s


      Newton’s 2nd Law for Rotation


      The Newton’s 2nd law also can be written in terms of rotational analogues;

      ∑F= ma =\sum F = \, ma \, = mΔvΔt\large \frac{m \Delta v}{\Delta t} == ΔpΔt\large \frac{\Delta p}{\Delta t} \quad , in translational motion “Force” causes linear acceleration

      \qquad \quad Similarly for rotational motion , ∑=\sum = ΔLΔt \large \frac{\Delta L }{\Delta t}

      ∑τ= \sum \tau = \, ΔLΔt\large \frac{\Delta L}{\Delta t} = = IΔωΔt\large \frac{I \Delta \omega}{\Delta t}  =I∝\, = I \propto \quad , in rotational motion “Torque” causes rotational acceleration

      ∑F=ma\sum F = ma \quad Newton’s 2nd Law in Translational Motion
      ∑τ=I∝\sum \tau = I \propto \quad Newton’s 2nd Law in Rotational Motion


      The law of Conservation of Angular Momentum

      The law of conservation of angular moment states that;

      “The total angular momentum of a rotating object remains constant if the net torque acting on it is zero.”

      ∑τ=0  ⇒  Li=Lf  ⇒  Iiωi=Ifωf\sum \tau = 0 \; \Rightarrow \; L_{i} = L_{f} \; \Rightarrow \; I_{i \omega i} = I_{f \omega f}

      Example: A skater doing a spin on ice, illustrating conservation of angular momentum.

      Rotational Kinetic Energy and Angular Momentum


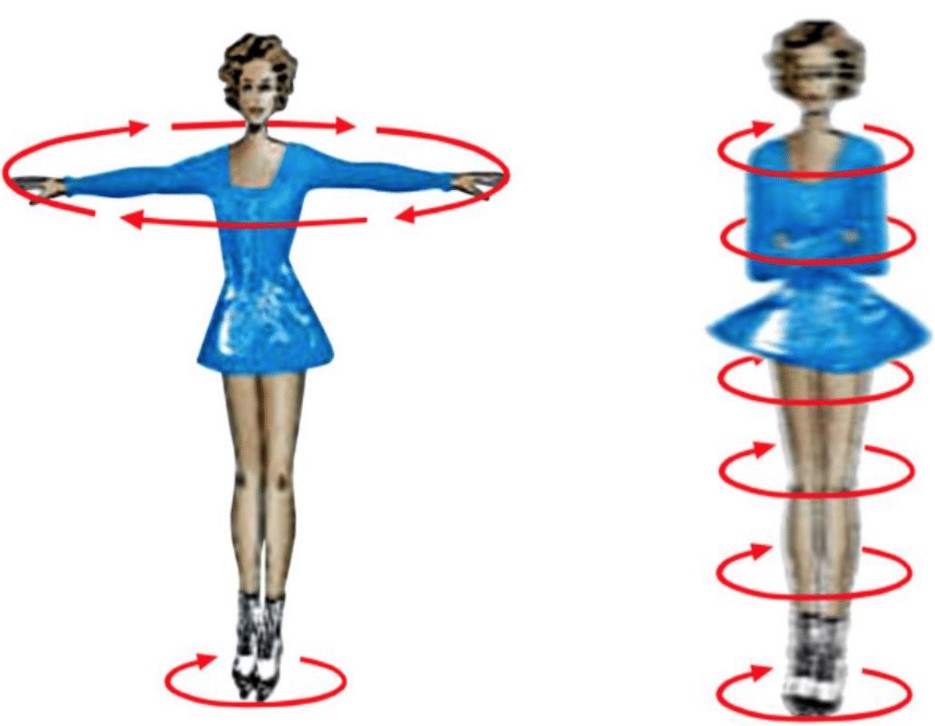
      Open arms: Li=Iωi=mri2ωi \qquad L_{i} = I \omega_{i} = mr^{2}_{i} \omega_{i}
      Closed arms:   Lf=Iωf=mrf2ωf\qquad L_{f} = I \omega_{f} = mr^{2}_{f} \omega_{f}

      When the arms of the skater are tucked in, the mass is not changing, but the radius of rotation decreases; rfr_{f} < rir_{i} , therefore;  mrf2mr^{2}_{f} < mri2mr^{2}_{i}

      According to the law of conservation of angular momentum; Li=LfL_{i} = L_{f}

      Li=LfL_{i} = L_{f}

      ⇒ωf\qquad \Rightarrow \quad \omega_{f} > ωi  \omega_{i} \; “the result would be higher angular velocity”

      mrf2 mr^{2}_{f} < mri2 mr^{2}_{i}
    Concept

    Introduction to Rotational Kinetic Energy and Angular Momentum

    Welcome to our exploration of rotational kinetic energy and angular momentum! These fascinating concepts are crucial in understanding the motion of rotating objects. Rotational kinetic energy is the energy possessed by an object due to its rotational motion, while angular momentum describes the object's tendency to continue rotating. Our introduction video serves as an excellent starting point, providing clear visuals and explanations to help you grasp these ideas. As your math tutor, I'm excited to guide you through these concepts, which have wide-ranging applications in physics and engineering. From spinning ice skaters to orbiting planets, rotational kinetic energy and angular momentum play key roles in explaining the world around us. By the end of this lesson, you'll have a solid foundation in these principles, enabling you to tackle more complex problems with confidence. Let's dive in and unravel the mysteries of rotational motion together!

    FAQs

    1. What is rotational kinetic energy?

      Rotational kinetic energy is the energy possessed by an object due to its rotational motion. It is defined by the equation KErotational = ½ I ω², where I is the moment of inertia and ω is the angular velocity. This energy form is distinct from translational kinetic energy and is crucial in understanding the behavior of rotating objects.

    2. How do you find angular kinetic energy?

      To find angular kinetic energy, use the formula KErotational = ½ I ω². First, determine the object's moment of inertia (I) based on its shape and mass distribution. Then, measure or calculate its angular velocity (ω). Multiply half of the moment of inertia by the square of the angular velocity to obtain the rotational kinetic energy.

    3. What is the angular equation for kinetic energy?

      The angular equation for kinetic energy is KErotational = ½ I ω². This equation is analogous to the linear kinetic energy equation (KE = ½ mv²), with moment of inertia (I) replacing mass (m) and angular velocity (ω) replacing linear velocity (v).

    4. What is the kinetic energy of a rotating object?

      The kinetic energy of a rotating object is the sum of its translational and rotational kinetic energies. If the object is purely rotating (not moving linearly), its kinetic energy is entirely rotational, given by KErotational = ½ I ω². For objects with both rotational and translational motion, the total kinetic energy is KEtotal = ½ mv² + ½ I ω².

    5. How does angular momentum relate to rotational kinetic energy?

      Angular momentum (L) and rotational kinetic energy are closely related but distinct concepts. Angular momentum is given by L = I ω, while rotational kinetic energy is KErotational = ½ I ω². Both involve moment of inertia (I) and angular velocity (ω), but angular momentum is a vector quantity conserved in closed systems, whereas rotational kinetic energy is a scalar quantity that can be transferred or converted to other forms of energy.

    Prerequisites

    Understanding rotational kinetic energy and angular momentum is crucial in physics, but to truly grasp these concepts, it's essential to have a solid foundation in prerequisite topics. Two key areas that form the bedrock of this understanding are Newton's Second Law and the principle of conservation of angular momentum.

    Newton's Second Law is fundamental to understanding rotational motion. This law, which states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass, forms the basis for analyzing rotational dynamics. When we extend this principle to rotating objects, we derive equations for rotational motion that parallel those for linear motion. The concept of force in linear motion translates to torque in rotational motion, while mass becomes moment of inertia. This connection allows us to calculate rotational kinetic energy, which is analogous to linear kinetic energy but involves angular velocity and moment of inertia instead of linear velocity and mass.

    The principle of conservation of angular momentum is another critical prerequisite for understanding rotational motion. While the link provided focuses on linear momentum, the concept extends beautifully to rotational systems. Just as linear momentum is conserved in the absence of external forces, angular momentum is conserved in the absence of external torques. This principle is crucial for analyzing spinning objects and explains phenomena like the speeding up of figure skaters when they pull in their arms. Understanding this conservation law is essential for solving problems involving rotating bodies and for grasping the behavior of systems like gyroscopes and planets.

    By mastering these prerequisite topics, students can build a strong foundation for understanding more complex concepts in rotational dynamics. Newton's Second Law provides the framework for analyzing forces and motion, which directly applies to rotational systems. The conservation of angular momentum principle, an extension of linear momentum conservation, offers powerful insights into the behavior of rotating objects. Together, these concepts enable students to tackle problems involving rotational kinetic energy and angular momentum with confidence and clarity.

    In conclusion, a thorough understanding of these prerequisite topics is not just helpful but essential for mastering rotational kinetic energy and angular momentum. By building on these fundamental principles, students can develop a deeper, more intuitive grasp of rotational dynamics, preparing them for more advanced topics in physics and engineering.