In this lesson, we will learn:
- How to solve two dimensional vector problems using the law of sines and the law of cosines
Notes:
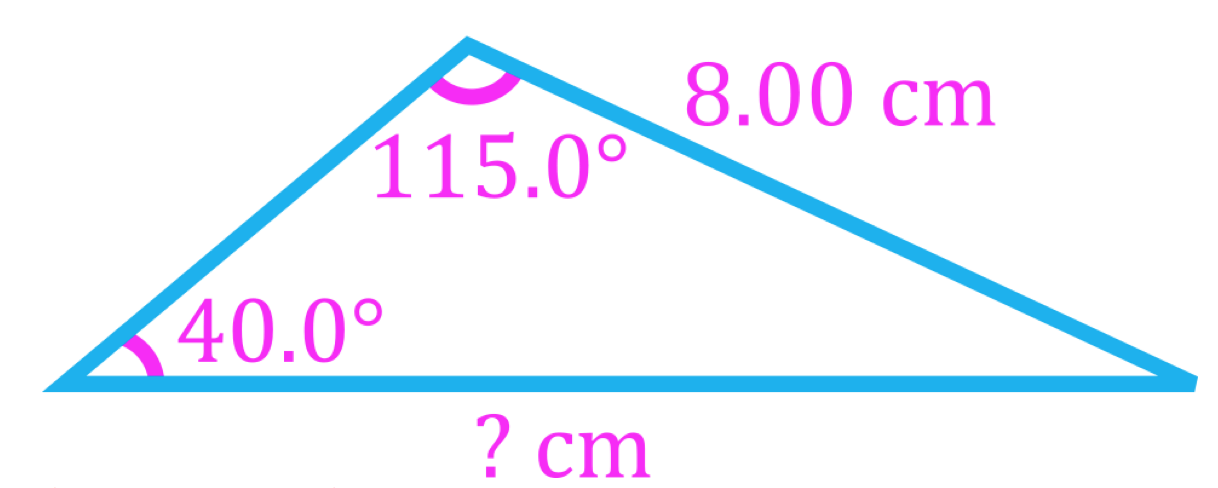
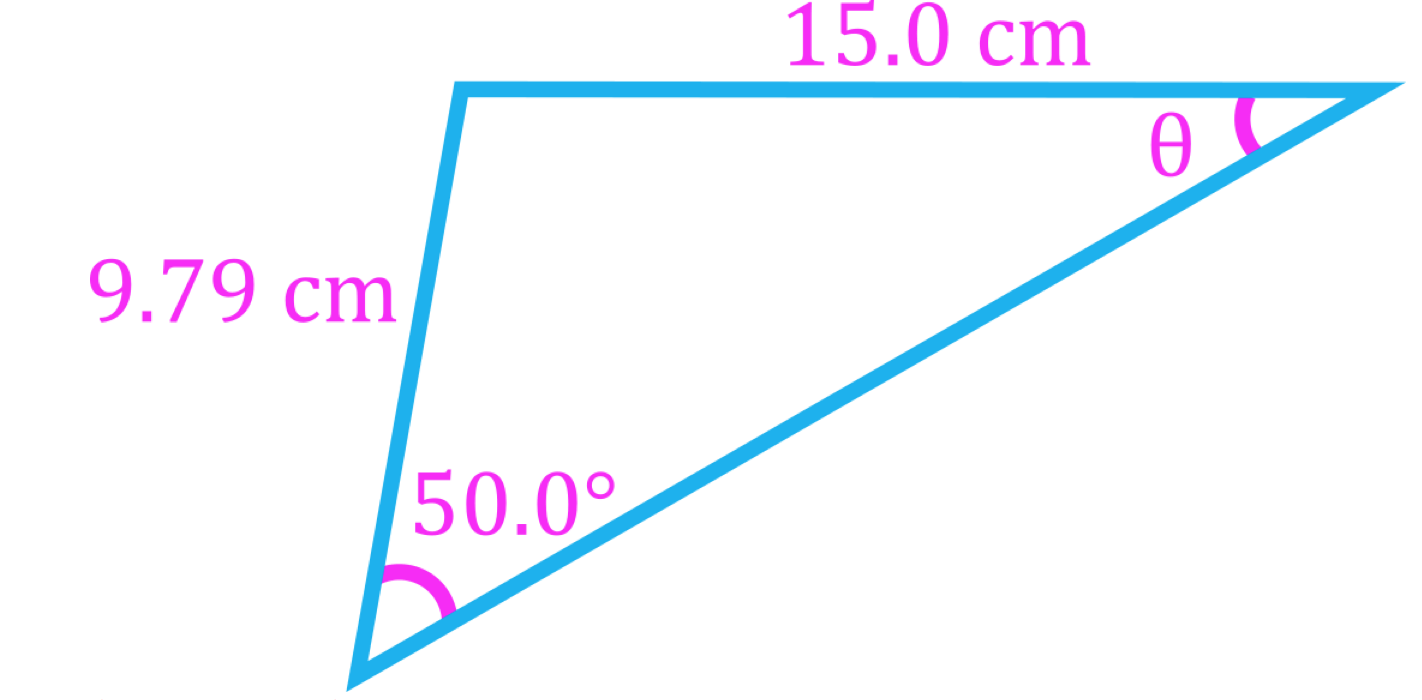
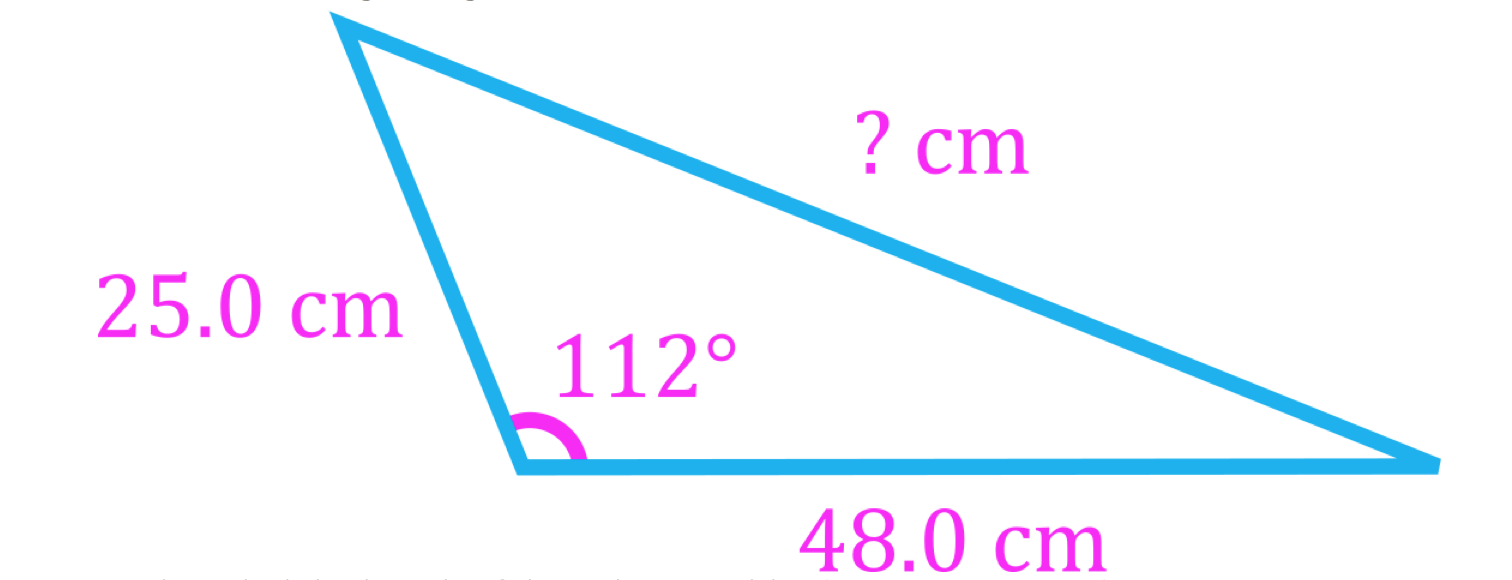
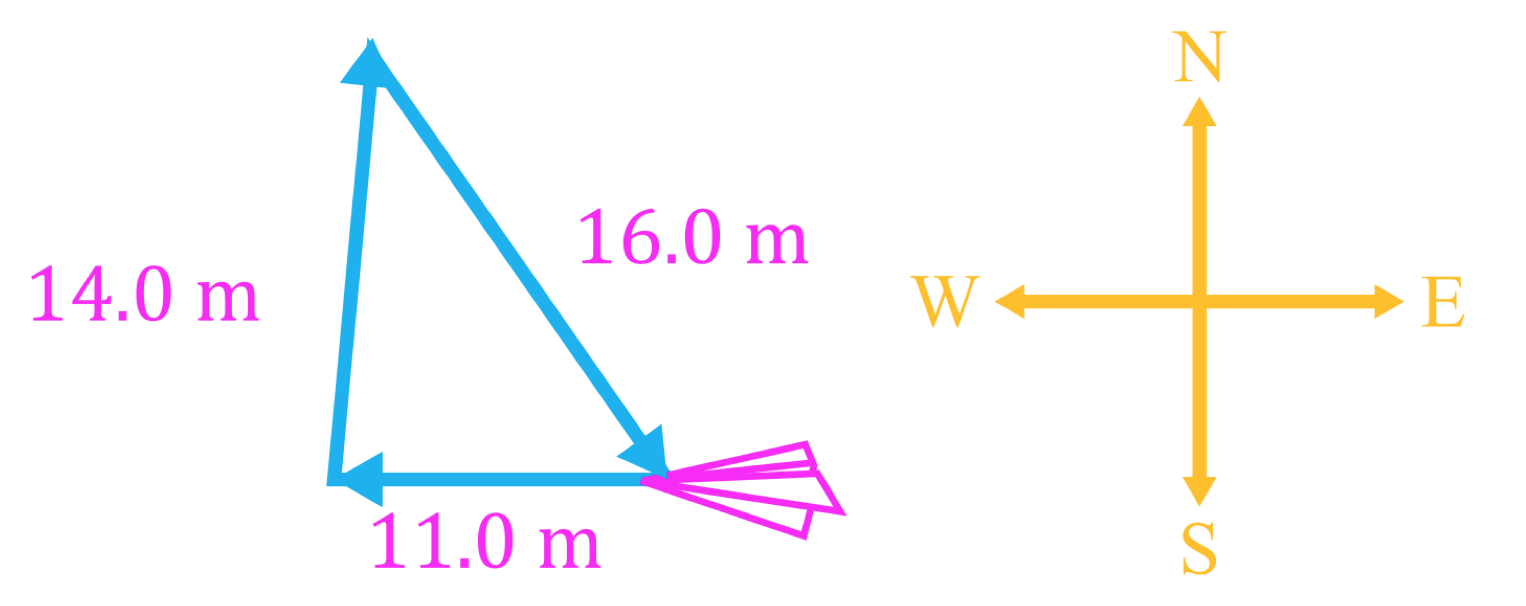
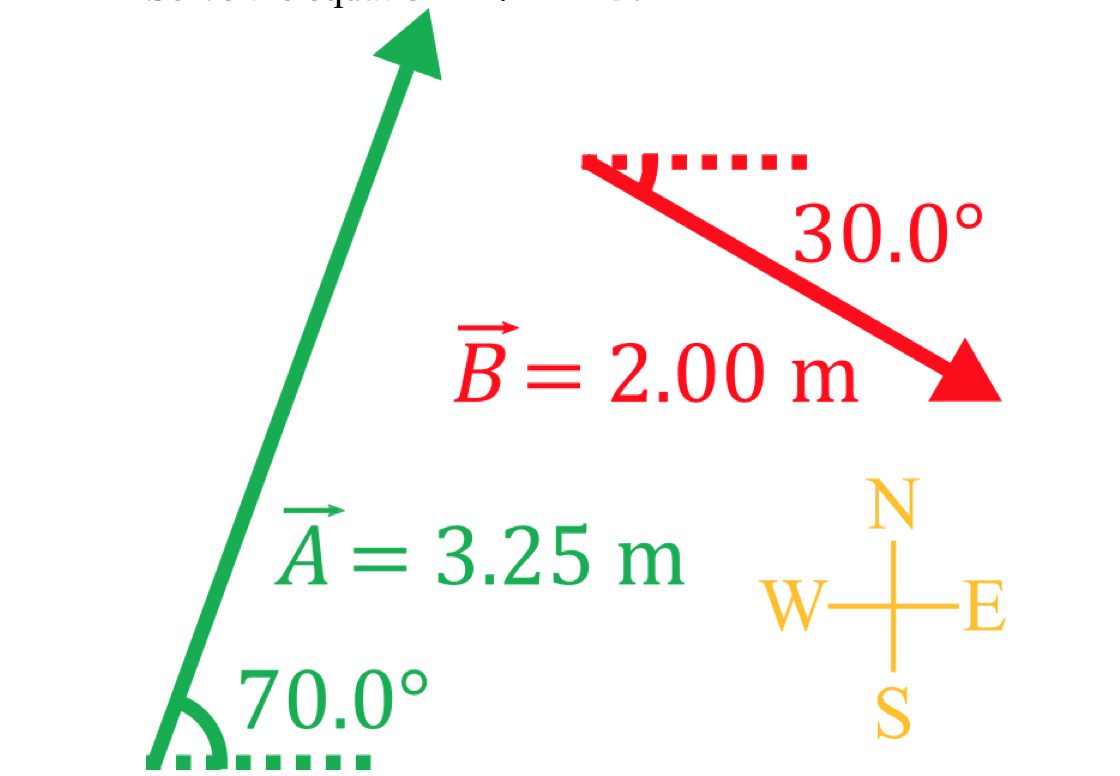
- Often, vector equations in physics problems result in vector triangles which can be solved using trigonometry
- At least three pieces of information are needed to solve a triangle, which can be three side lengths (SSS), two side lengths and one angle (SSA, SAS), or one side length and two angles (SAA, ASA).
- Knowing three angles (AAA) does not let you solve a triangle since you will not be able to solve for the side lengths. There is no way to know the size of the triangle without more information.
- You can always solve a triangle that you know four or more pieces of information about.
- Vector triangles that do not contain right angles can be solved either by breaking vectors into their components or using the law of sines and the law of cosines, which are trigonometric laws that apply to all triangles
Law of Sines
a,b,c: length of sides a,b,c
A,B,C: angles opposite sides a, b, c
Law of Cosines