How to Solve System of Equations:
Before we get into solving systems of linear equations via the elimination method, let's first consider and understand what it means to "solve" a system of equations. When we say "solve", with regards to linear, quadratic, exponential, or any other type of equation, what we really mean is that we are trying to find values of 'x' – the dependent variable – that satisfy 'y' – the independent variable.
Take for example the following, simple, equation: y = 2x = 2
In this example equation, we know that y is equal to 2x and is also equal to 2. With that knowledge, since y is equal to both 2x and 2, we can say that 2x = 2. Then, the next natural step is to solve this equation using algebra, giving us the "solution" that x = 1.
In the case of systems of equations, the process isn't that different. In solving systems of equations, what we are trying to do is trying to find values of x and y that makes two distinct equations equal to each other – effectively "solving" both equations. Further information on system of equations can be founded in another lesson. In a system of equations, there are several outcomes which can occur with regards to the number of solutions. We have the specific lessons on how to determine the number of solutions to linear equations and system of linear-quadratic equations. We also have graphing systems of equations and inequalities covered!
To do so, there are two main methods: solving systems by substitution, and solving systems by elimination. In this article, we will focus on substitution, which is arguably slightly more simple than the other method, elimination. For elimination, please check out the video and articles that focus on that method in particular. To make sure you're ready for elimination, it is important to master adding and subtracting polynomials and adding and subtracting rational expressions.
Now that we've covered the basics, let's solve systems using elimination!
Solving Systems of Equations By Elimination:
Before we get into using the method of elimination, make sure you're comfortable with your algebra by reviewing the lesson on solving linear equations with variables on both sides.
The procedure behind the process of solving by elimination isn't overly difficult. Given systems of linear equations, what we are trying to do is "modify" one or both of the equations so that we can add/subtract them in order to "eliminate" a variable. It's hard to outline the procedure of elimination without a concrete example, so let's take a look at the elimination method steps by doing an example.
Example 1:
Take the following simultaneous equations and solve by elimination.
Step 1: Identify modifications and operations needed to eliminate a variable
In this example, we are lucky enough to have the same coefficient on y for both equations. The first equation has 4y and the second equation has -4y. In this case, it is very easy to add these equations together to "eliminate" y. That is because when we add these two equations together, 4y + (-4y) will equal 0, leaving x as the only variable left, which we can easily solve for.
Thus, in order to eliminate the common variable 4y in both equations, we add the equations together:
Step 2: Solve for x
Now that we have the equation 4x = 4 from our elimination step, we can easily solve for x.
Step 3: Solve for y
Now that we have x = 1, all we have to do is put x = 1 into either of the equations to solve for y.
Step 4: Write out final answer as coordinate
Therefore, our final answer is: (1, 2)
The image below summarizes everything we have just done:
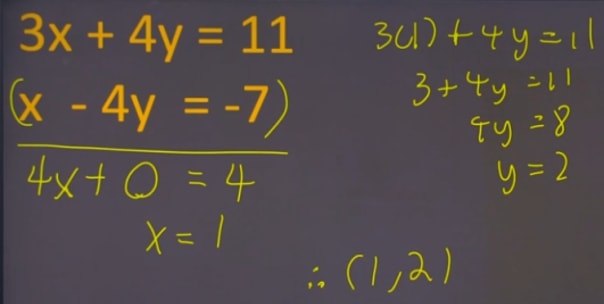
Now that we know how to use the elimination method to solve systems of linear equations, let's do another, more difficult, example.
Example 2:
Solve the following system of equations using the elimination method.
Step 1: Identify modifications and operations needed to eliminate a variable
In this example, we aren't lucky enough to have the same coefficient on y for both equations. So, to eliminate y, we need to multiply both equations by the common ratio as follows:
Which gives us:
Now that we have the common variable 10y in both equations, we can subtract the equations to eliminate y:
Step 2: Solve for x
Now that we have the equation 12x = 13 from our elimination step, we can easily solve for x.
Step 3: Solve for y
Now that we have , all we have to do is put into either of the equations to solve for y.
Step 4: Write out final answer as coordinate
Therefore, our final answer is: ()
The image below summarizes everything we have just done:
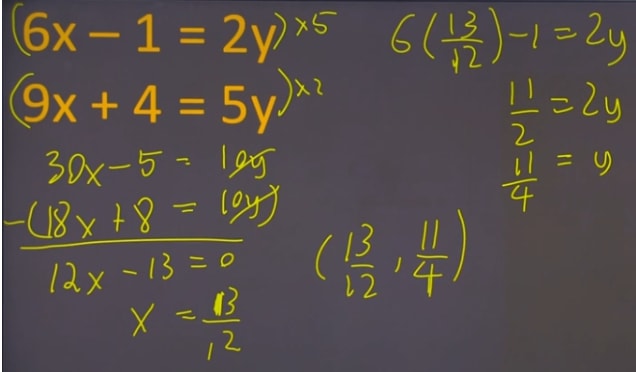
And that's all there is to it! Now, make sure you do lots of practice problems to get more comfortable using this method. As well, check out this great link, which will allow you to easily check your work.






