What is the polynomial remainder theorem?

0/4

Examples

Lessons

Understanding the remainder Theorem
Prove the Remainder Theorem
Finding the Remainder Using Synthetic Division and the Remainder Theorem
Find the remainder when $\left( {4{x^3} - 7x + 10} \right)$ $(4 x^{3} - 7 x + 10)$ is divided by $\left( {2x - 5} \right)$ $(2 x - 5)$
1. Using synthetic division
2. Using the remainder theorem
When $\left( {8{x^3} + a{x^2} + bx - 1} \right)$ $(8 x^{3} + a x^{2} + b x - 1)$ is divided by:
i) $\left( {2x - 5} \right)$ $(2 x - 5)$ , the remainder is $54$ $54$
ii) $\left( {x + 1} \right)$ $(x + 1)$ , the remainder is $- 30$ $- 30$
Find the values of $a$ $a$ and $b$ $b$ .

Topic Notes

You may want to refresh your memory on polynomial long division and synthetic division to better understand this lesson. The remainder theorem simply states that if a polynomial f(x) is divided by a linear expression x-r, the value of f(r) is equal to the remainder.

\cdot

When a polynomial,

P(x)

, is divided by

(x-a)

: Remainder

=P(a)

\cdot

When a polynomial,

P(x)

, is divided by

(ax-b)

: Remainder

=P(\frac{b}{a})

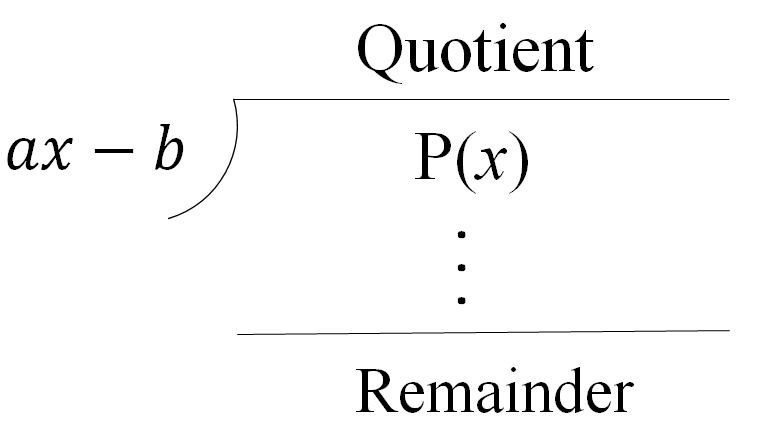
Remainder theorem

All in One Place

Learn with Ease

Instant and Unlimited Help

Make math click 🤔 and get better grades! 💯Join for Free

Examples

Lessons

Free to Join!

Easily See Your Progress

Make Use of Our Learning Aids

Last Viewed

Practice Accuracy

Suggested Tasks

Earn Achievements as You Learn

Create and Customize Your Avatar

Topic Notes

Basic Concepts

Remainder theorem

All in One Place

Learn with Ease

Instant and Unlimited Help

Make math click 🤔 and get better grades! 💯Join for Free

Lessons

Easily See Your Progress

Make Use of Our Learning Aids

Last Viewed

Practice Accuracy

Suggested Tasks

Earn Achievements as You Learn

Create and Customize Your Avatar

Become a member to get more!