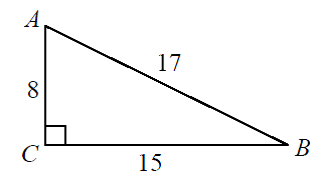1. Home
  2. >ACCUPLACER Test Prep
  3. >Right Triangle Trigonometry
Help

Use sine ratio to calculate angles and sides (Sin = o / h)

0/1
?
Intros
Lessons
  1. What are sine, cosine and tangent?
0/7
?
Examples
Lessons
  1. Determine each sine ratio using a calculator
    1. sin⁡60∘\sin 60^\circ
    2. sin⁡−60∘\sin-60^\circ
  2. Determine the angle to the nearest degree using a calculator
    1. sin⁡θ=0.57\sin \theta =0.57
    2. sin⁡θ=−0.65\sin \theta = -0.65
  3. Determine the angles and sides using Sine ratio
    1. Find angle A and B:

      Use sine ratio to find angles in a triangle
    2. Find the value of "x" using sine

      Use sine ratio, opposite side length to calculate hypotenuse
    3. Find the value of "x" using sine

      Use sine ratio, hypotenuse and angle to calculate opposite side length
  4. Draw and label a right triangle to illustrate sine ratio, then calculate the angle.
    1. sin⁡θ=513\sin \theta = {5 \over 13}
Topic Notes
?
One of the trigonometric ratios is sine ratio. It is the opposite side over the hypotenuse of right triangles. In other words, we can find the angles and sides of right-angled triangles by using the sine ratios.

Introduction: Understanding Sine Ratio in Trigonometry

Sine ratio is a fundamental concept in trigonometry, playing a crucial role in solving problems involving right-angled triangles. The introduction video provides an essential foundation for grasping this important trigonometric function. Sine ratio, along with cosine and tangent, forms the core of trigonometric ratios used to calculate sides and angles in right-angled triangles. This ratio is defined as the length of the opposite side divided by the length of the hypotenuse in a right-angled triangle. Understanding sine ratio is vital for various applications in mathematics, physics, engineering, and real-world scenarios. It allows us to determine unknown angles or side lengths in triangles, making it an indispensable tool in fields such as navigation, architecture, and astronomy. By mastering the concept of sine ratio, students can unlock a wealth of problem-solving techniques and gain a deeper appreciation for the practical applications of trigonometry in everyday life and advanced scientific disciplines.

What is Sine Ratio?

The sine ratio, often written as sin = opposite / hypotenuse or sin = o/h, is a fundamental concept in trigonometry. This ratio relates the lengths of sides in a right-angled triangle to one of its angles. To understand the sine ratio formula, let's break it down into its components.

In a right-angled triangle:

  • The opposite side is the one directly across from the angle we're focusing on.
  • The hypotenuse is always the longest side of the triangle, opposite the right angle.

The sine ratio formula is calculated by dividing the length of the opposite side by the length of the hypotenuse. This gives us the sine ratio formula: sin = opposite / hypotenuse.

Diagram of sine ratio in a right-angled triangle

Understanding the sine ratio is crucial in trigonometry for several reasons:

  1. It allows us to calculate sides or angles in right-angled triangles.
  2. It's used in more advanced trigonometric concepts and calculations.
  3. It has numerous real-world applications.

The importance of the sine ratio extends far beyond mathematics classrooms. Here are some real-world applications:

  • Architecture and Construction: Calculating heights of buildings or angles of roofs.
  • Navigation: Determining distances and directions in maritime and aviation.
  • Physics: Analyzing forces and motion in various scenarios.
  • Engineering: Designing structures and machines with precise angles.
  • Astronomy: Measuring distances between celestial bodies.

For example, an architect might use the sine ratio to calculate the height of a building based on its distance from an observer and the angle of elevation. Similarly, a navigator might use it to determine the distance to a landmark based on its angle of elevation and the height of the observer.

The sine ratio definition can be extended to non-right-angled triangles through the law of sines, further expanding its applications. This versatility makes the sine ratio a powerful tool in various fields of study and practical applications.

To use the sine ratio effectively:

  1. Identify the right-angled triangle in your problem.
  2. Determine which angle you're working with.
  3. Identify the opposite side and hypotenuse relative to this angle.
  4. Apply the formula: sin = opposite / hypotenuse.

Remember, the sine ratio is just one of the trigonometric ratios, alongside cosine and tangent. Together, these ratios form the foundation of trigonometry, enabling us to solve complex problems involving angles and distances in both theoretical and practical contexts.

In conclusion, the sine ratio formula (sin = o/h) is a fundamental concept in trigonometry that relates the opposite side to the hypotenuse in a right-angled triangle. Its importance lies in its wide-ranging applications across various fields, from construction and navigation to physics and astronomy. By understanding and applying this simple yet powerful ratio, we can unlock solutions to numerous real-world problems involving angles and distances.

Understanding Right-Angled Triangles and Trigonometric Ratios

Right-angled triangles are fundamental shapes in geometry and trigonometry, playing a crucial role in various mathematical and real-world applications. These triangles are unique because they contain one angle that measures exactly 90 degrees, known as the right angle. This special characteristic forms the basis for understanding trigonometric ratios and their relationships within the triangle.

The right angle, always measuring 90 degrees, is the cornerstone of a right-angled triangle. It significantly influences the other two angles and the lengths of the sides. According to the angle sum property of triangles, the sum of all angles in a triangle must equal 180 degrees. In a right-angled triangle, with one angle already fixed at 90 degrees, the remaining two angles must sum to 90 degrees. This property ensures that these triangles always maintain their distinctive shape and proportions.

Trigonometric ratios are mathematical relationships between the sides of a right-angled triangle. These ratios are essential tools in trigonometry and have wide-ranging applications in fields such as engineering, physics, and navigation. The three main trigonometric ratios are sine, cosine, and tangent. While all three are important, we'll focus primarily on the sine ratio in this discussion.

To help remember these ratios, many students use the mnemonic device "SOH CAH TOA." This acronym stands for:

  • SOH: Sine = Opposite / Hypotenuse
  • CAH: Cosine = Adjacent / Hypotenuse
  • TOA: Tangent = Opposite / Adjacent

Let's delve deeper into the sine ratio. In a right-angled triangle, the sine of an angle is defined as the length of the opposite side divided by the length of the hypotenuse. The hypotenuse is always the longest side of the triangle and is opposite the right angle. The opposite side is the one across from the angle in question.

For example, if we have a right-angled triangle with angles of 30°, 60°, and 90°, and we want to find the sine of 30°, we would divide the length of the side opposite to the 30° angle by the length of the hypotenuse. This ratio remains constant for all right-angled triangles with a 30° angle, regardless of their size.

The sine ratio is particularly useful in many practical applications. For instance, it can be used to calculate the height of a tall building or tree by measuring the angle of elevation and the distance from the base. In physics, sine waves are fundamental in understanding oscillations and waves. In engineering, the sine ratio is crucial for analyzing forces and designing structures.

While we've focused on the sine ratio, it's important to briefly mention the other two main trigonometric ratios for context. The cosine ratio relates the adjacent side to the hypotenuse, while the tangent ratio compares the opposite side to the adjacent side. Each of these ratios has its own unique applications and properties, but they all stem from the fundamental structure of the right-angled triangle.

Understanding right-angled triangles and trigonometric ratios opens up a world of mathematical possibilities. These concepts form the foundation for more advanced topics in trigonometry and calculus. They allow us to solve complex problems involving angles and distances, making them indispensable tools in many fields of study and professional practice.

In conclusion, right-angled triangles, with their distinctive 90-degree angle, provide the perfect framework for understanding trigonometric ratios. The sine ratio, along with cosine and tangent, offers powerful tools for analyzing and solving problems involving triangles. By mastering these concepts and remembering the SOH CAH TOA mnemonic, students and professionals alike can tackle a wide range of mathematical challenges with confidence.

How to Calculate Sine Ratio

Understanding how to calculate sine ratio is essential for trigonometry and many real-world applications. This comprehensive guide will walk you through the process step-by-step, provide examples, and explain how to use a scientific calculator for sine calculations.

Step-by-Step Guide to Calculate Sine

  1. Identify the right triangle and the angle you're working with.
  2. Locate the side opposite to the angle and the hypotenuse.
  3. Use the formula: sine = opposite / hypotenuse.
  4. Divide the length of the opposite side by the length of the hypotenuse.
  5. The result is the sine of the angle.

Examples of Sine Calculation

Let's look at some examples with varying difficulty levels:

Basic Example:

In a right triangle with an angle of 30° and a hypotenuse of 10 units, find the sine of 30°.

Solution: The opposite side is 5 units. Therefore, sine(30°) = 5/10 = 0.5

Intermediate Example:

Calculate the sine of 45° in a right isosceles triangle with legs of length 1.

Solution: The hypotenuse is 2. Thus, sine(45°) = 1/2 0.7071

Advanced Example:

Find the sine of 60° without a calculator.

Solution: In a 30-60-90 triangle, if the shortest side is 1, the hypotenuse is 2. The side opposite to 60° is 3. Therefore, sine(60°) = 3/2 0.8660

Using a Scientific Calculator for Sine

  1. Ensure your calculator is in the correct mode (degree or radian).
  2. Enter the angle value.
  3. Press the 'sin' button.
  4. The display will show the sine value.

Importance of Angle Measurement: Degrees vs. Radians

When calculating sine, it's crucial to know whether you're working with degrees or radians. Most calculators can switch between these modes.

Degrees:

  • More commonly used in everyday applications.
  • A full circle is 360°.
  • Easier to visualize for most people.

Radians:

  • Preferred in advanced mathematics and physics.
  • A full circle is 2π radians.
  • More natural for calculus and other higher-level math.

To convert between degrees and radians:

  • Degrees to radians: multiply by π/180
  • Radians to degrees: multiply by 180/π

Practice Problems

Try these practice sine problems to test your understanding:

  1. Calculate the sine of 60° without a calculator.
  2. Find sin(π/4) using a calculator in radian mode.
  3. In a right triangle with hypotenuse 13 and opposite side 5, what is the sine of the angle?
  4. Convert 45° to radians and calculate its sine.
  5. What is the sine of 180°? Explain your answer.

Applications of Sine Ratio

The sine ratio, a fundamental trigonometric function, finds extensive applications in various real-world scenarios, demonstrating its practical importance across multiple fields. In engineering, architecture, navigation, and physics, the sine ratio serves as a powerful tool for solving complex problems involving height, distance, and angles.

In engineering, the sine ratio is crucial for designing and analyzing structures. For instance, civil engineers use it to calculate the forces acting on beams and trusses in bridges. By applying the sine ratio to the angles between structural components, they can determine the distribution of loads and ensure the stability of the entire structure. Similarly, mechanical engineers employ the sine ratio in the design of gears and cams, where precise angular measurements are essential for smooth operation.

Architecture heavily relies on the sine ratio for both aesthetic and functional purposes. When designing sloped roofs, architects use the sine ratio to calculate the exact pitch and determine the length of rafters needed. This ensures proper water drainage and structural integrity. In the creation of spiral staircases, the sine ratio helps in determining the rise and run of each step, ensuring a comfortable and safe ascent.

Navigation, both maritime and aerial, extensively utilizes the sine ratio. In marine navigation, sailors use it to calculate distances between two points on a map, taking into account the curvature of the Earth. The sine ratio is essential in determining the ship's position using celestial navigation techniques. In aviation, pilots use the sine ratio to calculate the glide slope during landing approaches, ensuring a safe descent angle to the runway.

Physics applications of the sine ratio are numerous and diverse. In optics, it's used to describe the behavior of light as it passes through different mediums, known as Snell's law. The sine ratio helps explain refraction and is crucial in designing lenses and optical instruments. In acoustics, the sine ratio is used to analyze sound waves and their propagation, aiding in the design of concert halls and sound systems.

A simple, relatable example of the sine ratio in action is in determining the height of tall structures. Imagine you want to measure the height of a tall building without climbing to the top. By standing a known distance away and measuring the angle to the top of the building using a clinometer, you can use the sine ratio to calculate its height. This method is commonly used by surveyors and construction workers.

Another practical application is in solar panel installation. The efficiency of solar panels depends on their angle relative to the sun's position. Installers use the sine ratio to calculate the optimal tilt angle for maximum energy capture, considering factors like latitude and seasonal variations in the sun's path.

In the field of sports, the sine ratio plays a role in analyzing projectile motion. Coaches and athletes use it to optimize the launch angle for activities like long jump, javelin throw, or basketball free throws. By understanding the relationship between the sine of the launch angle and the distance traveled, they can improve performance and technique.

The sine ratio is also crucial in seismology for analyzing earthquake waves. Geologists use it to determine the depth and location of earthquake epicenters by studying the angles at which seismic waves travel through the Earth's layers. This information is vital for understanding tectonic activity and improving earthquake prediction models.

In conclusion, the sine ratio's applications extend far beyond the classroom, playing a vital role in solving real-world problems across various disciplines. From towering skyscrapers to precise navigation systems, and from intricate optical devices to renewable energy solutions, the sine ratio continues to be an indispensable tool in our modern world. Understanding and applying this trigonometric concept opens up a wide range of possibilities in problem-solving and innovation across numerous fields.

Common Mistakes and Tips for Using Sine Ratio

When working with sine ratios in trigonometric ratios, students often encounter several common errors. Understanding these mistakes and learning how to avoid them can significantly improve your problem-solving skills. One frequent error is confusing the sine ratio with other trigonometric ratios like cosine or tangent. To avoid this, remember that sine is always the ratio of the opposite side to the hypotenuse in a right-angled triangle.

Another common misconception is when to use the sine ratio versus other trigonometric ratios. Students sometimes apply sine in situations where cosine or tangent would be more appropriate. To determine when to use sine, ask yourself if you're dealing with the opposite side and hypotenuse of a right triangle. If so, sine is likely the correct choice.

Remembering the sine ratio formula can be challenging for some students. A helpful mnemonic device is "SOH" from the acronym "SOHCAHTOA," where SOH stands for "Sine equals Opposite over Hypotenuse." Visualizing this relationship in a right triangle can reinforce your understanding and help you apply it correctly.

When solving sine ratio problems, a step-by-step approach can be invaluable. Start by clearly identifying the given information and what you need to find. Draw a diagram if possible, labeling the known sides and angles. Then, determine which side of the triangle corresponds to the opposite and which is the hypotenuse in relation to the given angle. Apply the sine ratio formula and solve for the unknown value.

If you're struggling with sine ratio problems, consider this troubleshooting guide: First, double-check that you've correctly identified the opposite side and hypotenuse. Next, ensure you're using the sine function on your calculator, not cosine or tangent. If you're solving for an angle, remember to use the inverse sine function (arcsin or sin¹) function. Always check if your answer makes sense in the context of the problem for instance, side lengths should be positive, and angles in a triangle should sum to 180 degrees.

Practice is key to mastering sine ratios. Work through a variety of problems, gradually increasing in difficulty. Pay attention to word problems that require you to identify when sine is the appropriate ratio to use. By focusing on these common errors, applying the tips provided, and consistently practicing, you'll develop a strong foundation in using sine ratios effectively in trigonometry.

Practice Problems and Solutions

To master the sine ratio, practice is essential. Here's a set of problems ranging from basic to advanced difficulty. Try solving these before checking the solutions!

Basic Level:

  1. In a right triangle, angle A is 30°, and the hypotenuse is 10 cm. Find the length of the opposite side.
  2. A ladder leaning against a wall forms a 60° angle with the ground. If the ladder is 8 meters long, how high up the wall does it reach?

Intermediate Level:

  1. A kite is flying at an angle of 45° to the ground. The string is 100 meters long. How high is the kite?
  2. From the top of a 50-meter tower, the angle of depression to a point on the ground is 35°. How far is this point from the base of the tower?

Advanced Level:

  1. Two observers spot a hot air balloon. Observer A sees it at an angle of elevation of 60°, while observer B sees it at 45°. If the observers are 1000 meters apart on level ground, how high is the balloon?
  2. A ship sails 100 km due east and then 60 km on a bearing of N30°E. Find the direct distance and bearing from the starting point to the final position.

Solutions:

Basic Level:

  1. Opposite = Hypotenuse × sin(30°) = 10 × 0.5 = 5 cm
  2. Height = Ladder length × sin(60°) = 8 × 0.866 6.93 meters

Intermediate Level:

  1. Height = String length × sin(45°) = 100 × 0.707 70.7 meters
  2. Distance = Tower height ÷ tan(35°) = 50 ÷ 0.7002 71.4 meters

Advanced Level:

  1. Using trigonometry and simultaneous equations: Height 866 meters
  2. Using vector addition and sine law: Distance 151.7 km, Bearing N51.3°E

Remember, practice makes perfect! For more sine ratio practice problems and trigonometry practice, check out online resources like Khan Academy, MathIsFun, or your textbook's problem sets. Don't hesitate to seek help from teachers or tutors if you're struggling with certain concepts. Keep challenging yourself with increasingly complex problems to improve your sine calculations and overall problem-solving skills in trigonometry.

Conclusion: Mastering Sine Ratio

In this article, we've explored the fundamental concepts of sine ratio in trigonometry. We've covered the definition of sine ratio as the ratio of the opposite side to the hypotenuse in a right-angled triangle, and how it relates to angles. We've walked through step-by-step examples of calculating sine ratios and solving problems involving unknown sides or angles. Understanding and practicing these calculations is crucial for success in trigonometry and higher-level mathematics. The ability to apply sine ratios extends beyond the classroom, finding applications in fields such as engineering, physics, and architecture. To solidify your grasp of these concepts, we encourage you to review the introduction video provided. This visual recap will help reinforce the key points and provide additional context for the material covered. Remember, mastering sine ratios is a building block for more advanced trigonometric concepts, so take the time to practice and fully comprehend these fundamental principles.

Example:

Draw and label a right triangle to illustrate sine ratio, then calculate the angle. sin⁡θ=513\sin \theta = {5 \over 13}

Step 1: Draw and Label the Right Triangle

To start, we need to draw a right triangle. A right triangle has one angle that is exactly 90 degrees. Label this angle as 90 degrees. Next, we need to label the sides of the triangle. The side opposite the right angle is the hypotenuse, which is the longest side of the triangle. In this case, the hypotenuse is given as 13 units. The side opposite the angle θ\theta is the opposite side, which is given as 5 units. The remaining side is the adjacent side, which we do not need to label for this problem.

Here is a simple illustration of the triangle:

            |\
            | \
            |  \
            |   \
            |    \
            |     \
            |______\

Label the hypotenuse as 13 and the opposite side as 5. The right angle is at the bottom left corner.

Step 2: Understand the Sine Ratio

The sine ratio is defined as the ratio of the length of the opposite side to the length of the hypotenuse. Mathematically, it is expressed as:

\sin \theta = \frac{opposite}{hypotenuse}

In this problem, we are given that sin⁡θ=513\sin \theta = \frac{5}{13}. This means that the opposite side is 5 units and the hypotenuse is 13 units.

Step 3: Calculate the Angle Using Inverse Sine

To find the angle θ\theta, we need to use the inverse sine function, which is often denoted as sin⁡−1\sin^{-1} or arcsin. This function will allow us to find the angle whose sine is 513\frac{5}{13}.

Using a calculator, enter the inverse sine function and input the ratio 513\frac{5}{13}. Most calculators have a button for the inverse sine function, which may be labeled as sin⁡−1\sin^{-1} or arcsin. You may need to use the shift or second function key to access it.

Enter the following into your calculator:

sin⁡−1(513)\sin^{-1}\left(\frac{5}{13}\right)

After calculating, you should find that θ\theta is approximately 23 degrees.

Step 4: Verify the Angle

To ensure the accuracy of our calculation, we can verify that the sum of the angles in the triangle adds up to 180 degrees. In a right triangle, the two non-right angles must add up to 90 degrees because the right angle is 90 degrees by itself.

We have calculated one of the angles to be 23 degrees. Therefore, the other angle must be:

90−23=6790 - 23 = 67 degrees

So, the angles in the triangle are 90 degrees, 23 degrees, and 67 degrees, which add up to 180 degrees, confirming our calculation is correct.

Conclusion

By following these steps, we have successfully drawn and labeled a right triangle to illustrate the sine ratio and calculated the angle θ\theta to be 23 degrees. This process involves understanding the sine ratio, using the inverse sine function, and verifying the results to ensure accuracy.

FAQs

Here are some frequently asked questions about sine ratios:

1. What is the sine ratio?

The sine ratio is a trigonometric function defined as the ratio of the length of the opposite side to the length of the hypotenuse in a right-angled triangle. It is expressed as sin(θ) = opposite / hypotenuse, where θ is the angle in question.

2. How do you calculate the sine ratio?

To calculate the sine ratio, divide the length of the side opposite to the angle by the length of the hypotenuse. For example, if the opposite side is 3 units and the hypotenuse is 5 units, the sine ratio would be 3/5 or 0.6.

3. When should you use the sine ratio?

Use the sine ratio when you need to find an unknown side length (opposite or hypotenuse) or angle in a right-angled triangle, given one side length and one angle. It's particularly useful in problems involving height, distance, or angle calculations.

4. What is the relationship between sine and cosine ratios?

Sine and cosine are complementary functions. For any angle θ in a right-angled triangle, sin(θ) = cos(90° - θ). This relationship is known as the co-function identity. Additionally, sin²(θ) + cos²(θ) = 1, which is the Pythagorean identity.

5. Is the sine ratio always less than 1?

Yes, in a right-angled triangle, the sine ratio is always less than or equal to 1. This is because the opposite side can never be longer than the hypotenuse. The sine ratio equals 1 only when the angle is 90°, at which point the opposite side and hypotenuse have the same length.

Prerequisite Topics

Understanding the sine ratio to calculate angles and sides (Sin = o / h) is a crucial concept in trigonometry. However, to fully grasp this topic, it's essential to have a solid foundation in several prerequisite areas. These foundational concepts not only provide context but also enhance your ability to apply the sine ratio effectively.

One of the most closely related topics is using the cosine ratio to calculate angles and sides. This concept is vital for solving right triangle problems and complements the sine ratio. By understanding both sine and cosine, you'll be better equipped to tackle a wider range of trigonometric challenges.

Similarly, using the tangent ratio to calculate angles and sides is another essential skill. This knowledge helps in applying the angle sum property of triangles and provides a comprehensive understanding of trigonometric ratios.

To truly master these concepts, you should be familiar with combinations of SohCahToa questions. This practice will enhance your problem-solving skills and teach you about using a scientific calculator for sine calculations, which is invaluable for more complex problems.

Another crucial prerequisite is converting between degrees and radians. This skill is fundamental in trigonometry and is often required when working with sine ratios, especially in more advanced applications.

Understanding angles of elevation and depression is also important. This concept frequently appears in real-world applications of trigonometry and helps in solving angle of elevation problems, which often involve sine ratios.

Lastly, familiarity with finding inverse reciprocal trigonometric functions from graphs can be beneficial. This knowledge aids in understanding the inverse sine function usage, which is an extension of the basic sine ratio concept.

By mastering these prerequisite topics, you'll build a strong foundation for understanding and applying the sine ratio. Each concept contributes to a more comprehensive grasp of trigonometry, allowing you to approach problems with confidence and versatility. Remember, trigonometry is a interconnected field, and understanding these relationships will significantly enhance your problem-solving abilities and overall mathematical comprehension.

Detail Information
Basic Concepts
?
Related Concepts
?