In this lesson, we will learn:
- To understand how calorimetry is used to find enthalpy changes in chemical reactions.
- To recall the equation for heat absorbed or released during a chemical process.
- To use the calorimetry equation to calculate heat energy transferred in simple chemical processes.
- To use the calorimetry expression and the first law to find enthalpy changes of chemical reactions.
Notes:
- If you have heard the word calorie before, then you already know half of what calorimetry is about: energy.
Calorimetry measures the amount of heat energy released or absorbed by a substance from a chemical process.
We know that chemical reactions absorb and release heat energy, so this is one way of finding enthalpy changes that happen due to a chemical process. This is good for chemists because we have a way to measure the average heat energy of substances. Its called the temperature! - Calorimetry works because of a property of any material called the specific heat capacity.
Specific heat capacity is the energy (in joules) needed to raise the temperature of 1 gram of any substance by 1 K (or 1°C).
Most calculations involve heating water, which has a specific heat capacity of 4.18 J g-1 K-1 (joules per gram per kelvin). This means you need 4.18 joules of energy to heat one gram of water by 1 K. This 4.18 J of energy is equal to 1 calorie. In the same way, 4.18 kJ (kilojoule) is 1 kcal (kilocalorie), which are the calories people talk about with food and diet. - The amount of heat given off or absorbed in a reaction is given by the equation::
- WORKED EXAMPLE: A 50mL sample of water in an insulated cup was heated, making the water temperature rise by 13oC. How much energy did the water absorb? Give your answer in kJ to three significant figures.
- Your answers in these calculations contain some error. There are a few sources of error:
- The biggest source of error is the fact that some heat from the reaction is lost to the surroundings this heat was also produced by the reaction taking place but because it escaped it is not raising the temperature of the water, so the calculation ignores it. For this reason, the energy calculated using the calorimetry expression is probably lower than the real value, which would include heat lost to the surroundings.
- Completely pure water has a density of 1 g / mL but most water has some mineral ions or other impurities in it, which changes its density. If a solution is being heated, the water solvent is obviously not pure it has solute in it. Both of these instances mean that the density wont be exactly 1 g / mL so some error is introduced when you convert from mL to g.
- Calorimetry calculations can be used to find the enthalpy change of several processes, such as the enthalpy of solution or combustion..
This is because of the first law of thermodynamics. The first law is about conservation of energy; energy cannot be created or destroyed in a system, only transferred between objects in the system.
This means if one object in a system gains some heat energy (measured as a temperature increase), that comes with an equal loss of heat energy from something else.
An example might be the decrease of water temperature when an ice cube is put into water. We can measure the temperature drop of the water surroundings to find the heat energy loss, which corresponds to the heat energy gain of the ice cube. In an equation: - Remember that calorimetry calculations make the assumption that no heat energy is lost to the surrounding environment; it is all absorbed by the water, none to the walls of the beaker, or to the air around a spirit burner, for example.
This is practically impossible. Depending on the experiment, at least some heat is lost to the surroundings other than what you are measuring the temperature of. Your Qwater value will be lower than the real value, so any value you calculate will also be lower than the real value. Calorimetry estimates the Q and values, its not perfect!
Where:
Q = energy (J)
M = mass (g)
C = specific heat capacity (J g-1 K-1)
= change in temperature (K)
When doing these calculations, exam questions will often give you information about the substance (e.g. water or an aqueous solution) by its volume in mL. Assume that the density of the water or solution is 1 g / ml unless told otherwise, so the value in g will be the same as the value in g.
Be careful with units! It is almost always appropriate for a reaction on a laboratory scale to quote an enthalpy value in kJ mol-1 and this Q value will be in J initially.
Using our equation:
We need to find energy (Q) and are given the volume of water (50mL), and the change in temperature. The specific heat capacity of water is 4.18 J g-1 K-1 memorise this value!
Assuming the density of water is 1 g / mL, we have 50 g of water. The calculation then becomes:
Remember you are finding energy (J): your units should cancel for J on the right-hand side of the equation!
Look carefully at how your answer needs to be given. Calorimetry questions often need to be calculated in J but converted to kJ with significant figures.
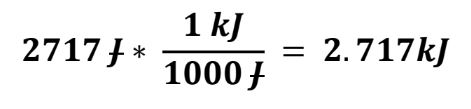
Round to three significant figures gives the final answer of 2.72 kJ
This is also true of chemical processes like dissolving. For example, an acid dissolving in water to make a solution leads to a temperature increase in the water. We could write a variation of the above equation:
If we know the quantity of acid dissolved to make this temperature change, we can find an enthalpy change per mole of acid/base that was caused by the dissolving process this is the enthalpy change of solution!
We can also use this to find the enthalpy of combustion of a fuel, such as one being used in a spirit burner. Any heat energy gained by water to raise its temperature has been provided by the fuel, which has lost that heat in the process. The heat is just transferring from one object to its surroundings.
Again, with the quantity of fuel used, and the temperature change of the water, we can find an enthalpy change of combustion (fuel reacting with oxygen).






