In this lesson, we will learn:
- How to use experimental data to produce graphs that show the order of reaction.
- How to use data and graphs to build a rate law equation for a reaction.
- How to use the integrated rate law to find the half-life of a first-order reaction.
- How to use the rate law equation to deduce a rate-determining step and mechanism.
- How to use the Arrhenius equation to find the activation energy of a reaction.
- How to use the steady-state approximation to derive a rate equation.
Notes:
- As we saw in Reaction-mechanisms, different reactions have different orders of reaction. This means their rate depends on the concentration of their reactants to varying degrees. This is shown in the rate law that can be written for any one-step or elementary reaction. For example:
- A reaction that is first order with respect to reactant A: if you double the concentration of A, you will double (the result of 21) the rate. The power x1 is why it is called first order.
- A reaction that is second order with respect to reactant A: if you double the concentration of A, you will quadruple (the result of 22) the rate. The x2 is why it is called second order.
- The methods to find the order of the reaction all start by measuring the change in concentration over time. Three graphs need to be made:
- A graph of concentration over time ([A] vs. t)
- A graph of the natural log of concentration over time (ln[A] vs. t)
- A graph of the inverse (1/x) of concentration over time (1/[A] vs. t)
- For some reactions, you will get a simple linear graph with a constant slope when plotting concentration, [A], over time.
Graphically we are being told that despite there being more [A] at the beginning of the reaction, this does not speed it up as the rate of decrease of [A] is constant. Therefore, the rate of the reaction doesn’t depend on [A] at all.
This a zero-order reaction, with respect to A. Since x0 = 1, [A] is not in the rate law expression: - In other reactions, when you plot [A] over time, you get a curve which starts steep at the beginning of the reaction and levels out near the end.
Graphically, this tells you that reactant A is being used up quicker at the beginning than at the end of the reaction. Early on, more reactant A is available than later, so there are more successful collisions of reactant A. Therefore, the drop in [A] is greater earlier on. - Other reactions will have a direct relationship between [A] and rate, but even more dependent on [A] than first-order. This is somewhat noticeable in plotting [A] over time with very large initial drops that flattens rapidly afterward:
- Using the integrated rate law for a first-order reaction, we can find the half-life of any first-order reaction, which does not depend on the initial concentration.
- The half-life of a reaction is the time taken for a reaction to use up half of the original reactant concentration, or when half of the original concentration is still left.
- Remember that a reaction mechanism is a theory, but experimental evidence is more important than theory!
Any mechanism proposed must agree with the experimental rate law, which we build up from our experiments of changing the reactant concentrations, conditions, and so on.
For example, the reaction of NO2 with CO can be described in the equation below: - The elementary steps cancel out to give the overall balanced equation above:
- It agrees with the experimental rate law – the reaction is second-order with respect to NO2 because there are two NO2 molecules involved in the RDS. The intermediate NO3 and CO are not involved in the RDS, so they appear zero order.
- Through these kinetics experiments and others, chemists have found rate laws for many reactions and developed different types of reaction mechanisms which are of importance in organic chemistry:
- SN1 reactions, where the rate-determining step involves only one molecule.
- SN2 reactions, where the rate-determining step involves two molecules.
- Just like finding the half-life of a reaction, we can use the natural logarithm to find the activation energy of reactions using the Arrhenius equation:
- Another method of finding the overall rate equation is the steady-state approximation. This assumes that any intermediates in the reaction are used up as fast as they are produced, so their concentration doesn’t change.
This simplifies the calculations made about the overall rate of the process. A steady state is not the same as equilibrium - equilibrium is a type of steady state.
The steady-state method can be used when the first step in a multi-step process is the slow step.
For example, in the reaction:
The following points will look at analyzing and graphing rate data for “reactant A”.
See the three graphs below for such a reaction:
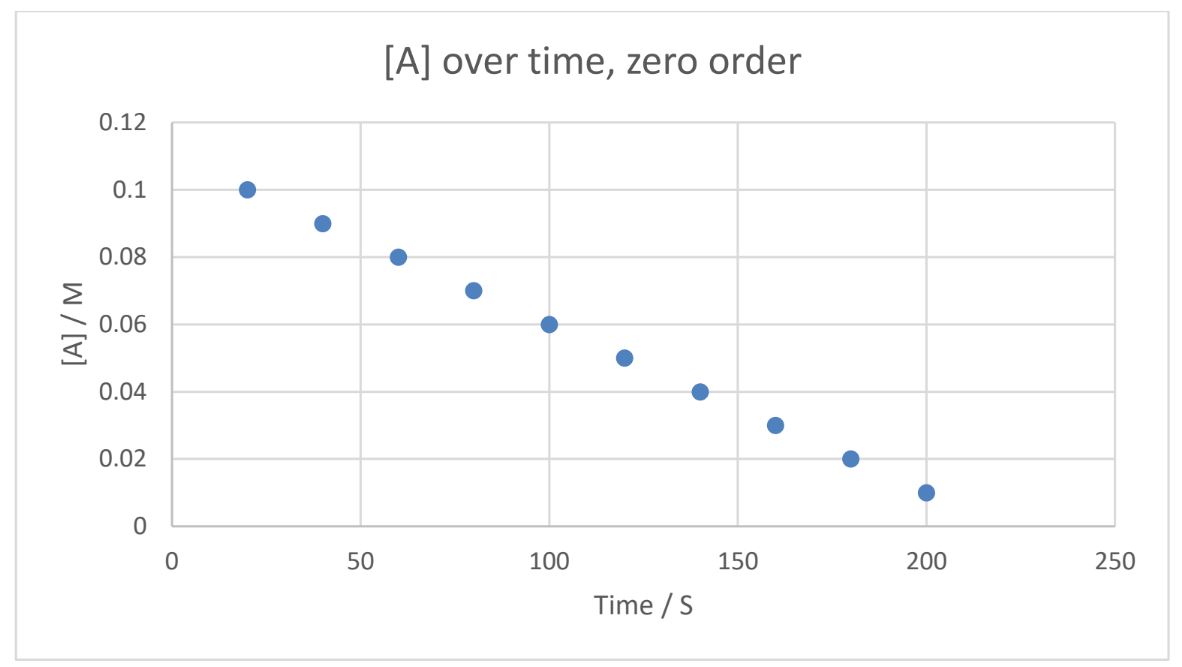
The slope of the line here is equal to -k, the negative of the rate constant.
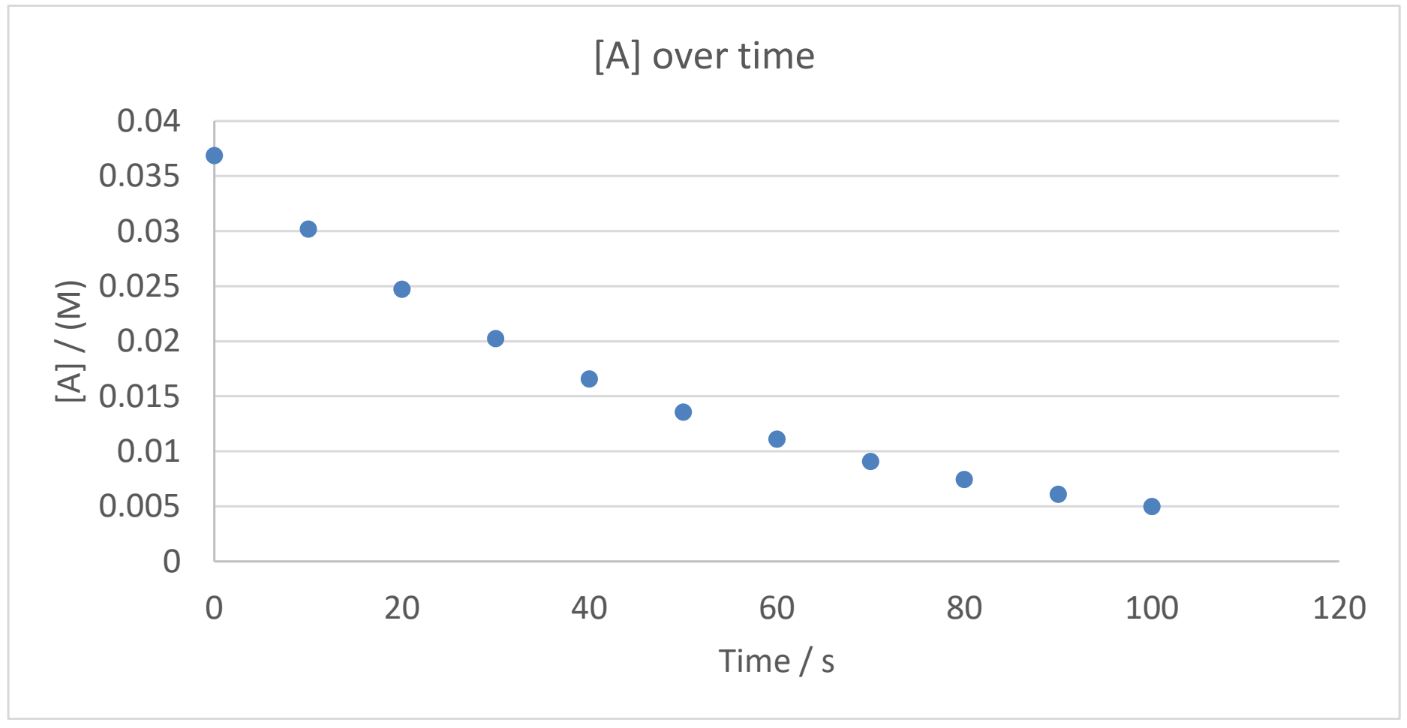
As product forms and less reactant A becomes available over time, the rate will decrease in a linear fashion; the slope flattens out. For example, if [A] is doubled, the rate will double. This is a first order reaction with respect to reactant A.
The rate law will include [A]1 in this case, or just [A]:
Since concentration is proportional to rate, plotting ln[A] over time for a first-order relationship gives a typical straight-line graph like the one below. This is typical of a first-order rate dependence:
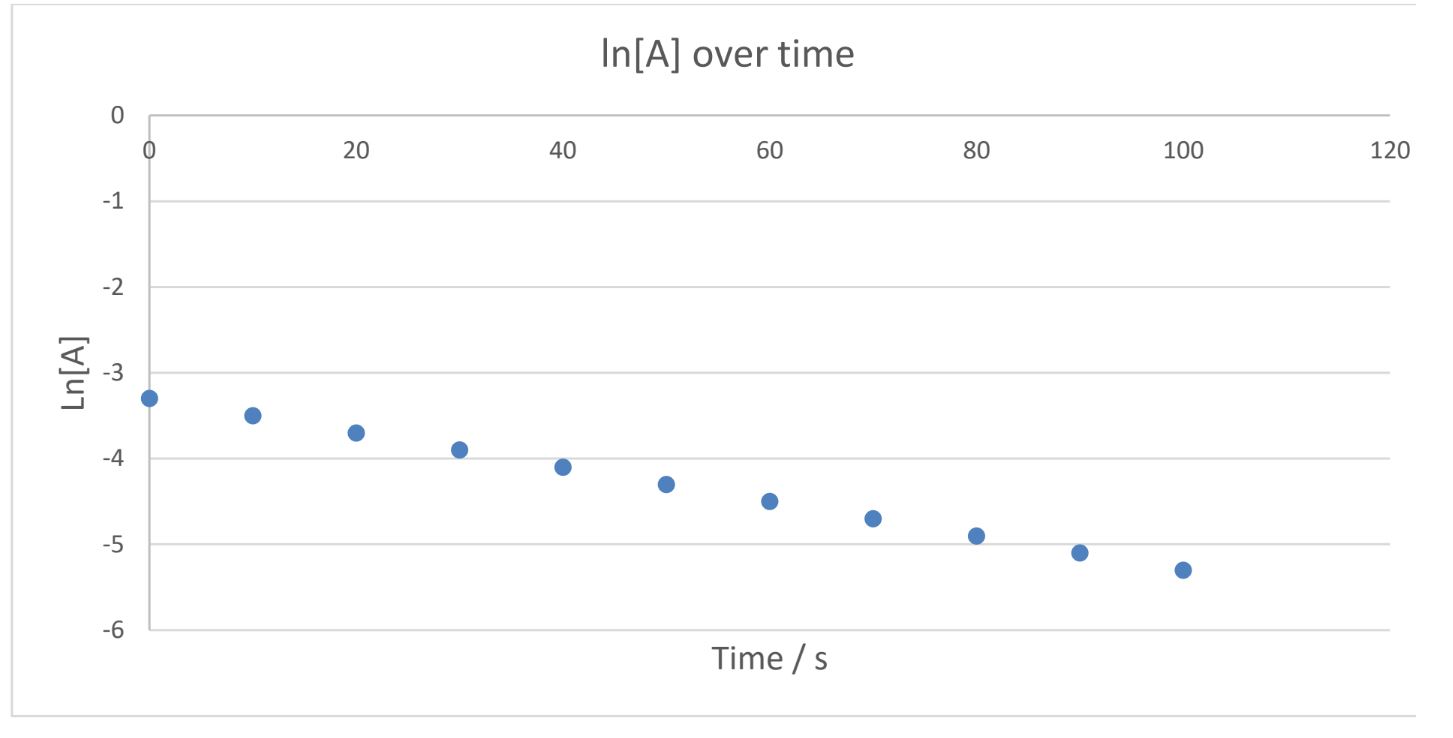
The slope of this straight line is equal to -k, the negative of the rate constant.
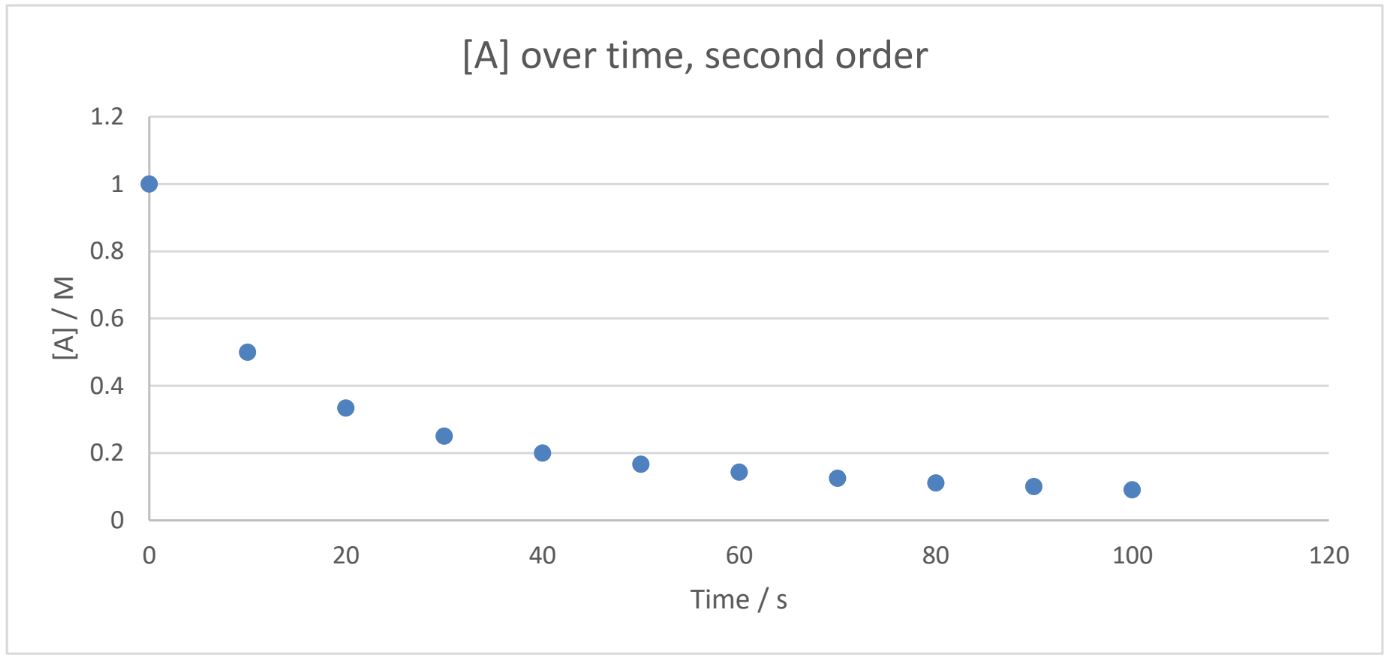
If plotting ln[A] over time results in a curve, plot 1/[A] over time instead. If you get a straight line, this is typical of a second order reaction with respect to a reactant. This means the rate law will include [A]2 – if you double [A], you will increase the rate by a factor of 22.
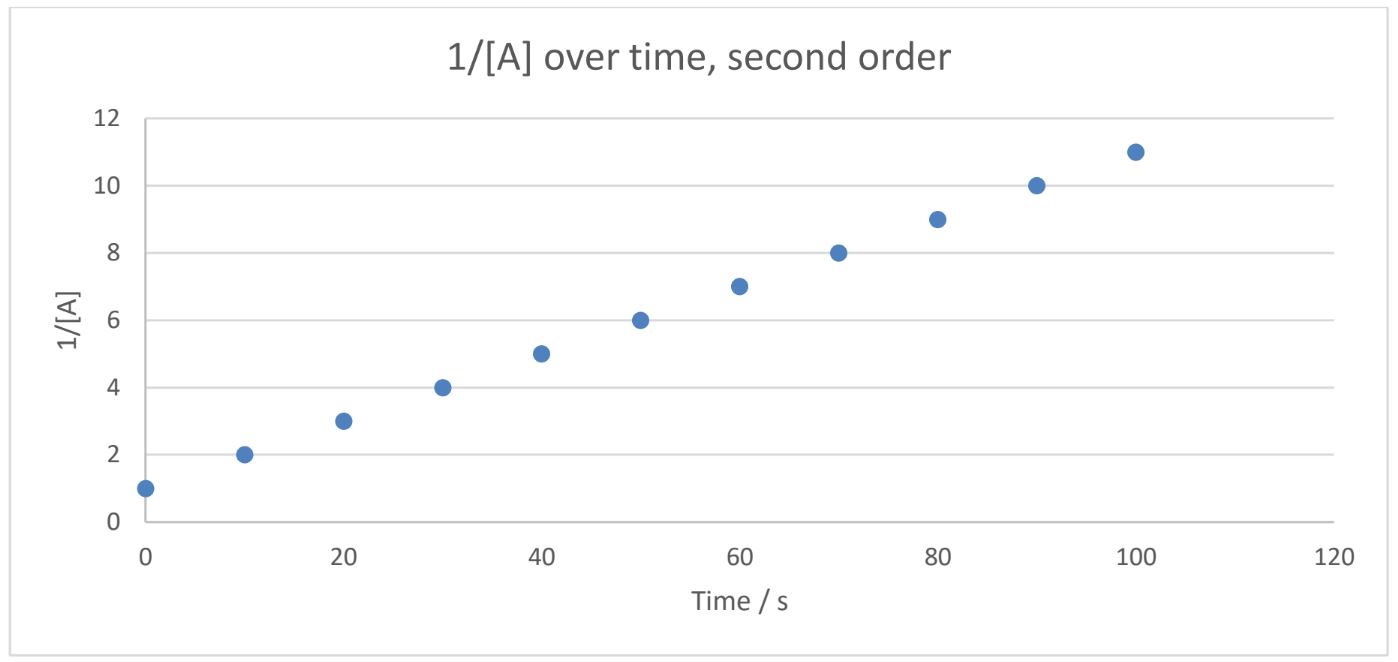
Here, the rate constant k is equal to the slope of the straight line.
A property of natural logarithms is that ln[x] – ln[y] can be expressed as ln[x/y]. Rearranging, then:
Taking the exponent of both sides:
At t = 0, before the reaction has started, we expect that [A]t = [A]0 and we can prove it:
Multiplying both sides by [A]0:
At the half-life, t1/2, we know [A] is half of the original concentration, [A]0
Therefore, plugging this into our previous expression, we can cancel out [A]0:
We are left with:
Taking the natural logarithm of both sides:
This leaves us with a simple equation to find the half-life of a first-order reaction that only depends on the rate constant:
Suppose that a chemist says the mechanism is that this reaction happens all in one step – one NO2 molecule and one CO molecule colliding to produce the NO and CO2 products.
This can be tested and if it is true, that single step would be the rate-determining step and our experiments must show that the reaction is first order with respect to NO2, and first order with respect to CO.
This is not observed in experiments. Because our mechanism does not agree with the experimental rate law, this mechanism must be incorrect. Experimentally, you can find that the rate law is second order with respect to NO2 and zero-order with respect to CO. With this, we build the theory that the rate-determining step does not involve CO, and so there must be more than one-step. If CO is not involved in it, NO2 must be. Proposing another mechanism:
This mechanism fulfills two key conditions:
Where:
k = rate constant
A = pre-exponential factor, which accounts for particles colliding and their orientation.
Ea = activation energy, measured in J mol-1
R = the universal gas constant, 8.31 J K-1 mol-1
T = temperature, measured in Kelvin
Taking the natural logarithm of both sides gives us:
A property of natural logs is that ln[x.y] can be written as ln[x] + ln[y], in the same way that ln[x/y] can be written as ln[x] – ln[y].
Another property of natural logs is any exponent of their subject can be written in front of it – as a coefficient:
The natural logarithm of the exponential function is equal to 1, so this cancels out (see above). We now have:
If we move ln[A] behind the Ea/RT term, and split this term apart, we can make:
This above equation fits the form y = mx + c, so it can be plotted as a straight line graph.
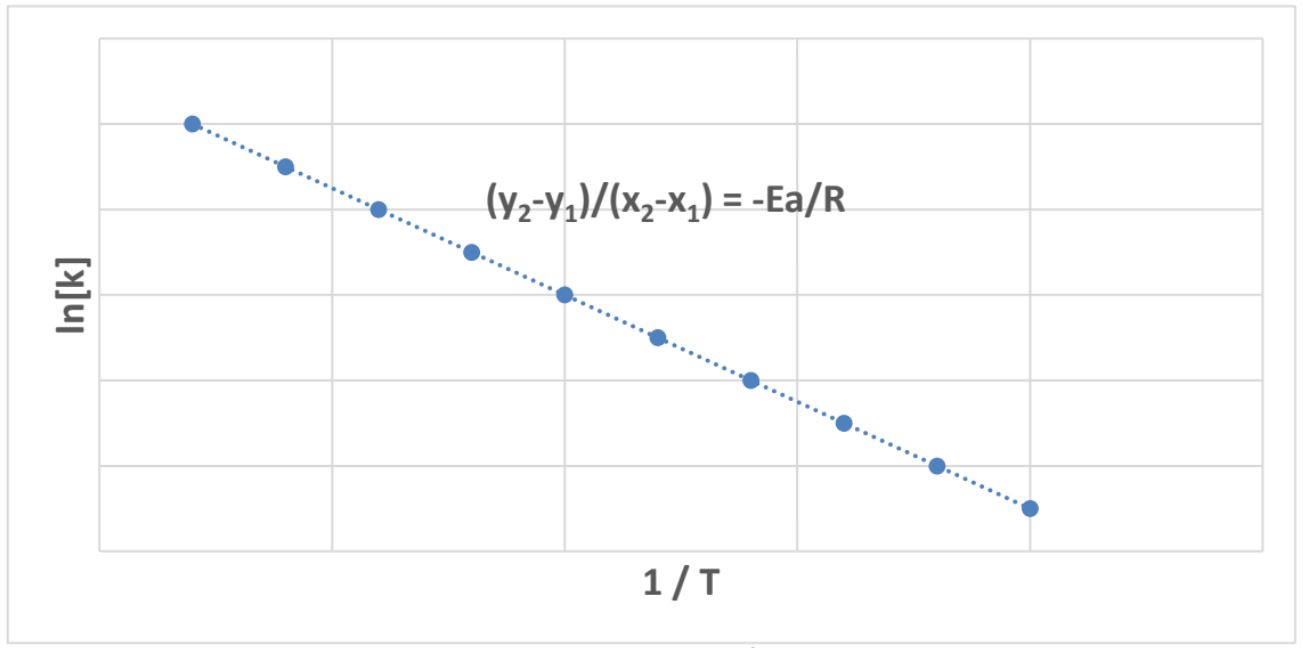
This is useful because it allows for multiple experiments to be performed where temperature (and therefore 1/T) is changed, and a different rate constant (and therefore ln[k]) is obtained. This lets us plot the graph, calculate the slope and find the activation energy.
Which has two steps:
Applying the steady-state approximation lets us assume that:
Because k2 is the fast step, k2 >>> k-1, we can approximate k-1 = 0 We can now solve this equation for:
And:
We now have a final equation for the rate of reaction to make product C:






