Torque and rotational inertia  - Rotational Motion

Notes:

In this lesson, we will learn:

• Definition of Torque
• Translational equilibrium Vs. Rotational equilibrium
• Rotational Inertia
• Moment of inertia of uniform objects

Notes:


Torque

• To have better understanding of the meaning of “torque”, let’s try the following activity and compare the motion of object as different forces are exerted.
• A ruler is placed on a horizontal flat surface.

(a) The force is applied at the center of mass

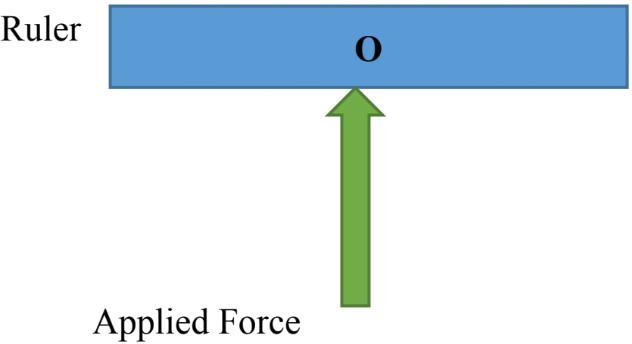
$\quad$The whole object (ruler) will accelerate in the direction of the force exerted.

(b) The force is applied away from the center of mass

The whole object (ruler) will rotate about the center of mass.


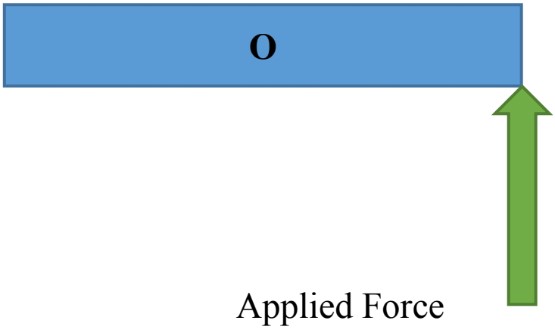
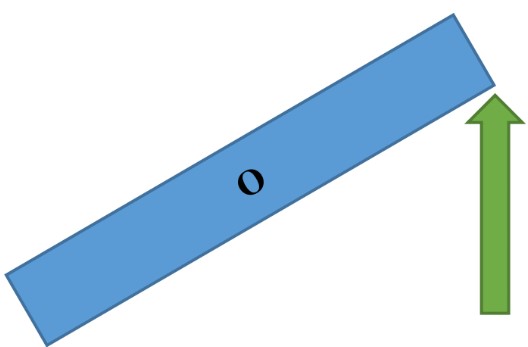
As the result of exerting force, the object will rotate about the “Pivot” or “Axis of Rotation”.

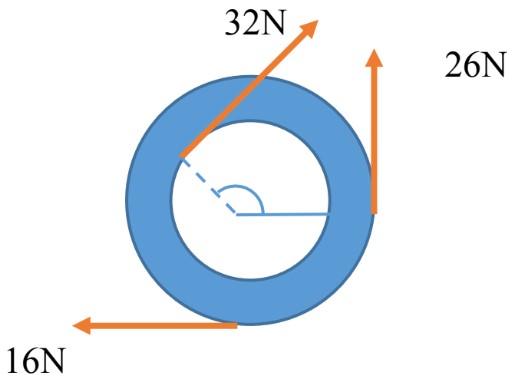
Therefore, we can define Torque as the “Turning Effect of a Force”.

Torque is represented by the Greek letter $\tau$ and the standard unit is $N.m.$


Conclusion:

• Force causes acceleration; $F=ma$


• Torque causes angular acceleration: $\tau = r F \sin \theta \;$ (vector quantity)
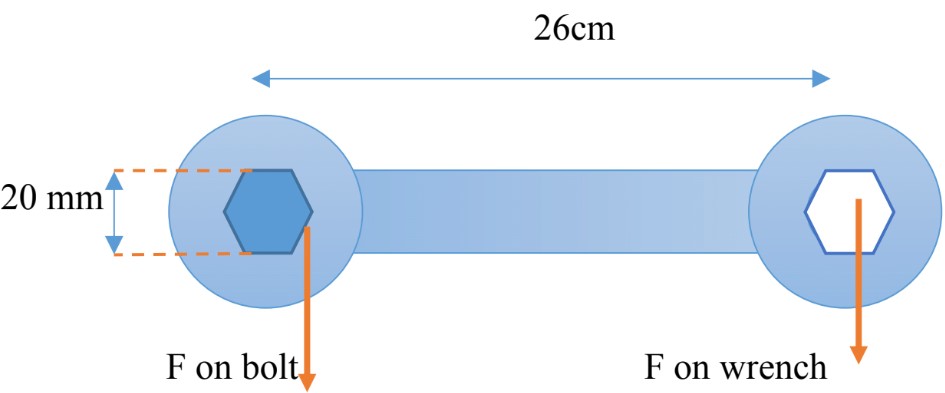
  $\theta$ : angle between $r$ and $F$
  $r$: distance between the pivot and point at which the force is exerted on.

$\tau \, \propto \, r$: the further the force, the bigger the torque
$\tau \, \propto \, \theta$: the larger the angle, the bigger the torque 

The object could be in Translational Equilibrium and Rotational Equilibrium;

$\sum F = 0 \qquad \qquad$ “Translational Equilibrium” $\; \Rightarrow \,$ acceleration is zero
$\sum \tau = 0 \qquad \qquad$ “Rotational Equilibrium” $\; \Rightarrow \,$ angular acceleration is zero


Rotational Inertia
Let’s consider a mass m rotating in a circle of radius r about a fixed point. The object is going to experience tangential and angular acceleration.


$I = mr^{2} \quad$ (scalar quantity) Unit: kg.m²

“Rotational Inertia represents the resistance to angular acceleration”

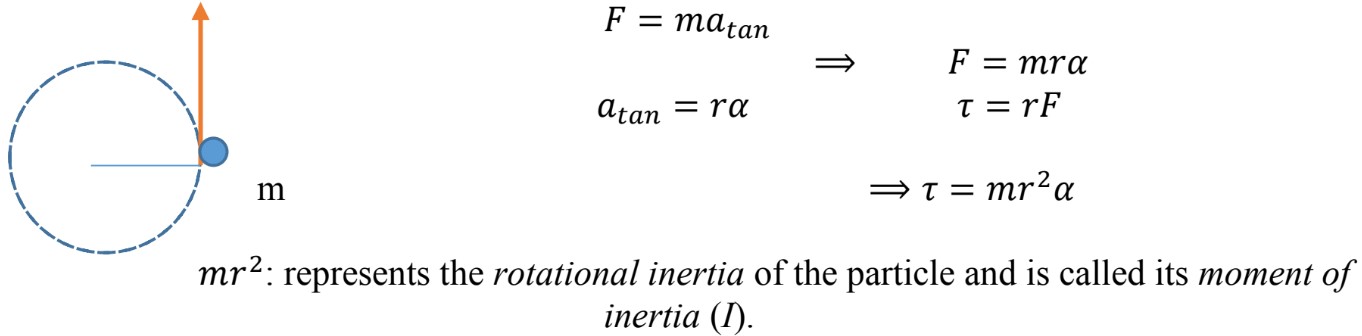
• Large Rotational Inertia: the mass of the object is distributed far from the axis of rotation.
  Objects with larger Rotational Inertia are harder to get rotating and harder to stop rotating.
• Small Rotational Inertia: the mass of the object is distributed close to the axis of rotation.

$I = mr^{2} \qquad$ (single mass rotating of a single radius)
$I = \sum mr^{2} \enspace \;$ (multiple individual masses rotating in circles of different radius)

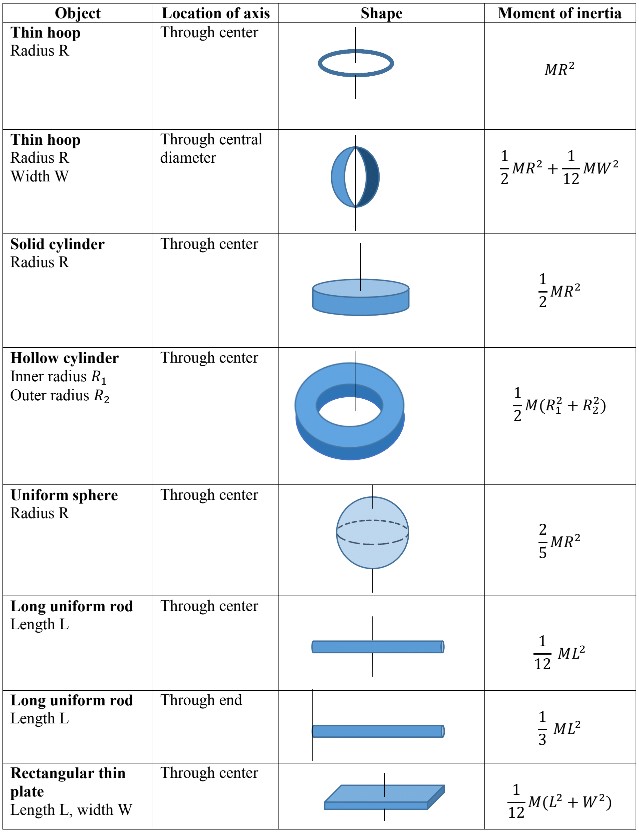
• Moment of inertia for objects of uniform composition is constant;