1. Home
  2. >Physics
  3. >Special Relativity
Help

Postulate of the special theory of relativity, time dilation and twin paradox

?
Intros
Lessons
  1. Twin Paradox
?
Examples
Lessons
    1. Calculate the mean lifetime of a moving muon, knowing that it travels at a speed of 0.80c with respect to the laboratory. Its mean life time is 4.4μ\mus when is at rest.

    2. Find the distance travelled by the muon before decaying
    Topic Notes
    ?

    Introduction

    The twin paradox, a fascinating concept in the special theory of relativity, illustrates the mind-bending effects of time dilation. This thought experiment involves one twin embarking on a high-speed space journey while the other remains on Earth. Upon the traveler's return, they find their Earth-bound sibling has aged more, demonstrating how time can flow differently for objects moving at different speeds. Time dilation, a key principle in Einstein's theory, explains how time slows down for fast-moving objects relative to stationary ones. Our introduction video delves into these complex ideas, offering a clear and engaging explanation of the twin paradox and time dilation. By visualizing these concepts, viewers gain a deeper understanding of how space and time are interwoven in the fabric of our universe. The video serves as an excellent starting point for those curious about the counterintuitive nature of relativistic physics and its profound implications for our perception of time and space.

    Understanding Time Dilation

    Time dilation is a fascinating concept in physics that challenges our everyday understanding of time. It refers to the phenomenon where time passes at different rates for observers in different reference frames, particularly when one is moving at high speeds relative to the other. This effect is a key prediction of Einstein's theory of special relativity and has been experimentally verified numerous times.

    To understand time dilation, we must first grasp the idea of reference frames. A reference frame is a coordinate system used to describe the position and motion of objects. In everyday life, we typically use Earth as our reference frame. However, in physics, we can consider any object or observer as a reference frame.

    The core principle of time dilation states that as an object moves faster relative to another, time slows down for the moving object from the perspective of the stationary observer. This effect becomes noticeable at speeds approaching the speed of light. It's important to note that each observer in their own reference frame experiences time normally; the difference only becomes apparent when comparing the two frames.

    To illustrate this concept, let's consider the example of delta t and delta t zero. Imagine two identical clocks, one stationary on Earth (clock A) and one on a spacecraft moving at high speed (clock B). Delta t represents the time measured by the stationary clock on Earth, while delta t zero represents the time measured by the moving clock on the spacecraft.

    As the spacecraft moves at high speed, an observer on Earth would notice that clock B appears to tick more slowly than clock A. This means that delta t zero (the time measured on the spacecraft) is less than delta t (the time measured on Earth). The faster the spacecraft moves, the greater this time difference becomes.

    The mathematical relationship between delta t and delta t zero is given by the Lorentz factor, which depends on the relative velocity between the two reference frames. As the velocity approaches the speed of light, the time dilation effect becomes more pronounced.

    It's crucial to understand that time dilation is not just a theoretical concept but a real phenomenon with practical implications. For example, GPS satellites must account for time dilation effects to maintain accurate positioning. The atomic clocks on these satellites run slightly faster than clocks on Earth due to their high orbital speeds and reduced gravitational influence.

    Time dilation also plays a role in particle physics experiments. Muons, subatomic particles created in the upper atmosphere, should decay before reaching Earth's surface based on their known lifetime. However, due to time dilation caused by their near-light speeds, they survive long enough to be detected at ground level.

    The concept of time dilation leads to interesting thought experiments, such as the famous "twin paradox." In this scenario, one twin stays on Earth while the other travels on a high-speed space journey. Upon return, the traveling twin would have aged less than the Earth-bound twin due to time dilation.

    Understanding time dilation requires us to abandon our intuitive notions of time as an absolute, universal constant. Instead, we must embrace the idea that time is relative and can be influenced by motion and gravity. This concept, while challenging, opens up new perspectives on the nature of our universe and continues to inspire scientific research and philosophical discussions about the nature of time itself.

    The Twin Paradox Explained

    The twin paradox is a fascinating thought experiment in special relativity that demonstrates the effects of time dilation on aging. It involves two identical twins, one who stays on Earth and another who embarks on a high-speed space journey. This paradox illustrates how time can pass differently for two observers in different reference frames, leading to a significant age difference upon reunion.

    Let's consider the example of 20-year-old twins, Alice and Bob. Alice remains on Earth, while Bob sets off on a space voyage at near-light speed. According to Einstein's theory of special relativity, time slows down for objects moving at high velocities relative to a stationary observer. This phenomenon is known as time dilation.

    In our scenario, Bob, the astronaut twin, travels at 99% the speed of light (0.99c) to a star system 10 light-years away. From Earth's perspective, the round trip would take approximately 20 years. However, due to time dilation, Bob experiences time differently during his journey.

    To calculate the time experienced by Bob, we use the Lorentz factor (γ), given by the equation:

    γ = 1 / (1 - v²/c²)

    Where v is the velocity of the spacecraft and c is the speed of light. For Bob's journey at 0.99c:

    γ = 1 / (1 - 0.99²) 7.09

    The time experienced by Bob (t') is related to the time passed on Earth (t) by the equation:

    t' = t / γ

    For the 20-year round trip as measured on Earth:

    t' = 20 years / 7.09 2.82 years

    This means that while 20 years pass for Alice on Earth, Bob only experiences about 2.82 years during his space travel. When Bob returns to Earth, he will have aged only 2.82 years, while Alice will have aged the full 20 years.

    The age difference upon Bob's return can be calculated as follows:

    Age difference = Time passed on Earth - Time experienced by Bob
    Age difference = 20 years - 2.82 years 17.18 years

    Therefore, when Bob returns from his space journey, he will be approximately 22.82 years old (20 + 2.82), while Alice will be 40 years old. The twins, who were once the same age, now have an age difference of about 17.18 years.

    This outcome may seem paradoxical because, from Bob's perspective, he might argue that Alice was the one moving away at high speed. However, the key to resolving this paradox lies in the fact that Bob's reference frame is not inertial throughout the journey. He experiences acceleration during departure, deceleration upon arrival at the distant star system, and again during the return trip. These changes in velocity break the symmetry between the twins' experiences, making Bob's journey fundamentally different from Alice's stationary state on Earth.

    The twin paradox has been confirmed through various experiments, including the Hafele-Keating experiment in 1971, where atomic clocks were flown around the world on commercial airliners. Although the time differences were much smaller due to lower speeds, they aligned with the predictions of special relativity.

    Understanding the twin paradox is crucial for space travel and GPS technology. GPS satellites, orbiting at high speeds relative to Earth's surface, experience time dilation effects that must be accounted for to maintain accurate positioning. Without these relativistic corrections, GPS systems would accumulate errors of up to 10 kilometers per day.

    In conclusion, the twin paradox vividly illustrates the profound implications of Einstein's theory of special relativity on our understanding of time and aging. It demonstrates that time is not absolute but relative, depending on the observer's motion. This concept challenges our intuitive notions of time and space, opening up new perspectives on the nature

    Relationship Between Speed and Time

    The relationship between an object's speed relative to the speed of light and the passage of time is a fascinating concept in physics, known as time dilation. As an object's velocity approaches the speed of light, time passes more slowly for that object compared to a stationary observer. This phenomenon, predicted by Einstein's theory of special relativity, challenges our everyday understanding of time and space.

    To comprehend this concept, it's crucial to understand that the speed of light is constant in all reference frames. As an object moves faster, it experiences time at a different rate than stationary objects. This time slowdown becomes more pronounced as the object's speed gets closer to the speed of light.

    Let's consider some examples to illustrate this concept. Imagine two identical clocks, one on Earth and one on a spacecraft traveling at 99% of the speed of light. The clock on the spacecraft would tick more slowly compared to the Earth-bound clock. If the spacecraft's journey lasted one year from the perspective of the crew, several years might have passed on Earth.

    Another example involves muons, subatomic particles created in the upper atmosphere by cosmic rays. These particles have a very short lifespan and should decay before reaching the Earth's surface. However, because they travel at speeds close to the speed of light, time slows down for them. This time dilation effect allows muons to reach the Earth's surface before decaying, a phenomenon that has been experimentally verified.

    The time slowdown effect becomes more dramatic as the relative speed increases. At 50% of the speed of light, time would pass about 15% slower for the moving object. At 90% of light speed, time would slow down by a factor of more than 2. As the speed approaches 99.99% of light speed, time would pass about 100 times more slowly.

    This concept has practical implications in modern technology. GPS satellites, which orbit the Earth at high speeds, experience time dilation effects. Their clocks run slightly faster than clocks on Earth due to their speed and position in Earth's gravitational field. Without accounting for these relativistic effects, GPS systems would quickly become inaccurate.

    It's important to note that this time slowdown is not just a theoretical concept but a real, measurable phenomenon. Experiments using precise atomic clocks on airplanes have confirmed the predictions of special relativity. The faster the plane travels, the slower its onboard clock ticks relative to a stationary clock on the ground.

    The implications of this speed-time relationship extend to hypothetical scenarios of space travel. If humans could travel at speeds close to light speed, they would experience less time passage than those left behind on Earth. This concept, often explored in science fiction as "time dilation," is based on real physics principles.

    Understanding the relationship between speed and time passage is crucial in fields like astrophysics, where scientists study objects moving at extreme velocities. It also plays a role in particle physics experiments, where subatomic particles are accelerated to speeds very close to the speed of light.

    In conclusion, the speed of an object relative to the speed of light profoundly affects the passage of time. This time slowdown effect, while counterintuitive to our everyday experience, is a fundamental aspect of the universe's nature. It reminds us that time is not absolute but relative, depending on the observer's frame of reference and motion. As we continue to explore the cosmos and push the boundaries of technology, understanding these principles becomes increasingly important in our quest to comprehend the fundamental workings of the universe.

    Implications of the Twin Paradox

    The twin paradox, a cornerstone of Einstein's theory of special relativity, continues to challenge our intuitive understanding of space-time and has far-reaching implications for physics and our perception of the universe. This thought experiment, involving twins who age differently due to relativistic effects, serves as a gateway to exploring the fundamental nature of time and space.

    At its core, the twin paradox demonstrates that time is not absolute but relative, depending on an observer's frame of reference. This concept has profound implications for our understanding of the universe. It suggests that time can flow at different rates for different observers, challenging our everyday experience of time as a constant, universal phenomenon. This relativistic effect becomes more pronounced at speeds approaching that of light, opening up intriguing possibilities for space travel and our understanding of cosmic events.

    The implications of the twin paradox extend beyond theoretical physics into practical applications and thought experiments. For instance, it plays a crucial role in the precise functioning of GPS systems, where satellites must account for time dilation effects to maintain accuracy. In the realm of space exploration, the paradox raises questions about the feasibility of long-distance space travel and its impact on astronauts' aging processes relative to Earth-bound observers.

    Furthermore, the twin paradox serves as a springboard for exploring other relativistic effects and their implications. It leads to discussions about the nature of simultaneity, the malleability of space-time, and the interconnectedness of space and time as a four-dimensional continuum. These concepts challenge our classical notions of reality and open up new avenues for understanding the fabric of the universe.

    Thought experiments related to the twin paradox often involve extreme scenarios that push the boundaries of our understanding. For example, considering the effects of traveling near a black hole, where gravitational time dilation becomes significant, or imagining civilizations existing at different relativistic frames, leading to complex temporal relationships between them. These scenarios, while often beyond our current technological capabilities, provide valuable insights into the nature of space-time and inspire further scientific inquiry.

    The twin paradox also intersects with other areas of physics, such as quantum mechanics, potentially offering insights into the reconciliation of these two fundamental theories. It raises questions about the nature of time at the quantum level and how relativistic effects might influence quantum phenomena. This intersection of relativistic and quantum effects remains an active area of research, promising to deepen our understanding of the universe's most fundamental laws.

    Real-World Applications and Experiments

    Time dilation and the twin paradox, once considered purely theoretical concepts, have found significant real-world applications and experimental validation in modern science and technology. These phenomena, predicted by Einstein's theory of relativity, have profound implications for our understanding of space, time, and the universe at large. In this section, we'll explore some of the most compelling practical applications and experiments that demonstrate these principles in action.

    One of the most ubiquitous and practical applications of time dilation can be found in GPS satellites. These orbital devices, which provide precise location data for countless everyday activities, must account for the effects of both special and general relativity to maintain accuracy. GPS satellites experience time dilation due to their high orbital speeds (special relativity) and their position in Earth's gravitational field (general relativity). Without correcting for these effects, GPS systems would accumulate errors of up to 10 kilometers per day, rendering them useless for navigation. Engineers must program the satellites to adjust their clocks to run slightly slower than Earth-based clocks to compensate for these relativistic effects, ensuring the system's precision.

    Particle accelerators, such as the Large Hadron Collider (LHC) at CERN, provide another striking example of time dilation in action. These massive scientific instruments accelerate subatomic particles to speeds approaching that of light. At these extreme velocities, the particles experience significant time dilation. For instance, muons, which typically have a lifetime of about 2.2 microseconds when at rest, can travel much farther than expected due to time dilation. This effect allows scientists to study these short-lived particles in detail, providing crucial insights into the fundamental laws of physics.

    Experimental evidence for time dilation has also been gathered through precise atomic clock experiments. In 1971, physicists Joseph Hafele and Richard Keating conducted a famous experiment where they flew atomic clocks around the world on commercial airliners. They found that the airborne clocks experienced a slight time dilation effect compared to stationary clocks on Earth, confirming Einstein's predictions. More recent experiments using even more precise atomic clocks have further validated these findings, measuring time dilation effects with unprecedented accuracy.

    The principles of time dilation also have practical implications for space travel and exploration. Future long-distance space missions will need to account for these effects, as astronauts traveling at high speeds or in different gravitational fields will experience time differently than those on Earth. This could impact communication, navigation, and even the aging process of space travelers.

    In the realm of astrophysics, time dilation plays a crucial role in our observations of the universe. The light from distant galaxies and cosmic phenomena is subject to gravitational time dilation as it passes through the warped spacetime near massive objects. This effect must be considered when interpreting data from deep space observations, influencing our understanding of cosmic distances, ages, and the evolution of the universe.

    The twin paradox, while more challenging to demonstrate experimentally on a human scale, has been indirectly validated through various high-precision experiments. While we can't send one twin on a relativistic space journey, the principles underlying the paradox are consistently confirmed in particle physics experiments and astronomical observations.

    As technology advances, our ability to measure and utilize the effects of time dilation continues to improve. From enhancing the accuracy of global navigation systems to pushing the boundaries of particle physics research, these relativistic phenomena have transitioned from theoretical curiosities to essential considerations in cutting-edge science and technology. The practical applications of time dilation not only validate Einstein's groundbreaking theories but also open up new avenues for exploration and innovation in fields ranging from telecommunications to space exploration.

    Conclusion

    In summary, time dilation and the twin paradox are fascinating concepts in special relativity that challenge our everyday understanding of time. The introduction video serves as an excellent starting point for grasping these complex ideas. Time dilation shows that time passes differently for objects moving at different speeds or in different gravitational fields. The twin paradox, a thought experiment involving one twin traveling at high speed while the other remains on Earth, illustrates the counterintuitive effects of time dilation. These concepts have profound implications for our understanding of the universe and have been experimentally verified. The video's clear explanations and visual aids make these abstract ideas more accessible. To deepen your understanding of special relativity, we encourage you to explore further resources on the subject. Delve into the mathematics behind these phenomena, study real-world applications, and consider the philosophical implications of time being relative. The world of special relativity offers endless opportunities for intellectual exploration and discovery.

    Twin Paradox

    The twin paradox is a thought experiment in the special theory of relativity that involves identical twins. One twin travels into space in a high-speed rocket and returns home to find that the twin who stayed on Earth has aged more. This paradox is a fascinating consequence of time dilation, a key concept in Einstein's theory of relativity.

    Step 1: Understanding Time Dilation

    Time dilation is a phenomenon predicted by the special theory of relativity. It states that time passes at different rates for observers in different frames of reference. Specifically, time passes more slowly for an observer in motion relative to a stationary observer. This effect becomes more pronounced as the speed of the moving observer approaches the speed of light.

    In the context of the twin paradox, time dilation means that the twin traveling in the spaceship (the moving observer) will experience time more slowly compared to the twin who remains on Earth (the stationary observer). This difference in the passage of time is the key to understanding the twin paradox.

    Step 2: Setting Up the Twin Paradox

    To illustrate the twin paradox, imagine a pair of 20-year-old identical twins. One twin boards a spaceship and travels to a distant star at a significant fraction of the speed of light, while the other twin stays on Earth. The traveling twin spends a certain amount of time in space before returning to Earth.

    According to the theory of relativity, the traveling twin will experience less time passing than the twin who remains on Earth. This is because the spaceship is moving at a high velocity, causing time to dilate for the traveling twin. As a result, when the traveling twin returns to Earth, they will be younger than the twin who stayed behind.

    Step 3: Calculating the Time Difference

    To quantify the time difference between the twins, we use the time dilation formula:

    Δt = Δt / (1 - v²/c²)

    Where:

    • Δt is the time interval experienced by the stationary observer (the twin on Earth).
    • Δt is the time interval experienced by the moving observer (the traveling twin).
    • v is the velocity of the moving observer.
    • c is the speed of light.

    In the twin paradox scenario, if 20 years pass for the twin on Earth, the traveling twin might experience significantly less time, depending on the speed of the spaceship. For example, if the spaceship travels at a speed close to the speed of light, the traveling twin might only age by one year during the journey.

    Step 4: Resolving the Paradox

    The twin paradox is resolved by understanding that the situation is not symmetrical. The traveling twin undergoes acceleration and deceleration during the journey, which breaks the symmetry of the situation. The twin on Earth remains in an inertial frame of reference, while the traveling twin does not.

    When the traveling twin returns to Earth, they will find that more time has passed for the twin who stayed behind. This difference in aging is a direct consequence of time dilation and the fact that the traveling twin experienced a different frame of reference during the journey.

    Step 5: Implications of the Twin Paradox

    The twin paradox has profound implications for our understanding of time and space. It demonstrates that time is not absolute and can vary depending on the relative motion of observers. This thought experiment also highlights the importance of considering the effects of acceleration and deceleration in relativistic scenarios.

    The twin paradox is not just a theoretical curiosity; it has practical implications for space travel and our understanding of the universe. As we explore the cosmos and develop technologies that allow for high-speed travel, the effects of time dilation will become increasingly relevant.

    FAQs

    Here are some frequently asked questions about time dilation and the twin paradox:

    1. What is time dilation?

      Time dilation is a phenomenon predicted by Einstein's theory of special relativity where time passes at different rates for objects moving at different speeds or in different gravitational fields. As an object's speed approaches the speed of light, time slows down for that object relative to a stationary observer.

    2. How does the twin paradox work?

      The twin paradox is a thought experiment where one twin stays on Earth while the other travels at high speed through space. Due to time dilation, the traveling twin ages more slowly. Upon return, the space-traveling twin is younger than the Earth-bound twin, despite both having experienced the same duration from their own perspectives.

    3. Has time dilation been proven experimentally?

      Yes, time dilation has been confirmed through various experiments. The Hafele-Keating experiment in 1971 used atomic clocks on airplanes to measure time differences. More recently, ultra-precise atomic clocks have demonstrated time dilation effects even at much smaller speed differences and altitude changes on Earth.

    4. How does time dilation affect GPS systems?

      GPS satellites experience time dilation due to their high orbital speeds and position in Earth's gravitational field. Without accounting for these effects, GPS systems would accumulate errors of up to 10 kilometers per day. Engineers program the satellites to adjust their clocks to compensate for these relativistic effects, ensuring accurate positioning.

    5. Can time dilation lead to time travel?

      While time dilation doesn't allow for traditional "time travel" as depicted in science fiction, it does result in different rates of time passage. An object moving at very high speeds or in strong gravitational fields will experience less time passage than a stationary object. This effect is more like time slowing down rather than traveling backwards or forwards in time.

    Prerequisite Topics

    Understanding the postulate of the special theory of relativity, time dilation, and the twin paradox requires a solid foundation in various physics and mathematics concepts. While there are no specific prerequisite topics provided for this subject, it's crucial to recognize that a strong background in classical physics, basic relativity, and mathematical principles is essential for grasping these advanced concepts.

    To fully comprehend the postulate of the special theory of relativity, students should be familiar with Newtonian mechanics and the principles of classical physics. This foundation helps in understanding how Einstein's theory revolutionized our perception of space and time. Additionally, a good grasp of vector algebra and basic calculus is crucial for working with the mathematical formulations of special relativity.

    Time dilation, a key concept in special relativity, builds upon the understanding of reference frames and relative motion. Students should be comfortable with these ideas to appreciate how time can appear to pass differently for observers in different reference frames. Furthermore, knowledge of light propagation and the speed of light as a universal constant is fundamental to grasping the core principles of special relativity.

    The twin paradox, an intriguing thought experiment in special relativity, requires a solid understanding of time dilation and length contraction. Students should be familiar with these effects to follow the logical progression of the paradox and its resolution. Additionally, concepts from general relativity, such as the equivalence principle, can provide a deeper insight into the twin paradox scenario.

    While not explicitly listed as prerequisites, topics like Lorentz transformations and spacetime diagrams are invaluable tools for visualizing and calculating relativistic effects. Familiarity with these concepts can significantly enhance a student's ability to tackle problems related to special relativity and the twin paradox.

    In conclusion, although specific prerequisites are not provided, a strong foundation in classical physics, mathematics, and basic concepts of relativity is crucial for mastering the postulate of special relativity, time dilation, and the twin paradox. Students are encouraged to review these fundamental topics to ensure a comprehensive understanding of these advanced concepts in modern physics.

    In this lesson, we will learn:

    • Galilean-Newtonian relativity
    • Inertial frame of references Vs. Non-inertial frame of references
    • Relativity principles
    • Comparison between variables among different frame of references
    • The Michelson-Morley experiment
    • Postulates of the Special Theory of Relativity
    • Time Dilation and Twin Paradox.

    Notes:

    Galilean-Newtonian relativity

    This theory deals with observing objects and events from different frame of references.
    The special relativity deals with the “inertial frame of reference” in which the Newton’s first law of motion is valid. It is easier to measure and observe events using the inertial frame of reference.

    Inertial frame of references Vs. Non-inertial frame of references

    Although Earth is rotating, for most purposes we take the earth as inertial frame of reference
    A frame of reference moving at constant speed with respect to an inertial frame is also considered as an inertial frame of reference.
    Any rotating or accelerating frame is considered as a non-inertial frame of reference.

    Relativity principles

    The laws of physics are the same in all inertial frame of reference;
    Examples: while travelling in a train, which moves with constant velocity, bodies move just as just they do when you are at rest on the Earth.

    Relativity principles

    The laws of physics are the same in all inertial frame of reference;
    Examples: while travelling in a train, which moves with constant velocity, bodies move just as just they do when you are at rest on the Earth.

    Dropping a coin inside the car and taking two different frame of references;

    1. Frame of reference: car
      The coin falls straight downward and hit the floor directly below the point of release.

    2. Frame of reference: Earth
      From an observer point of view on the Erath, the coin covers a curved path.

      3. Conclusion;
      The actual path covered by the coin is different as viewed from different frames of references.
      This does not contradict the laws of physics, the same law of gravity and motion applies in both reference frames. (same acceleration in both frame of references)

      The only difference;
      In the first frame of reference the initial velocity of the coin is zero but in the second frame of reference the coin has same initial velocity as the car.

    In classical mechanics, space and time intervals are considered to be absolute;
    In any frame of reference, the TIME INTERVAL, LENGTH, is the same. Their measurement does not change form one reference frame to another.

    Mass of the object and all forces are assumed to be the same in all frame of references.

    Comparison between variables among different frame of references

     \, Variable  \,

    Length

    Time

    Position

    Velocity

    Mass

    Force

    Acceleration

    Stays the same

    ✓ \checkmark

    ✓ \checkmark

    ✓ \checkmark

    ✓ \checkmark

    ✓ \checkmark

    Changes

    ✓ \checkmark

    ✓ \checkmark



    Example
    A person walks inside a train toward the front with a speed of 4m/s. but if the train moves 20m/s with respect to the Earth, then the person is moving with 24m/s with respect to the Earth. The acceleration, however, is the same for both frame of references.

    Frame of reference; train
    The person accelerates from 0 to 4m/s in 2 seconds

    α=v2−v1t=4−02= \alpha = \frac{v_{2} - v_{1}} {t} = \frac{4 - 0} {2} = 2mm/s2


    Frame of reference; the Earth
    The person accelerates from 20 to 24 in 2 seconds

    α=v2−v1t=24−202= \alpha = \frac{v_{2} - v_{1}} {t} = \frac{24 - 20} {2} = 2mm/s2

    According to Newton’s second law;

    F=maF= ma
    Acceleration is constant
    Mass is constant

    Therefore, force does not change as the frame of reference changes.

    In general, we can say, all inertial frames are equivalent, laws of mechanics are the same in any initial frame of reference.

    The “ether”

    Waves can travel trough medium, such as; water, air, rope.
    The medium though which light waves can travel is called the “ether”.
    A transparent medium with zero density through which light can travel.
    Maxwell’s predicted that light is an electromagnetic wave, using his equation the velocity of light calculated to be 3.0×108m/s, but is equation has no provision for relative velocity. (no specific frame of reference is defined by Maxwell) Scientists assume that the Maxwell’s equitation must be with respect to the ether.

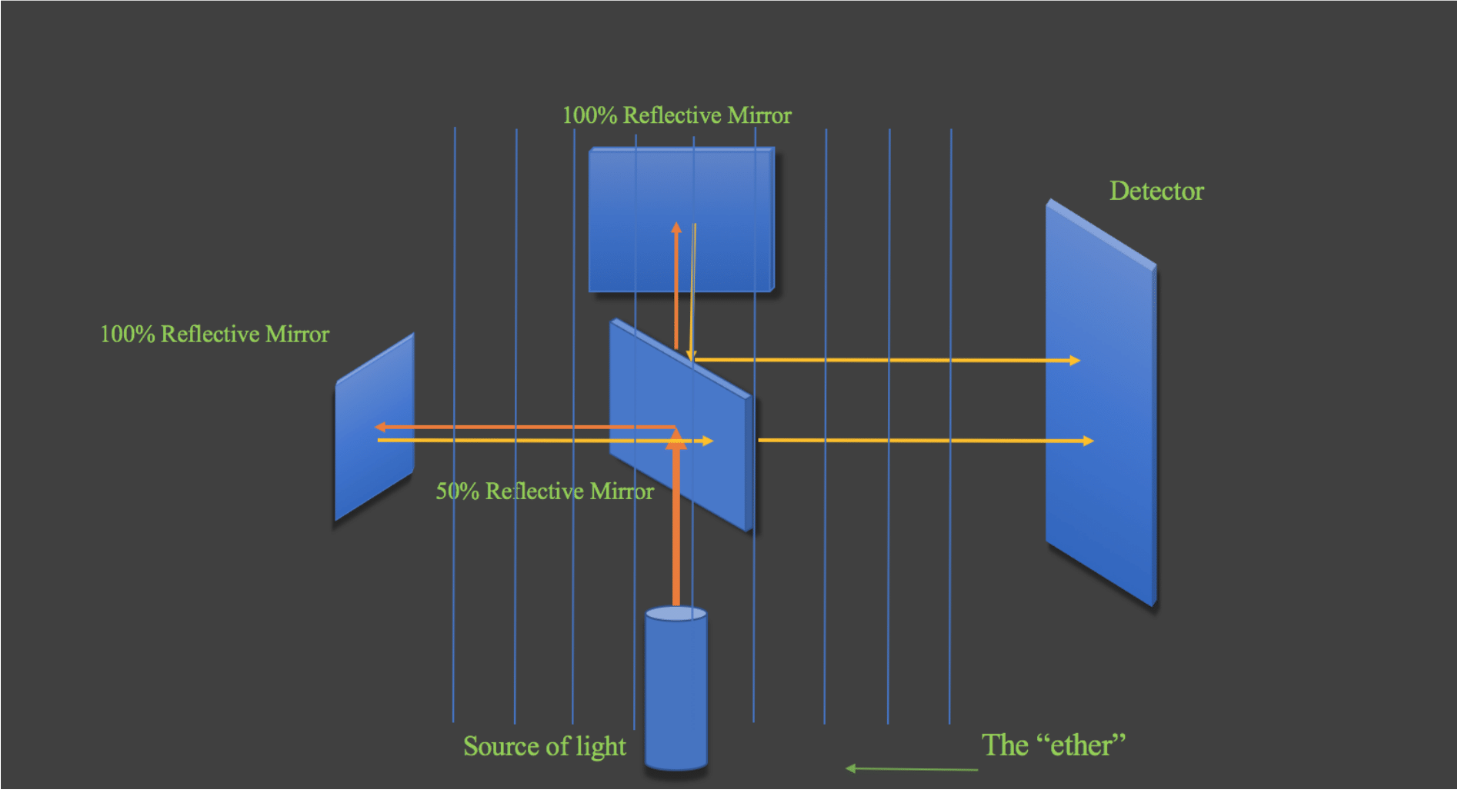
    The Michelson-Morley experiment

    The Michelson-Morley experiment was done to check the existence of the ether.
    The difference in the speed of light in different directions is measured.
    They were expecting to find different values for the speed of light as the orientation of the apparatus was changing. The existence of the ether should affect the velocity of the light in different directions.
    Null result: no difference was detected

    Postulate of Special Theory of Relativity Time Dilation and Twin Paradox


    Postulates of the Special Theory of Relativity

    The postulates proposed by Einstein is to come up with a solution to the “ether” existence.

    The first postulate
    The laws of physics (not only the laws of mechanics) have the same form in all inertial reference frames.

    The second postulate
    Light propagates through empty space with a definite speed c independent of the speed of the source or observer.
    (The speed of light predicted by Maxwell’s equation, is the speed of light in vacuum in any frame of reference)

    Note: The special theory of relativity, deals only with inertial frames.

    Time Dilation and Twin Paradox

    To calculate the time Dilation, lets calculate the time taken for light to travel across a spaceship and back from two different observers;

    1. Observer on the spaceship

      2. Postulate of Special Theory of Relativity Time Dilation and Twin Paradox

      △t0=2Dc \triangle t_{0} = \frac{2D} {c}

    2. Observer on the Earth

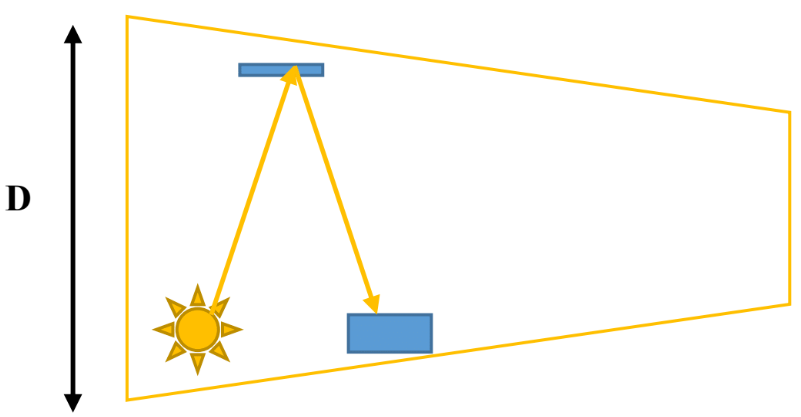
      3. Postulate of Special Theory of Relativity Time Dilation and Twin Paradox


      2L=v.△t ⇒ L = v.△t/L = v. \triangle t \, \Rightarrow \, L \, = \, v. \triangle t/2

    vv is the speed of the spaceship

    the time interval, △\trianglet, observed by the observer on the Earth can be calculated as follows;

    The light ravels the total distance on its diagonal path (Pythagoras theorem), therefore,
    distance travelled by light is  d=  \,d = \,2 D2+L2\, \large \sqrt{D^{2} + L^{2}}
    distance travelled by light can be calculated using;

    d=cd = c. △t\triangle t (distance travelled by light)

    but,  d=  \,d = \,2 D2+L2\,\large \sqrt{D^{2} + L^{2}}

    ⇒ \Rightarrow \enspace 2 D2+L2=c.△t \, \large \sqrt{D^{2} + L^{2}} = c.\triangle t \, where L=v.△t/L = v.\triangle t/2  ⇒ \, \Rightarrow \, 2 D2+(v.△t/2)2=c.△t\, \large \sqrt{D^{2} + (v.\triangle t/2})^{2} = c.\triangle t

    We square both sides,

    4[D2+(v.△t/2)2]=c2△t2 ⇒ 4[D2+(v2.△t2)/4]=c2△t2 ⇒ 4[D^{2} + (v.\triangle t/2)^{2}] = c^{2}\triangle t^{2} \, \Rightarrow \, 4[D^{2} + (v^{2.}\triangle t^{2})/4] = c^{2}\triangle t^{2} \, \Rightarrow

    4D2+(v2.△t2)=c2△t2 ⇒ 4D2=c2△t2−(v2.△t2) ⇒ 4D2=△t2(c2−v2) ⇒4D^{2} + (v^{2.}\triangle t^{2}) = c^{2}\triangle t^{2} \, \Rightarrow \, 4D^{2} = c^{2}\triangle t^{2} - (v^{2.}\triangle t^{2}) \, \Rightarrow \, 4D^{2} = \triangle t^{2}(c^{2} - v^{2}) \ \Rightarrow

    △t2=4D2/(c2−v2)⇒△t=2D/c2−v2 ⇒ \triangle t^{2} = 4D^{2}/(c^{2} - v^{2}) \quad \Rightarrow \quad \triangle t = 2D / \sqrt{c^{2} - v^{2} } \, \Rightarrow

    △t=2D/c2(1−v2/c2)⇒△t=\triangle t = 2D / \sqrt{c^{2}(1 - v^{2} / c^{2}) } \quad \Rightarrow \quad \triangle t = 2Dc(1−v2/c2)\large \frac{2D} {{^c}\sqrt{(1 - v^{2} / c^{2})}}

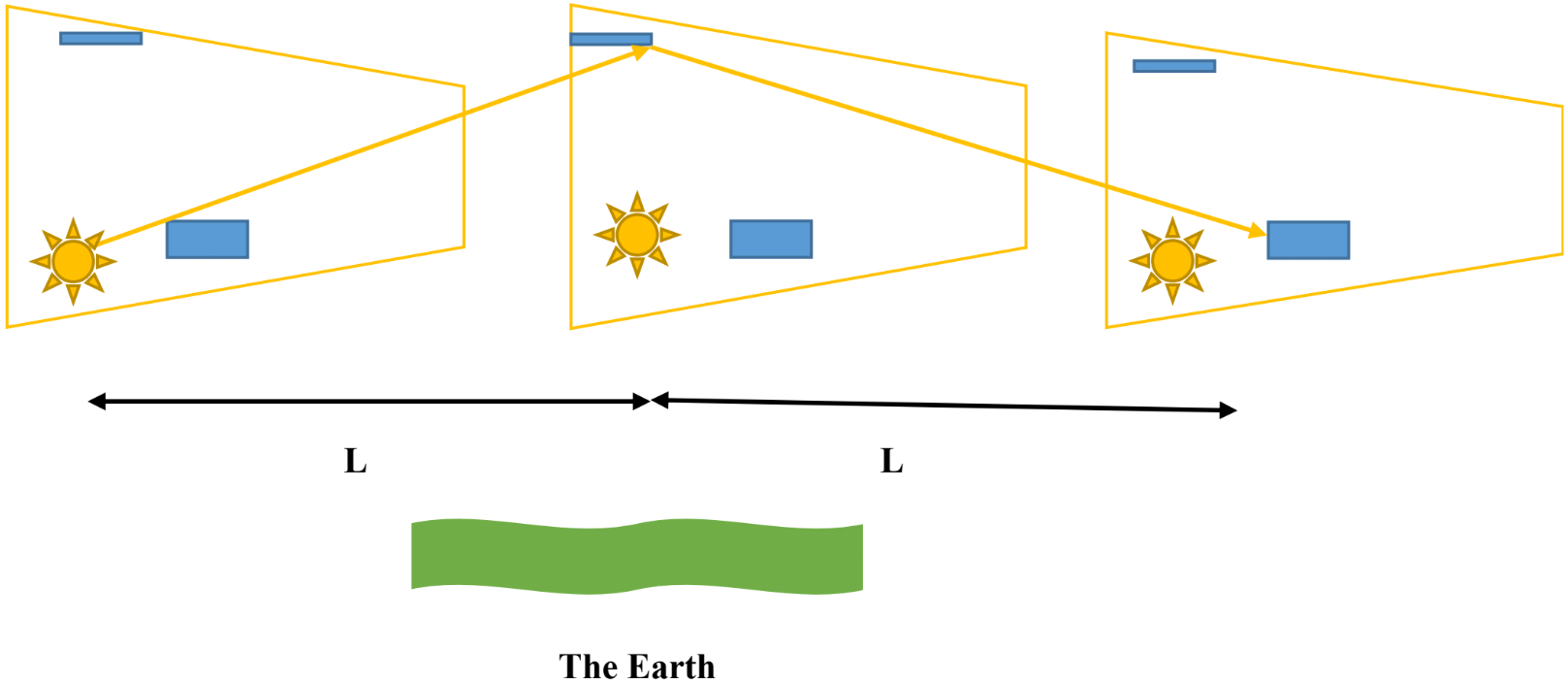
    In the reference frame of the spaceship, the time interval measured by observer on spaceship is calculated as △t0=2Dc,2D=c.△t0\triangle t_{0} = \frac{2D}{c} , 2D = c. \triangle t_{0}

    Substituting 2D in △t\triangle t equation we can find △t\triangle t in terms of cc and vv.

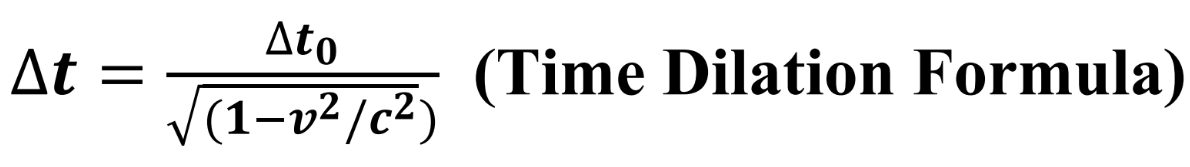
    Postulate of Special Theory of Relativity Time Dilation and Twin Paradox

    △t\triangle t: the time interval observed by the observer on Earth
    t0t_{0}: the time interval observed by the observer on Earth
    vv: the speed of the spaceship
    vv: the speed of light

    Note:
    Always (v2(v^{2} < c2) ⇒ △t0 (1−v2/c2)  c^{2}) \, \Rightarrow \, \triangle t_{0} \, \sqrt{(1 - v^{2} / c^{2})} \, is always more than one  ⇒ △t \, \Rightarrow \, \triangle t >  △t0 \, \triangle t_{0}

    Time Dilation equation can also be written as; △t=γ△t0 \triangle t = \gamma \triangle t_{0} \, where, γ=1/(1−v2/c2) \gamma = 1/ \sqrt{(1 - v^{2} / c^{2})}

    The fact that two events simultaneous to one observer may not be simultaneous to a second observer, suggest that time is not absolute.

    Twin paradox

    According to the twin paradox, if a pair of 20-year-old twins are separated, one takes off to the space with a spaceship travelling at very high speed, and the other one stays on the Earth. The astronaut tween will age lese. Whereas 20 years might pass for the Earth twin, the astronaut, perhaps only one year would pass for the traveler. When the traveler returns, the earthbound twin would be 40 years old whereas the traveling twin would be only 21.

    As the speed of a moving object gets closer to the speed of the light, the slower the time travels.