Sign In
Try for Free
 flag-us

Redox Titrations: Mastering Analytical Chemistry Techniques
Dive into the world of redox titrations and unlock the power of precise chemical analysis. Learn to quantify oxidizing and reducing agents, and apply this crucial skill to real-world scenarios in various scientific fields.

Get the most by viewing this topic in your current grade. Pick your course now.

Now Playing:Redox titrations – Example 0a
Intros
  1. Using titration for redox reactions.
  2. Using titration for redox reactions.
    Recap of titration.
  3. Using titration for redox reactions.
    Titration for redox reactions.
Examples
  1. Find the full equation and concentration of substances in a redox titration.
    A solution containing Co2+ ions of unknown concentration is made. 25mL of this Co2+ solution was measured and was titrated by 0.25M MnO4- solution until equivalence point was reached. An average titre of 16.20 mL MnO4- solution was required. The reaction produces Mn2+ and Co3+ ions.

    Write the full redox equation for this reaction and find the concentration of the aqueous Co2+ solution.
    Redox titrations
    Jump to:NotesConceptFAQsPrerequisites
    Notes

    In this lesson, we will learn:

    • To recall the practical use of titration experiments
    • How titration applies to redox reactions.
    • How to calculate chemical quantities required in redox reactions.
    • How to use the Winkler titration method to find the biochemical oxygen demand (BOD).

    Notes:

    • We learned the basics of a titration with its use in acid-base chemistry in Acid-base-titration.
      Just like acid-base titrations are used to find the concentration of acids and bases, a redox titration can be done to find the unknown concentration of a chemical in a redox process. The working out and calculations are detailed in Acid-base-titration and is summarized in the image below. Chemical A and chemical B in a redox titration would simply be the two chemicals in the redox (the reducing and oxidizing agent):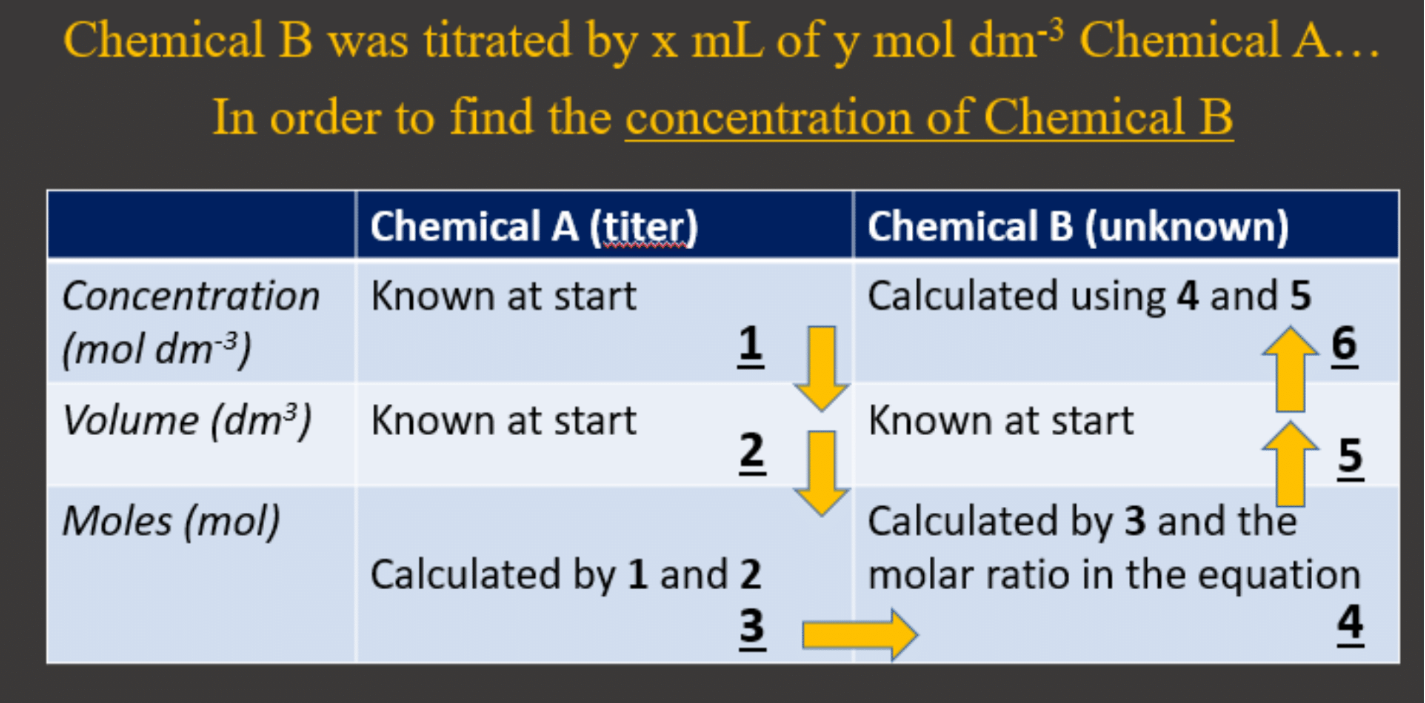

      Redox titrations


    • Redox titrations will involve a reducing and oxidizing agent reacting together, but indicator is normally not used like it is in acid-base titrations. This means that one of the reactants used has to be one with a color difference between its reduced and oxidized form. There are two good options:
      • Potassium permanganate (KMnO4) is an oxidizing agent that is purple in solution, but turns colorless when reduced to Mn2+ ions.
      • Potassium iodide (KI) in solution gives I- ions that get oxidized (lots of chemicals can be used for this part) into brown-colored I2 in solution. Then in a redox titration, I2 can be reduced back to colorless I- ions. Starch can be added (it acts like an indicator for I2) to this, which is blue-black when I2 is present, the color fading when I2 becomes I- again.


    • WORKED EXAMPLE:
      A solution containing Co2+ ions of unknown concentration is made. 25mL of this Co2+ solution was measured and was titrated by 0.2M MnO4- solution until equivalence point was reached. 19.40 mL of the MnO4- solution was required.
      The first thing that needs doing is the finding out of the two half-reactions:
      • Manganese in MnO4- will be reduced to Mn2+ ions as shown in the half-equation:

        MnO4- + 8H+ + 5e-\enspace → \enspace Mn2+ + 4H2O

      • Co2+ ions can be oxidized to Co3+ according to the half-equation:

        Co2+\enspace → \enspace Co3+ + e-

        The method for working out half-equations in redox was covered in Half equations.

      Next, the combining of the two half-reactions will give us the overall equation

      1 x [ MnO4- + 8H+ + 5e-\enspace → \enspace Mn2+ + 4H2O ]

      5 x [ Co2+\enspace → \enspace Co3+ + e- ]

      These balance for electrons and give the overall equation:

      MnO4- + 8H+ + 5Co2+\enspace → \enspace Mn2+ + 4H2O + 5Co3+

      This reaction has the cobalt solution as the unknown, so MnO4- with known concentration is being added by burette. MnO4- is purple and as it is added to the cobalt solution, the purple color will disappear as Co2+ reacts it away. When equivalence point is reached the purple color will no longer be removed as there will be no more Co2+ to remove the MnO4- and the purple color that it causes. Therefore the equivalence point is shown by the appearance of the purple color of the MnO4- thats now in excess.

      The number of moles of MnO4- can be calculated using the information in the question:

      Mol MnO4- = 19.40 mL * 1  L1000  mL  ∗  0.2  mol  MnO4−1  L\frac{1\; L}{1000 \; m L} \;* \; \frac{0.2\; mol \; MnO_{4}^{-}}{1 \; L} = 3.88 * 10-3 mol MnO4-

      Looking at the equation, we can see a 1:5 MnO4- to Co2+ ratio. The equivalence point will have five times as many moles of cobalt as manganese, then.

      Mol Co2+ = 3.88 * 10-3 MnO4-  \,* 5  mol  Co2+1  mol  MnO4−\frac{5\; mol \; Co^{2+}}{1 \; mol \; MnO_{4}^{-}} = 0.0194 mol Co2+

      With the moles of Co2+ ions now found in 25 mL volume of the sample used, we can calculate the concentration.

      [Co2+] = 0.0194  mol  Co2+0.025  L\frac{0.0194\; mol \; Co^{2+}}{0.025 \; L } = 0.776 M Co2+

    • The Winkler method (or Winkler titration) is a way of finding the concentration of oxygen in water systems using a redox titration reaction. This is important for knowing the biochemical oxygen demand (BOD) of a water system, which is a good indicator of water purity. A lot of decaying matter like dead organisms and sewage in the water will cause an upsurge in bacteria, which demand oxygen in their respiratory processes.
      The Winkler method makes oxygen the limiting reagent in a redox titration process.
      A common redox reaction to do this with is the titration of iodine by thiosulfate ions. The limited supply of oxygen initially oxidises iodide to produce the iodine:

      • Mn2+ (aq) + O2 (aq) + 4 OH- (aq)  \,→  \, 2 MnO2 (aq) + 2 H2O (l)

      MnO2 (aq) + 4 H+ (aq) + 2 I- (aq)  \,→  \, Mn2+ (aq) + I2 + 2 H2O (l)

      The iodine then reacts with the thiosulfate:

      I2 (aq) + 2 S2O32- (aq)  \,→  \, S4O62- (aq) + 2 I- (aq)

      The amount of iodine that reacts in the final step is entirely dependent on how much oxygen was available to turn it from iodide into iodine. This is what the Winkler method is trying to find out.

      The concentration of oxygen is an indicator of how much is available and not being used up by microorganisms in the water. At any temperature, there is a general solubility of oxygen in water.
      With a typical atmosphere of 21% O2 gas, O2 solubility at 298K and 100 kPa (room temperature and pressure) is around 8.2*10-3 g dm-3. Any gap between this value and oxygen concentration in your sample indicates the biochemical oxygen demand (BOD).

    • Worked example: Using the Winkler method of titration to find BOD.

      • A 750 mL sample of water from a lake is saturated with oxygen and left for a week. Afterward, the titration of iodine by thiosulfate ions is run, with the iodine being prepared in solution by reacting with oxygen in the following complete reaction scheme:

      Mn2+ (aq) + O2 (aq) + 4 OH- (aq)  \,→  \, 2 MnO2 (aq) + 2 H2O (l)

      MnO2 (aq) + 4 H+(aq) + 2 I- (aq)  \,→  \, Mn2+ (aq) + I2 + 2 H2O (l)

      I2 (aq) + 2 S2O32- (aq)  \,→  \, S4O62- (aq) + 2 I- (aq)

      5.7 mL of a 0.05 M thiosulfate (S2O32-) solution completely reacted the I2 present in the water sample.
      Calculate how many moles of O2 were present in the water. Assuming 8.2*10-3 g dm-3 solubility of oxygen in this water, determine the BOD of this water sample.

      This question needs to be answered like a regular titration question first. We need to know how much oxygen is there. We can get this information by the amounts of thiosulfate and the molar ratios involved.
      • There is a 1:2 ratio of iodine to thiosulfate, so


        • mol S2O32- = 0.05 M S2O32- * 5.7  mL  S2O32−1000 \large \frac{5.7\; mL\; S_{2}O_{3}^{2-}}{1000} =2.85 * 10-4


        mol I2 = 2.85  ×  10−42 \large \frac{2.85 \; \times \; 10^{-4}}{2} = 1.425 × 10-4

      • There is a 1:1 ratio with iodine and MnO2, which itself is in a 2:1 ratio with O2. So we need to cut this amount in half again:


        • mol O2 = 1.425  ×  10−42 \large \frac{1.425\; \times \; 10^{-4}}{2} = 7.125 × 10-5


        You could say overall there is a 4 : 1 thiosulfate : O2 ratio in this reaction sequence, so cutting the thiosulfate moles by 4 will give us moles of O2.

      • This second step needs us to find quantities in grams per decimeter cube. We are currently in moles, so we need to convert to grams and divide by volume in dm3 (where 1 dm3 = 1 L)


        • g O2 = 7.125 × 10-5 mol × 32gmol \frac{g}{mol} =2.28 × 10-3g O2

        This is the amount found in a 750 mL sample. This is 3/4 of a litre (or dm3), so this value needs to be divided by 0.75 to find the value for 1 dm-3.

        2.28  ×  10−3 g  O20.75  dm3\large \frac{ 2.28 \; \times \; 10^{-3} \, g \; O_{2}} {0.75\; dm^{3}} = 3.04 × 10-3 g dm-3

        This concentration is the amount of oxygen still available in the water sample. Assuming from above that a saturated sample at room temperature and pressure has a solubility of 8.2 × 10-3 g dm-3, the gap between our concentration and this concentration is the biochemical oxygen demand (BOD):

        BOD=8.2 × 10-3 - 3.04 × 10-3 = 5.16 × 10-3 g dm-3

        This is the concentration of oxygen being used up by (micro)organisms in the water system, assuming the level is at equilibrium now.
    Concept

    Introduction to Redox Titrations

    Redox titrations are a fundamental analytical technique in chemistry, essential for determining the concentration of oxidizing or reducing agents in a solution. Our introduction video provides a comprehensive overview of this crucial concept, serving as an excellent starting point for students and researchers alike. Similar to acid-base titrations, redox titrations involve the gradual addition of one solution to another until the reaction reaches completion. However, instead of neutralizing acids and bases, redox titrations focus on the transfer of electrons between oxidizing and reducing agents. This process allows for precise quantification of these agents in various samples. Understanding redox titrations is vital for applications in environmental analysis, industrial quality control, and pharmaceutical research. By mastering this technique, chemists can accurately measure the concentration of important substances like vitamin C, chlorine in water, or iron in ore samples. The principles of redox titrations form the foundation for more advanced electrochemical methods and are indispensable in modern analytical chemistry.

    FAQs

    1. What is meant by redox titration?

      Redox titration is an analytical technique used to determine the concentration of an oxidizing or reducing agent in a solution. It involves the transfer of electrons between the analyte and a standardized titrant, resulting in a change in oxidation states. The endpoint is typically detected through a color change or using electrochemical methods.

    2. What is the difference between a redox titration and an acid-base titration?

      The main difference lies in the type of reaction occurring. Redox titrations involve electron transfer and changes in oxidation states, while acid-base titrations involve proton transfer. Redox titrations can analyze a wider range of substances and often use different indicators or endpoint detection methods compared to acid-base titrations.

    3. Why is acid needed in redox titration?

      Acid is often added in redox titrations to create favorable conditions for the reaction. It can help prevent side reactions, adjust the pH to an optimal range for the redox reaction, and in some cases, it's necessary for the formation of reactive species. For example, in permanganate titrations, acid is required to form the reactive Mn2+ species.

    4. What is being oxidized in a redox titration?

      In a redox titration, either the analyte or the titrant is being oxidized, while the other is being reduced. The species being oxidized loses electrons and increases its oxidation state. For example, in the titration of Fe2+ with KMnO4, the Fe2+ is being oxidized to Fe3+, while the MnO4- is being reduced to Mn2+.

    5. How to solve redox titrations?

      To solve redox titrations, follow these steps: 1) Balance the redox equation, 2) Determine the mole ratio between the analyte and titrant, 3) Calculate the moles of titrant used, 4) Use the mole ratio to find the moles of analyte, and 5) Calculate the concentration or mass of the analyte. Always consider the stoichiometry of the reaction and any dilutions made during the process.

    Prerequisites

    Understanding the foundation of redox titrations is crucial for mastering this important analytical technique in chemistry. One of the key prerequisite topics that students should grasp is calculating cell potential (voltaic cells). This fundamental concept plays a vital role in comprehending the principles behind redox titrations and their applications in various chemical analyses.

    Redox titrations are a type of volumetric analysis used to determine the concentration of an analyte in a solution by utilizing oxidation-reduction reactions. To fully appreciate the intricacies of redox titrations, it's essential to have a solid understanding of cell potentials and how they relate to the spontaneity of redox reactions. The ability to calculate and interpret cell potentials provides valuable insights into the direction and extent of electron transfer in redox reactions, which is the cornerstone of redox titrations.

    When performing redox titrations, students often encounter color changes that indicate the endpoint of the reaction. These color changes are directly related to the redox processes occurring in the solution. By understanding cell potentials and predicting redox reactions, students can better interpret these visual cues and accurately determine the endpoint of the titration.

    Moreover, the concept of cell potentials is crucial for selecting appropriate indicators in redox titrations. Many indicators used in these titrations are themselves redox-active species, and their color changes are dependent on the oxidation state of the indicator molecules. A thorough understanding of cell potentials helps in choosing the right indicator for a specific redox titration, ensuring accurate and reliable results.

    In addition, knowledge of cell potentials aids in understanding the limitations and potential sources of error in redox titrations. For instance, competing side reactions or incomplete reactions can affect the accuracy of the titration. By applying their understanding of cell potentials, students can predict these potential issues and take appropriate measures to minimize their impact on the analysis.

    Furthermore, the ability to calculate and interpret cell potentials is invaluable when working with more complex redox systems, such as those involving multiple redox couples or in non-standard conditions. This knowledge allows students to adapt their titration techniques and calculations to a wide range of analytical scenarios, making them more versatile and proficient in chemical analysis.

    In conclusion, a solid grasp of calculating cell potentials and predicting redox reactions is indispensable for students approaching the study of redox titrations. This prerequisite knowledge not only enhances their understanding of the underlying principles but also improves their practical skills in performing and interpreting redox titrations accurately. By mastering these fundamental concepts, students will be well-equipped to tackle more advanced topics in analytical chemistry and electrochemistry.