Set builder notation - Set Theory

Do better in math today
Get Started Now

Set Theory Topics:

Set builder notation

Lessons

Notes:

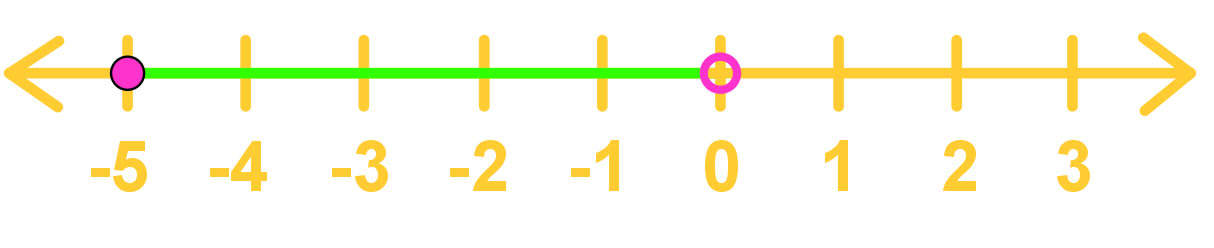
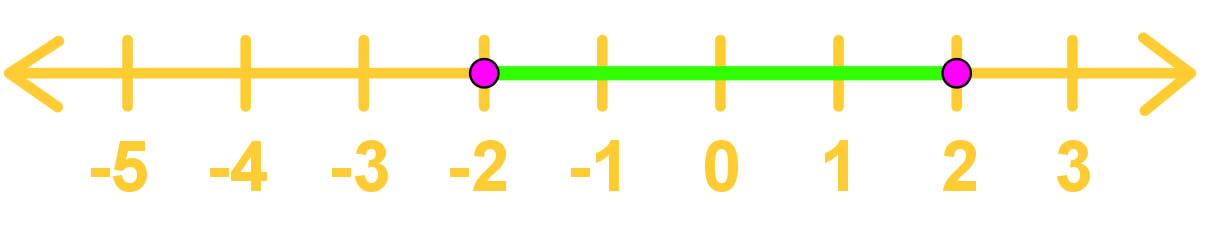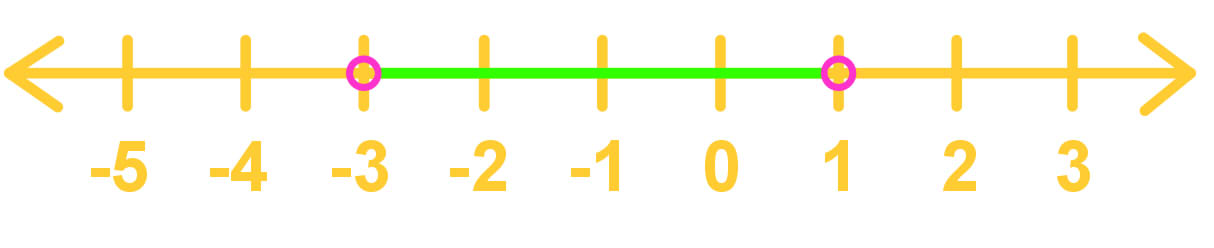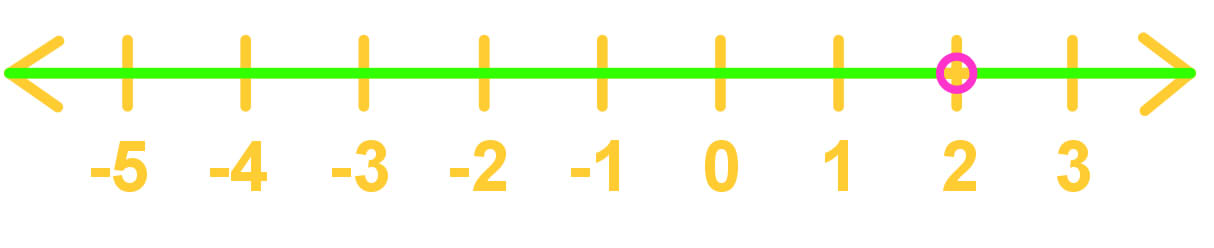A set is a collection of elements (usually numbers)

E.g. {x∈R∣xx \in R | x > 0} should be read as “the set of all x’s that are an element of the real numbers such that x is greater than 0.”

Special symbols:

- RR = real numbers

- ZZ = integers

- NN = natural numbers

- QQ = rational numbers

- CC = complex numbers

- II = imaginary numbers

  • 1.
    Translating Intervals On Number Lines Into Set Builder Notation Form

    Translate the following intervals into set builder notation form.

  • 2.
    Evaluating the Domains of Expressions in Set Builder Notation Form

    What are the domains for the following expressions? Write the answers in set builder notation form.

Teacher pug

Set builder notation

Don't just watch, practice makes perfect.

We have over 1850 practice questions in Algebra for you to master.
Get Started Now