Sign In
Sign Up
flag-us

Reflection across the x-axis: y = -f(x)

Get the most by viewing this topic in your current grade. Pick your course now.

Now Playing:Reflection across the x axis– Example 1
Intros
  1. An Experiment to Study "Reflection Across the X-axis"
    Sketch and compare: y=(x−4)3y = {\left( {x - 4} \right)^3}
    VS.
    −y=(x−4)3 - y = {\left( {x - 4} \right)^3}
  2. An Experiment to Study "Reflection Across the X-axis"
    Sketch and compare: y=(x−4)3y = {\left( {x - 4} \right)^3}
    VS.
    −y=(x−4)3 - y = {\left( {x - 4} \right)^3}
    Sketch both quadratic functions on the same set of coordinate axes.
  3. An Experiment to Study "Reflection Across the X-axis"
    Sketch and compare: y=(x−4)3y = {\left( {x - 4} \right)^3}
    VS.
    −y=(x−4)3 - y = {\left( {x - 4} \right)^3}
    Compared to the graph of y=(x−4)3y = {\left( {x - 4} \right)^3}:
    • the graph of −y=(x−4)3 - y = {\left( {x - 4} \right)^3} is a reflection in the ___________________.
Examples
  1. Reflection Across the X-axis
    Given the graph of y=f(x)y = f\left( x \right) as shown, sketch:
    1. y=−f(x)y = - f\left( x \right)

    2. In conclusion:
      • (y)→(−y)\left( y \right) \to \left( { - y} \right): reflection in the ____________________ ⇒\Rightarrow all yy coordinates ______________________________.
      Reflections of the x-axis

Relationship between two variables
Jump to:NotesPrerequisitesRelated
Notes
The concept behind the reflections about the x-axis is basically the same as the reflections about the y-axis. The only difference is that, rather than the y-axis, the points are reflected from above the x-axis to below the x-axis, and vice versa.

Reflection Across the X-Axis

Before we get into reflections across the y-axis, make sure you've refreshed your memory on how to do simple vertical and horizontal translations.

How to Reflect Over X-Axis:

One of the most basic transformations you can make with simple functions is to reflect it across the x-axis or another horizontal axis. In a potential test question, this can be phrased in many different ways, so make sure you recognize the following terms as just another way of saying "perform a reflection across the x-axis":

1) Graph y=−f(x)y = -f(x)

2) Graph −f(x)-f(x)

3) Reflect over xx axis

In order to do this, the process is extremely simple: For any function, no matter how complicated it is, simply pick out easy-to-determine coordinates, divide the y-coordinate by (-1), and then re-plot those coordinates. That's it!

The best way to practice drawing reflections across the y-axis is to do an example problem:

Example:

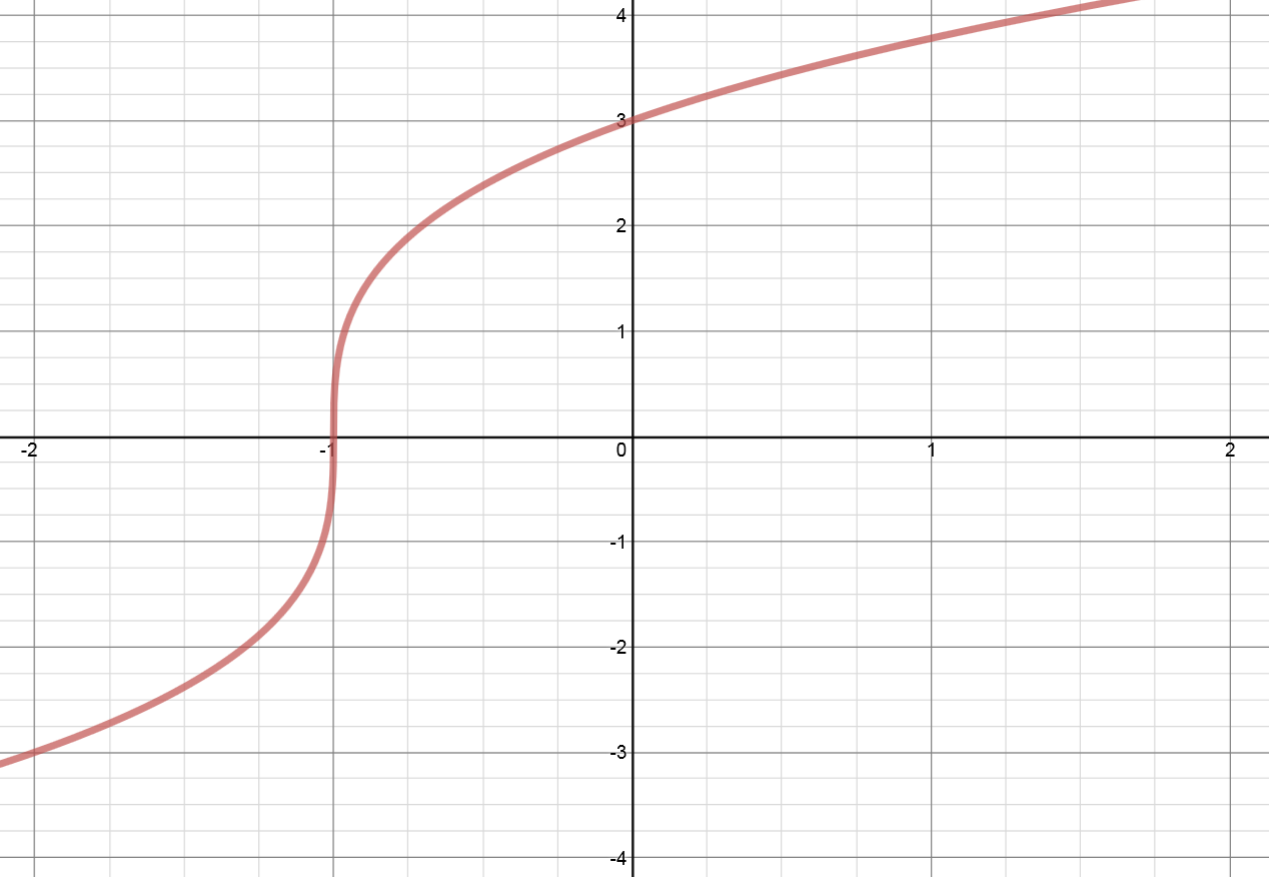
Given the graph of y=f(x)y = f(x) as shown, sketch y=−f(x)y = -f(x).

Remember, the only step we have to do before plotting the −f(x)-f(x) reflection is simply divide the y-coordinates of easy-to-determine points on our graph above by (-1). When we say "easy-to-determine points" what this refers to is just points for which you know the x and y values exactly. Don't pick points where you need to estimate values, as this makes the problem unnecessarily hard. Below are several images to help you visualize how to solve this problem.

Step 1: Know that we're reflecting across the x-axis

Since we were asked to plot the –f(x)f(x) reflection, is it very important that you recognize this means we are being asked to plot the reflection over the x-axis. When drawing reflections across the xx and yy axis, it is very easy to get confused by some of the notations. So, make sure you take a moment before solving any reflection problem to confirm you know what you're being asked to do.

Step 2: Identify easy-to-determine points

Remember, pick some points (3 is usually enough) that are easy to pick out, meaning you know exactly what the x and y values are. In this case, let's pick (-2 ,-3), (-1 ,0), and (0,3).

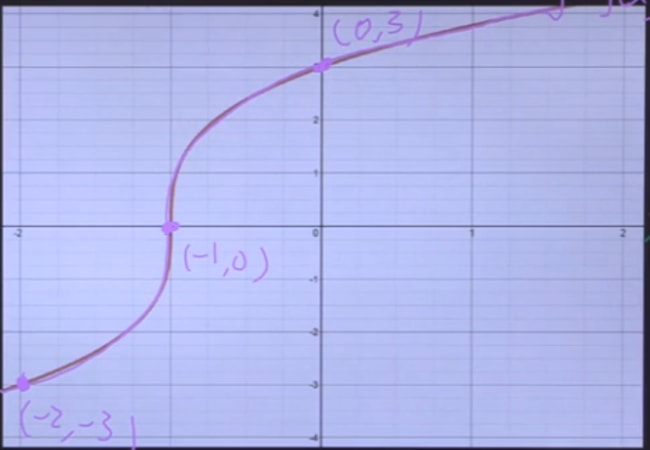
Pick three points with x and y value and graph
Pick three points and graph

Step 3: Divide these points by (-1)

While the xx values remain the same, all we need to do is divide the yy values by (-1)!

Divide y values by -1 while x values stay the same
Divide y values by -1

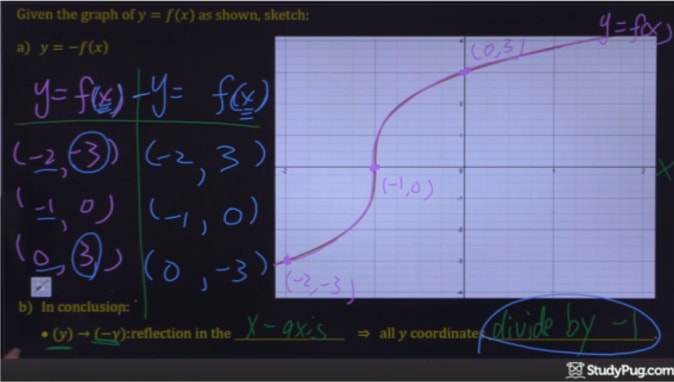
Step 4: Plot the new points

Plot the new points using the values after dividing y by -1
Plot new points after dividing y values by -1

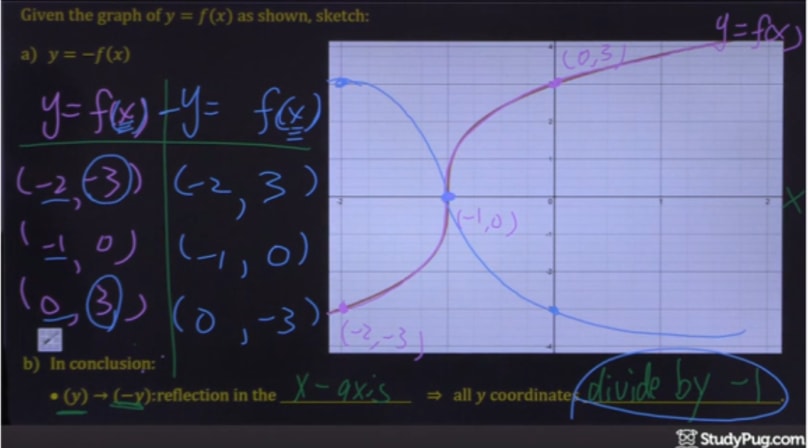
And that's it! Simple, right?

What is the Axis of Symmetry:

In some cases, you will be asked to perform horizontal reflections across an axis of symmetry that isn't the x-axis. But before we go into how to solve this, it's important to know what we mean by "axis of symmetry". The axis of symmetry is simply the horizontal line that we are performing the reflection across. It can be the x-axis, or any horizontal line with the equation yy = constant, like yy = 2, yy = -16, etc.

How to Find the Axis of Symmetry:

Finding the axis of symmetry, like plotting the reflections themselves, is also a simple process. In this case, all we have to do is pick the same point on both the function and its reflection, count the distance between them, divide that by 2, and count that distance away from one of the graphs. This is because, by it's definition, an axis of symmetry is exactly in the middle of the function and its reflection.

The best way to practice finding the axis of symmetry is to do an example problem.

Example:

Find the axis of symmetry for the two functions shown in the images below.

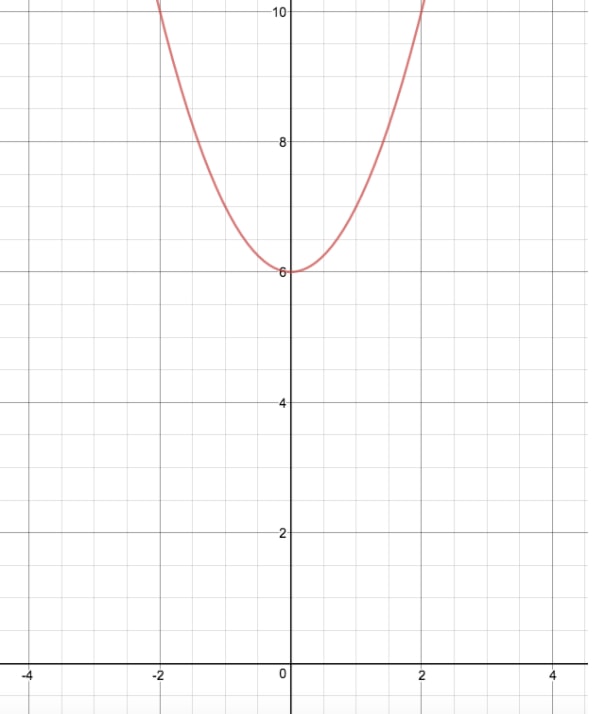
Graph A to reflect through x axis
Graph A to reflect through x axis
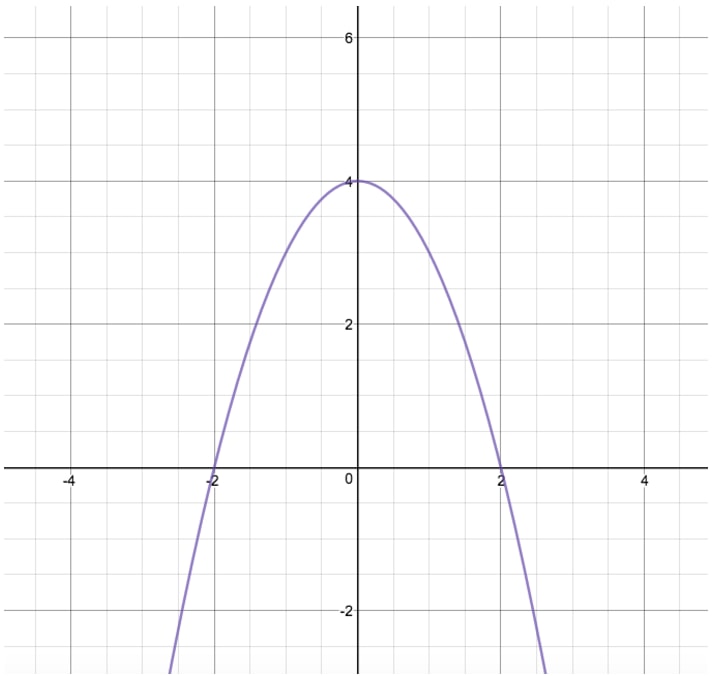
Graph B to reflect through x axis
Graph B to reflect through x axis

Again, all we need to do to solve this problem is to pick the same point on both functions, count the distance between them, divide by 2, and then add that distance to one of our functions. Let's pick the origin point for these functions, as it is the easiest point to deal with.

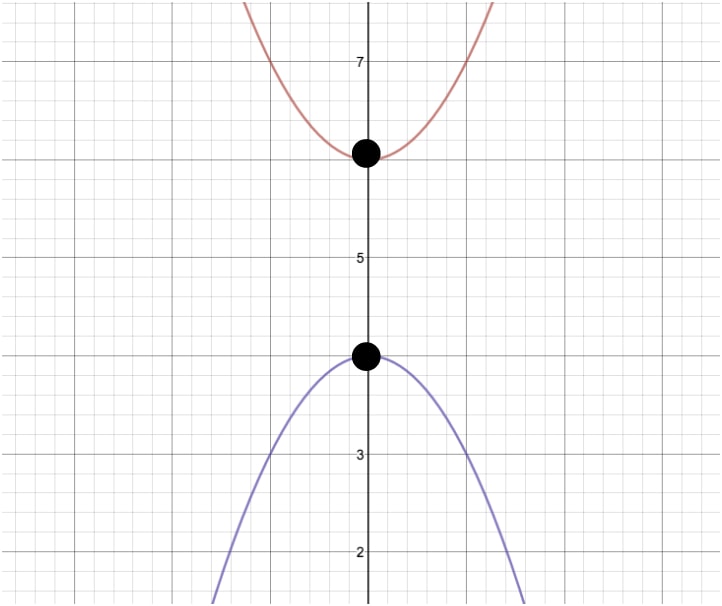
The distance of the same point of two functions
Distance of the same point in two functions

Now, by counting the distance between these two points, you should get the answer of 2 units. The last step is to divide this value by 2, giving us 1. Now we know that our axis of symmetry is exactly one unit below the top function's origin or above the bottom functions origin. Looking at the graph, this gives us yy = 5 as our axis of symmetry! Let's take a look at what this would look like if there were an actual line there:

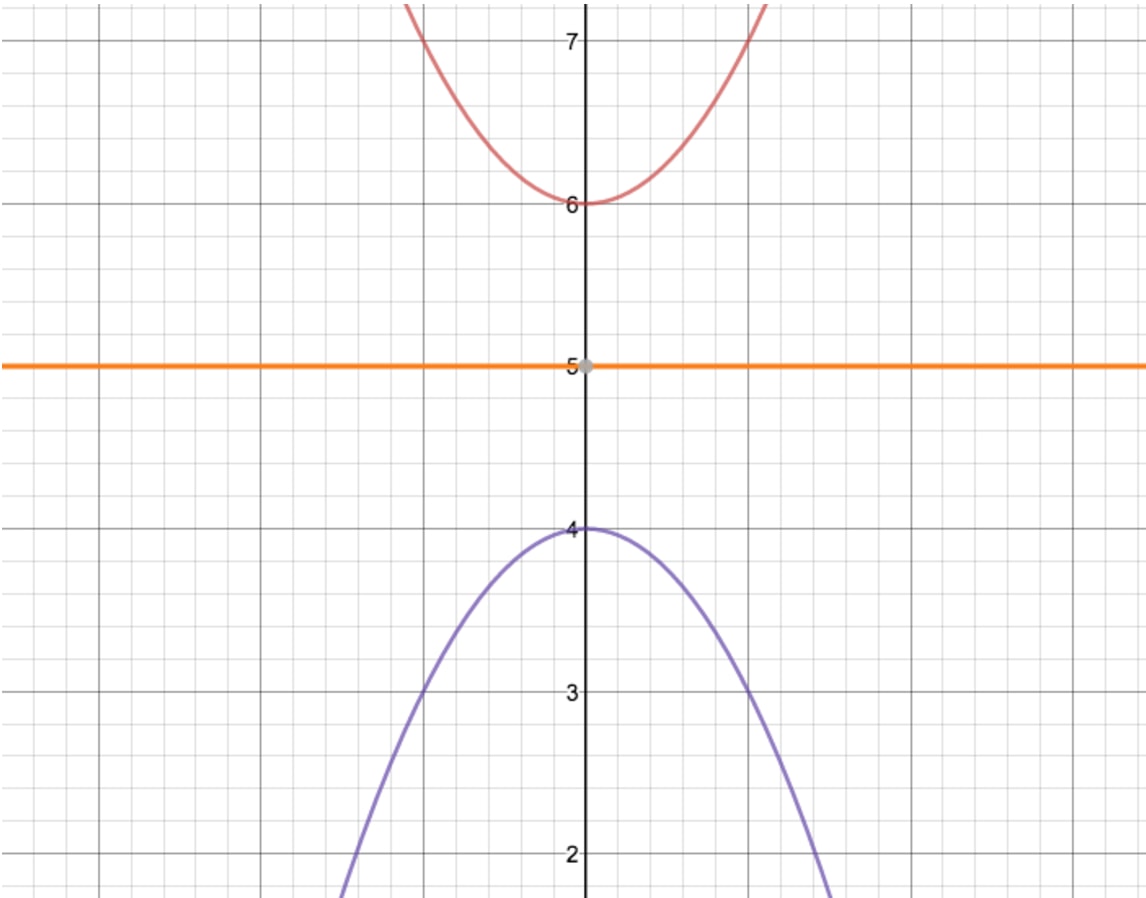
Imaginary line between two graphs for reflection through x axis
Imaginary line for reflection through x axis

And that's all there is to it! You may learn further on how to graph transformations of trigonometric functions and how to determine trigonometric functions from their graphs in other sections.