In this lesson, we will learn:
- Identifying Indirect Variation Equations
- Identifying Indirect Variation Graphs
- Evaluating Indirect Variations
- Word Problem: Application of Indirect Variations



In this lesson, we will learn:
Welcome to our exploration of inverse variation, a fascinating concept that contrasts sharply with direct variation. While you might be familiar with direct relationships where variables increase together, inverse variation flips this idea on its head. In inverse variation, as one quantity increases, the other decreases proportionally. This unique relationship is crucial in many real-world applications, from physics to economics. To kick off our learning journey, I've prepared an introduction video that will visually demonstrate these concepts. This video is a key resource in grasping the fundamental principles of inverse variation. It'll help you see how inverse relationships work in practice and why they're so important. As we dive deeper into this topic, you'll discover how inverse variation differs from direct variation and how to recognize it in various scenarios. Remember, understanding inverse variation is a stepping stone to mastering more complex mathematical relationships. So, let's get started and unravel the intricacies of this essential mathematical concept together!
Identifying Indirect Variation Equations
Classify the following variations. (Direct or Indirect).
b=−8a
In this step, we need to understand the given equation and the type of variation it represents. The equation provided is b=−8a. Our task is to determine whether this equation represents a direct or indirect (inverse) variation.
To classify the variation, we convert the equation into a fraction form. This helps us identify the relationship between the variables. For the given equation b=−8a, we can write it as:
1b=1−8a
Here, both b and a are in the numerator.
Next, we need to determine if the variables are directly or inversely proportional. In an inverse variation, one variable is in the numerator and the other is in the denominator. In a direct variation, both variables are on the same side, typically in the numerator.
Since both b and a are in the numerator, this indicates a direct variation.
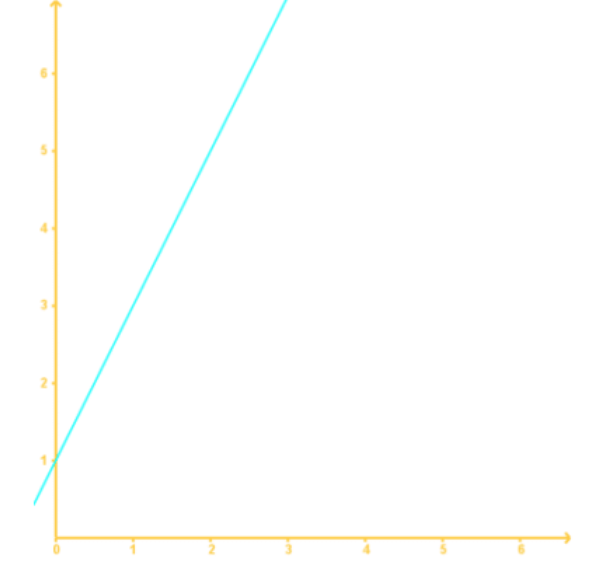
To further confirm, we can use the direct variation formula, which is y=kx. In our equation, b corresponds to y, a corresponds to x, and −8 is the constant of variation k. Thus, the equation b=−8a fits the form of a direct variation.
Based on the steps above, we have determined that the equation b=−8a represents a direct variation. Both variables are in the numerator, and the equation fits the direct variation formula y=kx.
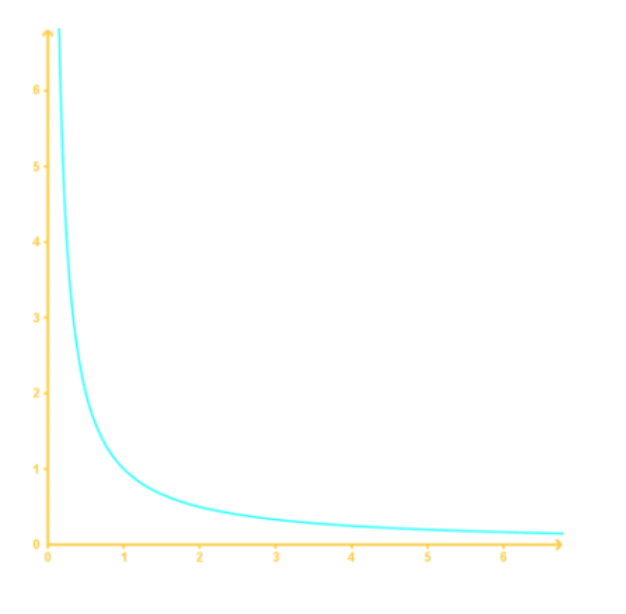
Inverse variation is a mathematical relationship between two variables where as one increases, the other decreases proportionally, so that their product remains constant. It's expressed as y = k/x, where k is the constant of variation.
In inverse variation, variables move in opposite directions while maintaining a constant product. In direct variation, variables increase or decrease together, maintaining a constant ratio. The formula for direct variation is y = kx, while for inverse variation it's y = k/x.
The constant of variation, denoted as 'k', is the product of the two variables in an inverse variation relationship. It remains constant throughout the relationship and is crucial for solving inverse variation problems.
A common real-life example is the relationship between speed and time when traveling a fixed distance. As speed increases, the time taken decreases proportionally, maintaining a constant product (distance).
The graph of inverse variation is a hyperbola. It's plotted by selecting a range of x-values, calculating corresponding y-values using y = k/x, and plotting these points. The resulting curve approaches but never touches the x and y axes, which are its asymptotes.
Before diving into the concept of inverse variation, it's crucial to have a solid foundation in several key mathematical areas. Understanding these prerequisite topics will significantly enhance your ability to grasp and apply the principles of inverse variation effectively.
One of the most important concepts to master is direct variation. This fundamental relationship between variables serves as a counterpoint to inverse variation, and understanding how quantities can increase or decrease in proportion to each other lays the groundwork for comprehending more complex relationships. Direct variation provides a basis for comparing and contrasting with inverse variation, allowing students to recognize the unique characteristics of each.
Another critical skill is understanding tables of values of linear relationships. This ability to interpret and analyze data presented in tabular form is essential when working with inverse variation problems. By becoming proficient in reading and creating these tables, students can more easily identify patterns and relationships between variables, which is crucial when dealing with inverse variations.
Additionally, familiarity with distance and time related questions in linear equations provides practical context for inverse variation. Many real-world applications of inverse variation involve relationships between distance, time, and speed. Having a strong foundation in solving these types of problems enhances your ability to apply inverse variation concepts to practical scenarios.
By mastering these prerequisite topics, students build a robust framework for understanding inverse variation. Direct variation serves as a comparative concept, helping to highlight the unique properties of inverse relationships. Proficiency in interpreting tables of values enables students to recognize and analyze the patterns characteristic of inverse variation. Finally, experience with distance and time problems provides valuable context for applying inverse variation to real-world situations.
As you progress in your study of inverse variation, you'll find that these prerequisite skills continually come into play. They form the building blocks upon which more advanced concepts are constructed. For instance, when graphing inverse variations, your understanding of linear relationships will prove invaluable. Similarly, when solving complex word problems involving inverse variation, your experience with distance and time questions will give you a significant advantage.
In conclusion, taking the time to thoroughly understand these prerequisite topics is not just beneficialit's essential for mastering inverse variation. Each concept builds upon the others, creating a comprehensive understanding that will serve you well in your mathematical journey. By solidifying your knowledge in these areas, you'll be well-prepared to tackle the intricacies of inverse variation and its wide-ranging applications in mathematics and the real world.