Transformers and Power Transmission: Efficient Energy Transfer

Intros

Start Watching

Lessons

Introduction to transformers
Transformers equations
Power transmission

Examples

Start Watching

Lessons

An ideal transformer with 120V, ac supply, on the primary coil supplies power to the resistor R. If this resistor dissipates 35W, what is the current in the primary coil and the secondary coil?
The secondary coil in an ideal transformer has 5 times as many windings as the primary. If the current in the primary is 0.40A, determine the current in the secondary, and the type of the transformer.
A certain step-down transformer has a 500-turns primary that operates at 120V ac supply. Which one of the following sets of conditions could describe the secondary turns and voltages of this transformer?
A step-down transformer is required to operate a 12 V, 25 W halogen lamp. Which of the following sets of conditions could apply to this transformer?
1. $N_{p} = 20, \qquad N_{s} = 200$
2. $V_{p} = 120V, \qquad I_{s} = 0.21 A$
3. $I_{p} = 2.1 A, \qquad I_{s} = 2.1 A$
4. $V_{p} = 120V, \qquad I_{p} = 0.21 A$
An ideal transformer has a potential difference of 130V ac across the primary windings and a potential difference of 780V ac across the secondary windings. There are 390 turns in the secondary. The secondary current is
1. twice the primary current
2. one half the primary current
3. six times the primary current
4. one-sixth the primary current

Topic Notes

In this lesson, we will learn:

What does a transformer do and what does it consist of?
Types of transformers
Transformers equations
How to reduce power loss in transmission lines?

Notes:

Transformers

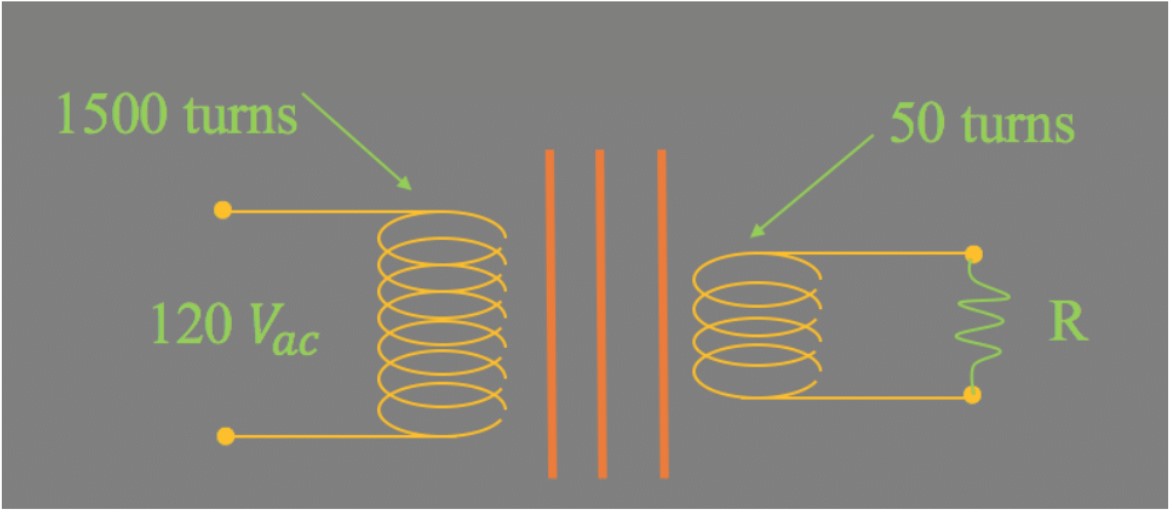
A device for increasing or decreasing an ac voltage.
Example of transformers:
1. There is a transformer in TV sets to provide high voltage for the picture tube.
2. Utility poles require transformers to reduce the high voltage from the electric company to a useable voltage in houses.

Transformers operate only with ac voltages; dc voltage does not produce any change in flux to induce an emf.

A transformer consists of two coils, the primary coil, and a secondary coil.

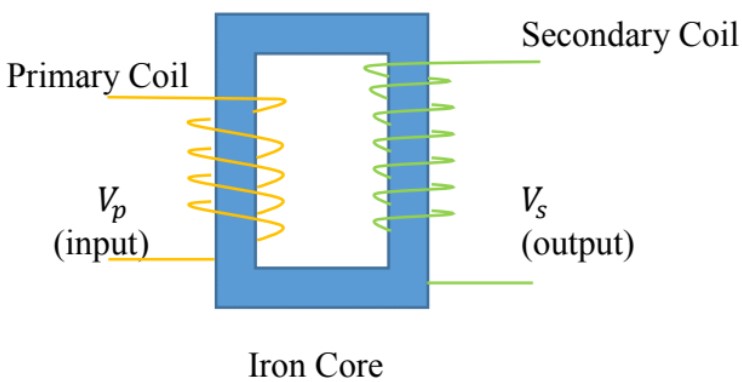
The two coils are interconnected with an iron core.
Nearly all magnetic flux produced in the primary coil is transferred to the secondary coil.
As the primary coil is connected to an ac voltage, the change in the magnetic field induced a current, which is then transferred to the secondary coil with the same frequency.
Due to the number of coils, the voltage would be different in the secondary coil compared to that of the primary.

Transformers Equations

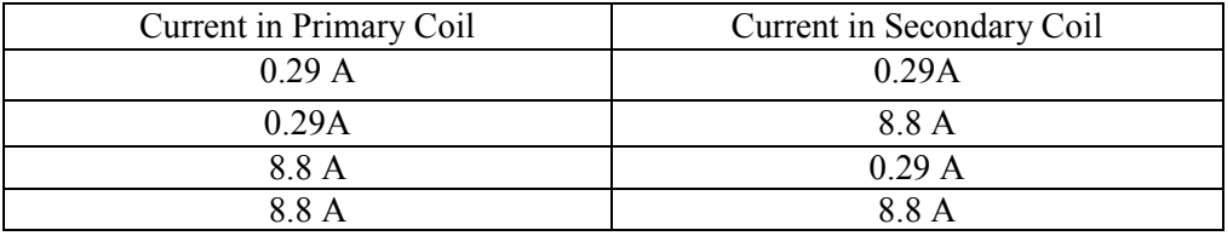
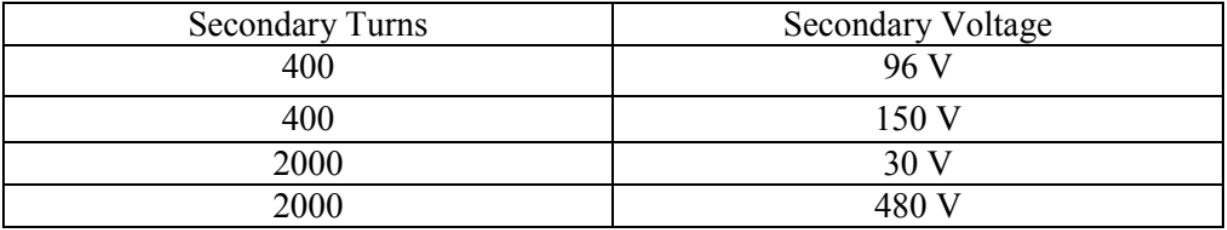
According to Faraday’s law, the voltage induced in the secondary coil can be calculated using the following equation:

V_{s} = N_{s}

\large \frac{\Delta \phi B} {\Delta t}

N_{s} =

number of turns in the secondary coil

\large \frac{\Delta \phi B} {\Delta t} =

the rate of change in flux

The input voltage would be the result of the change in flux in the primary coil, therefore;

V_{p} = N_{p}

\large \frac{\Delta \phi B} {\Delta t}

N_{p} =

number of turns in the primary coil
Assuming no or little amount of flux is lost, we divide the above two equations;

\large \frac{V_{s} } {V_{p} } = \frac{N_{s} \frac{\Delta \phi B} {\Delta t} } { N_{p} \frac{\Delta \phi B} {\Delta t} }

\, \Rightarrow \,

\large \frac{V_{s}} {V_{p}} = \frac{N_{s}} {N_{p}} \,

(transformer equation I )

The above equation shows that the voltage in the secondary coil is related to the primary coil.

Types of Transformers

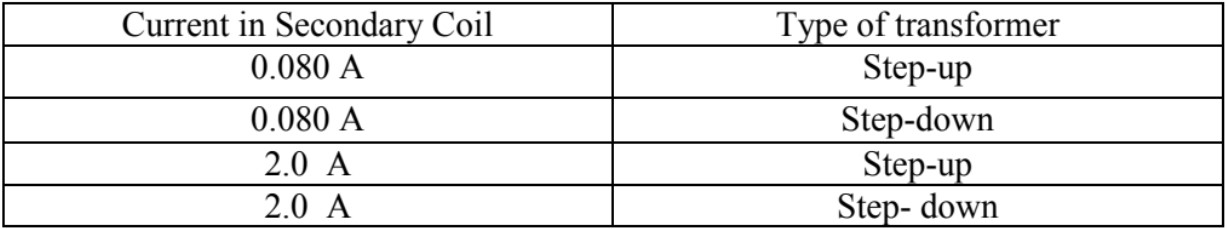
There are two types of transformer:

Step-up transformer
If the number of turns in the secondary coil is more than the primary coil; the voltage in the secondary coil would be higher than that of the primary.

$N_{s}$ > $N_{p} \, \Rightarrow \quad V_{s}$ > $V_{p}$

Step- down transformer
If the number of turns in the primary coil is more than the secondary coil; the voltage in the primary coil would be higher than that of the secondary.

$N_{p}$ > $N_{s} \, \Rightarrow \quad V_{p}$ > $V_{s}$

Note: the output power is equal to the input power in ideal transforms where their efficiency is 99%.

P = IV

P_{s} = I_{s} V_{s}

P_{s} = P_{p} \, \Rightarrow \quad I_{s} V_{s} = I_{p}V_{p} \quad \Rightarrow \quad

\large \frac{I_{s}} {I_{p}} = \frac{V_{p}} {V_{s}} = \frac{N_{p}} {N_{s}}

P_{p} = I_{p} V_{p}

\large \frac{I_{s}} {I_{p}} = \frac{N_{p}} {N_{s}} \;

(transformers equation II)

Transmission Lines

Transformers are very important electric devices in transmitting electricity from the power plant to houses.
Since the power has to be transmitted over long distances, part of the energy will be lost in the transmission lines.
To minimize the loss in energy, the power is transmitted at high voltage.
This is done by

Using step-up transformers to increase the voltage, as the power leaves the power plant station.
Using step-down transformers to decrease the voltage before the power enters the houses.

Example of Transmission Lines

An average of 140 KW of electric power is sent from a power plant, 20 km away, to a small town. The total resistance of the transmission lines is 0.60

\Omega

. calculate the power loss if the power is transmitted at:

240V
12000 V

Solution

To find the power loss we should apply the equation $P = I^{2} R$ ,
The equation $\, P = \frac{V^{2}} {R} \,$ does not work in this case, since we do not have the voltage drop across the lines.

Step 1
calculate the current:

$\large I = \frac{P} {V} = \frac{1.4 \, \times \, 10^{5}} {2.4 \, \times 10^{2}} =$ 583.3 $A$

Step 2
Find the power loss:

$P = I^{2}R = (583.3)^{2} \, (0.60)$ = 204143.33 $W$ = 204 $KW$

Almost 60% of all overpower is lost in the power lines!!!!!

If the transmission voltage increases to 12000 $V$ ,

$\large I = \frac{P} {V} = \frac{1.4 \, \times \, 10^{5}} {1.2 \, \times 10^{4}} =$ 11.6 $\, A \, \simeq$ 12 $A$

$\quad P = I^{2} R =$ (12)²(0.60) = 86.4 $W$

the power loss decreases to 0.6%

Transformers and power transmission

Intros

Lessons

Examples

Lessons

Free to Join!

Easily See Your Progress

Make Use of Our Learning Aids

Last Viewed

Practice Accuracy

Suggested Tasks

Earn Achievements as You Learn

Create and Customize Your Avatar

Topic Notes

Introduction to Transformers and Power Transmission

Understanding Transformers: Function and Components

Types of Transformers: Step-Up and Step-Down

Transformer Equations and Principles

Power Transmission and Transformers

Efficiency and Challenges in Power Transmission

Conclusion: The Future of Transformers in Power Systems

Introduction to Transformers

Step 1: Understanding the Purpose of Transformers

Step 2: AC Voltage and Transformers

Step 3: Components of a Transformer

Step 4: Working Principle of Transformers

Step 5: Reducing Power Loss in Transmission Lines

FAQs

What is the transmission of power?

What is a transformer and how does it work?

What are the 3 types of transformers?

How do transformers work in the power grid?

How do transformers reduce transmission loss?

Prerequisite Topics

Transformers and power transmission

Lessons

Lessons

Easily See Your Progress

Make Use of Our Learning Aids

Last Viewed

Practice Accuracy

Suggested Tasks

Earn Achievements as You Learn

Create and Customize Your Avatar

Introduction to Transformers and Power Transmission

Understanding Transformers: Function and Components

Types of Transformers: Step-Up and Step-Down

Transformer Equations and Principles

Power Transmission and Transformers

Efficiency and Challenges in Power Transmission

Conclusion: The Future of Transformers in Power Systems

Introduction to Transformers

Step 1: Understanding the Purpose of Transformers

Step 2: AC Voltage and Transformers

Step 3: Components of a Transformer

Step 4: Working Principle of Transformers

Step 5: Reducing Power Loss in Transmission Lines

FAQs

What is the transmission of power?

What is a transformer and how does it work?

What are the 3 types of transformers?

How do transformers work in the power grid?

How do transformers reduce transmission loss?

Prerequisite Topics

Become a member to get more!