Teorema de seno
Examples
Free to Join!
StudyPug is a learning help platform covering math and science from grade 4 all the way to second year university. Our video tutorials, unlimited practice problems, and step-by-step explanations provide you or your child with all the help you need to master concepts. On top of that, it's fun — with achievements, customizable avatars, and awards to keep you motivated.
Easily See Your Progress
We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.Make Use of Our Learning Aids
Earn Achievements as You Learn
Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.Create and Customize Your Avatar
Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Topic Notes
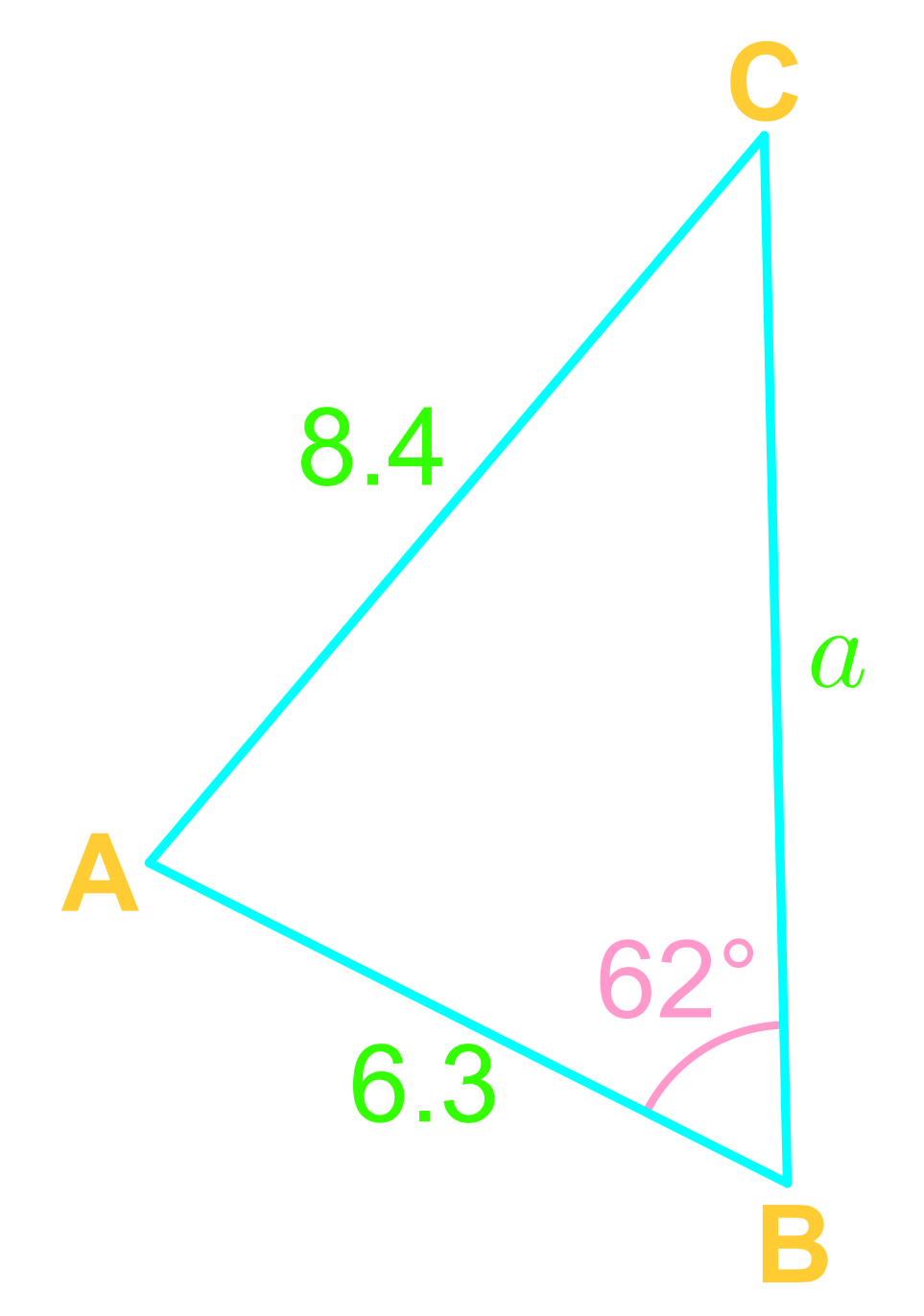
Para cualquier △ ABC,
Sen(A)a =Sen(B)b =sin(C)c
Y
aSen(A) =bSen(B) =cSen(C)
¡Así se puede usar uno de estos pares con el Teorema de Seno para resolver problemas!
Caso ambiguo:
El caso ambiguo del Teorema de Seno proviene del caso LLA (lado-lado-ángulo).
Sen(A)a =Sen(B)b =sin(C)c
Y
aSen(A) =bSen(B) =cSen(C)
¡Así se puede usar uno de estos pares con el Teorema de Seno para resolver problemas!
Caso ambiguo:
El caso ambiguo del Teorema de Seno proviene del caso LLA (lado-lado-ángulo).
- Paso 1: Utiliza el ángulo en cuestión para encontrar la altura del triángulo: h=bsin(A)
- Paso 2: Revisa si,
Lado a < h, entonces no triángulos
Lado a = h, entonces 1 triángulo
Lado a > h, entonces 1 triángulo
h < Lado a < Lado b, entonces 2 triángulos - Paso 3: ¡Resuelve los triángulos!
2
videos
remaining today
remaining today
5
practice questions
remaining today
remaining today