Derivative of ln x
So far in Calc, we have learned how to find trig derivatives such as the derivative of , and the derivative of . We have also done harder trig derivatives like the derivative of . Next, we went one step farther and looked at derivatives of inverse trig functions like and . Not only that, but we've also looked at differentiating exponential functions like the derivative of and even more difficult ones like the derivative of . Give yourself a pat in the back because there is only one last derivative to look at now. This will be the derivative of the logarithmic function .
How to Find Derivative of lnx
To find the derivative of , let us start from the very beginning. Consider the equation
-

Equation 1: Derivative of lnx pt.1 Note that ln is log with base e, so this can be rewritten as
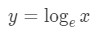
Equation 2: Derivative of lnx pt.2 We need to convert this into exponential form. Doing so will give us the following equation:
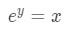
Equation 3: Derivative of lnx pt.3 Let's assume that this is differentiable. Now what we want to do here is something called implicit differentiation. In other words, we want to take the derivative of both sides of the equation. Doing so will give us,
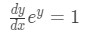
Equation 4: Derivative of lnx pt.4 Notice that dy/dx shows up in the equation because of the chain rule. If you don't understand implicit differentiation or the derivative of exponential functions, we prefer you click those hyperlinks!
Here is the interesting part. Recall that . We can actually substitute y with this in our equation. Doing this will give us:
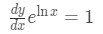
Equation 5: Derivative of lnx pt.5 Now you may think to yourself that we just made the equation even more complicating, but do not worry! The in our equation can be simplified to . If you have trouble understanding that, we recommend you to look at the section "Ln as inverse function of exponential function" of this link
http://www.rapidtables.com/math/algebra/Ln.htmSince , then we can simply our equation to
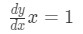
Equation 6: Derivative of lnx pt.6 Lastly we divide both sides of the equation by x, thus giving us the final result:
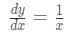
Equation 7: Derivative of lnx pt.7 We're done!
How to Find Second Derivative of lnx
First of all, recall that the first derivative of is:
-
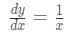
Equation 7: Derivative of lnx pt.7 Taking the second derivative is not that challenging because we only need to use the quotient rule. Recall that the formula of the quotient rule is:
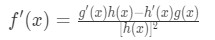
Formula 1: Quotient Rule The second derivative will be:
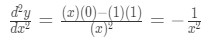
Equation 8: Second Derivative of lnx pt.1 Hence we can conclude that:
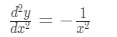
Equation 9: Second Derivative of lnx pt.2
Proof of the Derivative of lnx
You may think that finding the derivative of is the same as proving it, but it is actually a little bit different. This is because we cannot assume that is differentiable. Hence we cannot use implicit differentiation like we did earlier. This means that we are going to use a different approach to proving the derivative of .
First of all, consider the following equation:
-
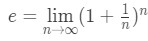
Equation 10: Proof of Derivative of lnx pt.1 We are going to change some variables here and say that and so . Notice that for the equation as →, we see that →. Hence we can rewrite our formula to be the following:
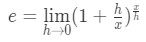
Equation 11: Proof of Derivative of lnx pt.2 Then we can take both sides of the equation to the power of , thus giving us
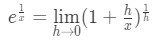
Equation 12: Proof of Derivative of lnx pt.3 We will use this formula later in the proof and do a substitution.
Now to take the derivative of , we need to go back to the very beginning and use the definition of derivative. Recall that the definition of derivative is
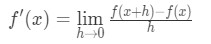
Formula 2: Definition of Derivative If , then we will have that
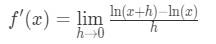
Equation 13: Proof of Derivative of lnx pt.4 Using the logarithm quotient rule, we can change our equation to be
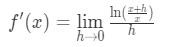
Equation 14: Proof of Derivative of lnx pt.5 Simplifying this equation will give us
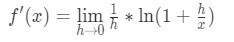
Equation 15: Proof of Derivative of lnx pt.6 We can then use the Logarithm power rule so that
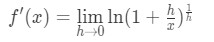
Equation 16: Proof of Derivative of lnx pt.7 Again, if you are not very familiar with logarithm rules, then we suggest you look at the section "Natural logarithm rules and properties" of this link.
Now using the property of limits, we can transport the limit so that it is inside the brackets of ln. This will give us
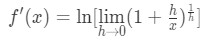
Equation 17: Proof of Derivative of lnx pt.8 Now you might be asking yourself, why does the inside brackets of ln look so familiar? Well that's because earlier we showed that
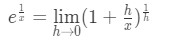
Equation 18: Proof of Derivative of lnx pt.9 So we could actually do a substitution here! Doing so will give us
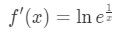
Equation 19: Proof of Derivative of lnx pt.10 Using the Logarithm power rule, we can bring the as a coefficient. This gives us
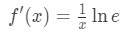
Equation 20: Proof of Derivative of lnx pt.11 Finally, we know that , so finally we can say that
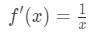
Equation 21: Proof of Derivative of lnx pt.12






