Rolle's Theorem
What is Rolle's Theorem?
Rolle's Theorem is one of the most important Calculus theorems which say the following:
Let f(x) satisfy the following conditions:
- The function f is continuous on the closed interval [a,b]
- The function f is differentiable on the open interval (a,b)
- f(a) = f(b)
Then there must be a number c such that a < c < b and f'(c) = 0. Now this theorem is only true if the three conditions are fulfilled. Though the third condition is easy to verify, a lot of people struggle to see if the and conditions are fulfilled. Let's take a look at some methods we can use to see if a function is continuous and differentiable.
How to Tell if a Function is Continuous
The best way to see if a function is continuous is by finding values of that makes the function undefined. Then we see if the value is within the closed interval [a,b]. If it is, then the function is discontinuous. If it is not, then it is continuous. Let's look at an example.
Question 1: Show that function f is continuous on the closed interval [1,7].
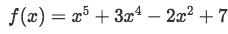
If you look at the function carefully, you will notice that this is a polynomial. Generally, the domain of a polynomial is:
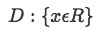
This means that there is no values in which the function is undefined. In other words, the function is continuous from to . If that's the case, then it must also be continuous on [1,7]. Okay so that was really easy. Let's look at a harder question.
Question 2: Is the function f continuous on the closed interval [0,2]?
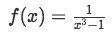
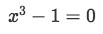
Equation 2: Discontinuous Rational Question pt.2 Adding both sides by 1 gives:
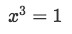
Equation 2: Discontinuous Rational Question pt.3 -
Cube rooting both sides lead us to have
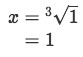
Equation 2: Discontinuous Rational Question pt.4 So the function is undefined at x = 1. Now is this within the interval [0,2]? We see that it is! So the function is not continuous at [0,2]. Now that we are familiar with continuity, let's take a look at what it means to be differentiable.
This is fraction, and a fraction already has a problem if the denominator is equal to 0. This is because we cannot divide by 0. So there is bound to be a value in each the function is undefined. Let's set the denominator equal to 0 to see what that is:
What Does it Mean to be Differentiable?
A function is differentiable at (a, b) if its derivative exists within that interval. So all we really have to do here is take the derivative, and check the domain. If an value within the interval (a, b) makes the derivative undefined, then we say that the function f(x) is not differentiable. Let's look at an example.
Question 3: Is the function differentiable on the open interval (-1,1)?
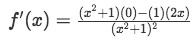
Equation 3: Differentiable Rational pt.1 Simplifying gives us:
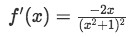
Equation 3: Differentiable Rational pt.2 -
Now we are going to look at when the derivative is undefined. Again, the best way to do this is by setting the denominator equal to 0. Doing so gives us:
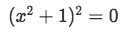
Equation 3: Differentiable Rational pt.3 -
Square rooting both sides of the equation leads to
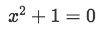
Equation 3: Differentiable Rational pt.4 -
Subtracting both sides by 1 gives:
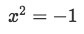
Equation 3: Differentiable Rational pt.5 -
Square rooting both sides again leads to an x value:
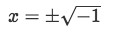
Equation 3: Differentiable Rational pt.6 Notice that this is an imaginary number, so this isn't a valid x value. That means there are no x values which makes the derivative undefined. So we can conclude that the function is differentiable at (-1,1). Let's do a different example.
First, we need to take the derivative. Doing so gives us:
Question 4: Is the function differentiable on the open interval (-4, 4)?
-
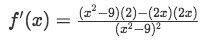
Equation 4: Non-Differentiable Rational pt.1 -
Simplifying the numerator gives:
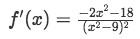
Equation 4: Non-Differentiable Rational pt.2 -
Again, let's set the denominator equal to 0 to see when the function is undefined.
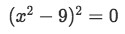
Equation 4: Non-Differentiable Rational pt.3 -
Square rooting both sides gives:
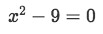
Equation 4: Non-Differentiable Rational pt.4 -
Adding 9 to both sides leads to
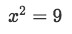
Equation 4: Non-Differentiable Rational pt.5 -
Square rooting both sides again leads to 2 x-values:
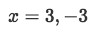
Equation 4: Non-Differentiable Rational pt.6 So these values make the derivative undefined. Also notice that these x values are within the open interval (-4, 4). Hence, we conclude that that the function f(x) is not differentiable at (-4, 4).
Again, taking the derivative gives us:
Rolle's Theorem Proof
Now that were familiar with the conditions of Rolles Theorem, let's actually prove the theorem itself. Rolle's Theorem says that if a function f(x) satisfies all 3 conditions, then there must be a number c such at a < c < b and f'(c) = 0. We can show that this is always true if we prove that it is true for each of these cases:
- A function with only a constant at [a,b]
- A function with a maximum at [a,b]
- A function with a minimum at [a,b]
Let's look at each case separately.
A Function with Only a Constant
Consider the function f (x) = k within the interval [a,b], where k is a constant. Notice that differentiating the function gives:
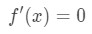
This is interesting because the derivative is always 0. Since f'(x) = 0 for all x, then we can pick any number within the interval [a,b] as c. Thus, we found a number c such that a < c < b and f'(c) = 0. That was very easy! Let's look at the second case.
A Function with a Maximum
Consider some number k in the interval [a,b] such that f (k) > f(a) = f(b). If f(x) is continuous, then that means there exists a maximum at point c. Since we know f(x) is differentiable (from the 2nd condition), then we know that f'(x) exists. Since f'(x) exists and there is a maximum within the interval [a,b], then we know that f'(c) = 0 within [a,b]. In other words, there exists a number c such that a< c < b and f'(c) = 0. Lastly, let's look at the third case.
A Function with a Minimum
Consider some number k in the interval [a,b] such that f (k) < f(a) = f(b). If f(x) is continuous, then that means there exists a minimum at point c. Since we know f(x) is differentiable (from the 2nd condition, then we know that f'(x) exists. Since f'(x) exists and there is a minimum within the interval [a,b], then we know that f'(c) = 0 within [a,b]. In other words, there exists a number c such that a < c < b and f'(c) = 0.
Since all cases are true, then Rolle's Theorem is proved. Now let's actually do some examples of Rolle's Theorem.
Rolle's Theorem Examples
Question 5: Show that the equation has exactly one real root.
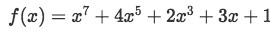
-
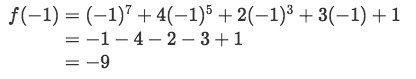
Equation 6: One Real root Polynomial pt.2 -
In addition, we see that
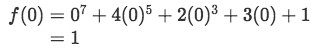
Equation 6: One Real root Polynomial pt.3 -
Since -9 < 0 < 1 and f(x) is a polynomial that is continuous everywhere, then the Intermediate Value Theorem states that there must be a number c such that -1 < c < 0, and f(c) = 0. In other words, the function must have at least one real root. However, we need to show that f(x) has exactly one real root, not at least one. So what do we do?
-
We do something called a contradictory proof. Let's assume that f(x) has at least two real roots. That means we can find at least two x values (call it a, b) where f(a) = 0 and f(b) = 0. Now here is the interesting part. If you didn't notice, we actually satisfied all the condition for Rolle's Theorem. First, f(x) is a polynomial. So it is continuous and differentiable anywhere. This satisfies the 1s and 2nd condition. Lastly, we know that f(a) = f(b) because they both equal 0. So this satisfies the 3rd condition. So what does Rolle's Theorem say about this? Well, it says that much be a number c such that a < c < b and f'(c) = 0.
-
But look at this! If we take the derivative of f(x), we get:
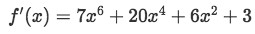
Equation 6: One Real root Polynomial pt.4 -
If you look closely, the derivative can never equal to 0! This is because the exponents are even, and that always leads to positive terms. You also have to add 3 to it, so it becomes even more positive. So therefore, we are certain that it is always > 0.
-
Since f'(x) > 0 for all x, then it is impossible get to f'(x) = 0. So there is no number c such that f'(c)=0. Rolle's Theorem actually ends up failing here (which is impossible), so we have a contradiction.
-
Contradictions happen because we made a wrong assumption in the first place. Recall we assumed that "there is at least 2 real roots". So since the assumption leads us to something mathematically illogical, then we say that it is impossible to have at least 2 real roots. This means there can only be exactly one real root. Thus, we answered the question.
Now from this function, you should realize that this is a degree polynomial. Therefore it has a total of 7 roots. Since the question states that it has only one real root, then the six other roots must be complex. How do we show this? Well, notice that
Let's look at another Rolle's Theorem example.
Question 6: Let . Does Rolle's Theorem guarantee the existence of c from the interval [-5,1]?
- First, let's see if f(x) satisfies the three conditions.
- Is the function continuous from [-5,1]? Well the function is a polynomial, so it is continuous everywhere. So it must be continuous from the closed interval [-5,1].
- Is the function differentiable from (-5,1)? Well again, the function is a polynomial. So it must be differentiable everywhere. Hence, it must be differentiable from the open interval (-5,1).
- Does f(-5)=f(1). Well we see that:
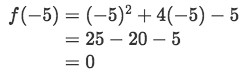
Equation 6: Rolle's Theorem example pt.1 -
And
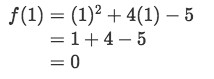
Equation 6: Rolle's Theorem example pt.1 -
Hence we can conclude that f(-5)=f(1). Since all 3 conditions are fulfilled, then Rolle's Theorem guarantees the existence of c. To find c, we solve for f'(x)=0 and check if -5 < x < 1. Notice that
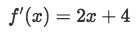
Equation 6: Rolle's Theorem example pt.3 -
Setting it equal to 0 gives
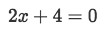
Equation 6: Rolle's Theorem example pt.4 -
Isolating for x gives:
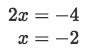
Equation 6: Rolle's Theorem example pt.5 -
Notice that -5 < x = -2 < 1, so we can say that the number c = -2. If you want to do more practice questions, we suggest you click this link below.
http://tutorial.math.lamar.edu/Problems/CalcI/MeanValueTheorem.aspx
There are a lot of practice questions involving Rolle's Theorem, and there are also step by step solutions!
Now that we are familiar with Rolle's Theorem, let's take a look at the Mean Value Theorem.
Mean Value Theorem
What is the Mean Value Theorem? In differentiable calculus, the Mean Value Theorem (MVT) is the expansion of Rolle's Theorem. The Mean Value Theorem states that there exists a number c such that a < c < b, and
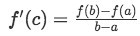
Even though the word "mean" is in this theorem, you can see that it has nothing to do with "average". The equation doesn't even relate to the formula for mean. Anyways, let's look at how we can prove this theorem.
Mean Value Theorem Proof
In order to prove the Mean Value Theorem (MVT), we need to again make these assumptions:
Let f(x) satisfy the following conditions:
- f(x) is continuous on the interval [a,b]
- f(x) is differentiable on the interval (a,b)
Now, consider two points (a, f(a)) and (b, f(b)) in the function. We are going to draw a line between those points and call that the secant equation.

We see that this is a straight line. So from this graph, one can conclude that we need to write an equation for this straight line. Recall that the equation of a line in point slope form is:
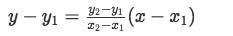
So our equation of the secant line is:
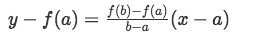
Moving f(a) to the other side of the equation gives:
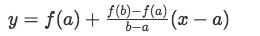
Now we are going to create another function called g(x). This function is the subtraction between the function f(x) and the secant line y. In other words,
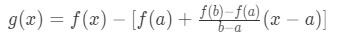
Notice that g(x) is also continuous and differentiable because a subtraction of two continuous and differentiable function is still continuous and differentiable. Hence, we can take the derivative. Taking the derivative will give us:
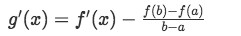
Now we have to somehow relate this to Rolle's Theorem. How? Well, Rolle's Theorem holds for three conditions, but we only have two. In other words, we know that g(x) is continuous and differentiable, but we are missing the condition where g(a) = g(b). Let's see if this is true.
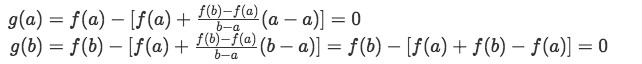
Notice that g(b) =g(a), and therefore g(x) satisfies the three conditions of Rolle's Theorem. Thus, we know that there exists a number c such that g'(c) = 0 within the interval [a,b]. We can also use this fact for g'(x). Recall g'(x) was
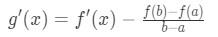
So if g'(c) = 0, then
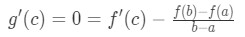
This would imply that
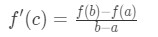
So we just proved the Mean Value Theorem! If you are interested in the Mean Value Theorem for derivatives and Mean Value Theorem for integrals, then we suggest you to look at the Mean Value Theorem section.
Let satisfy the three following conditions:
1) is continuous on the interval
2) is differentiable on the interval
3)
If the conditions are fulfilled, then Rolle's Theorem states that there must be a number (call it ) such that and .






