Areas Between Curves: Essential Calculus Concepts Unlock the power of calculus by mastering areas between curves. Learn integration techniques, handle intersecting functions, and apply your skills to real-world problems in physics and engineering.
Free to Join!
Easily See Your Progress
 We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.
We track the progress you've made on a topic so you know what you've done. From the course view you can easily see what topics have what and the progress you've made on them. Fill the rings to completely master that section or mouse over the icon to see more details.Make Use of Our Learning Aids
Earn Achievements as You Learn
 Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.
Make the most of your time as you use StudyPug to help you achieve your goals. Earn fun little badges the more you watch, practice, and use our service.Create and Customize Your Avatar
 Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.
Play with our fun little avatar builder to create and customize your own avatar on StudyPug. Choose your face, eye colour, hair colour and style, and background. Unlock more options the more you use StudyPug.

Introduction to Area Between Two Curves
Finding the area between two curves is a fundamental concept in calculus that builds upon the idea of calculating the area under a single curve. This topic is crucial for students advancing their understanding of integral calculus. Our introduction video serves as an essential starting point, providing a clear and visual explanation of this concept. It demonstrates how to identify the region between two functions and set up the integral to compute its area. This method extends the techniques learned for finding the area under one curve, now applied to the space enclosed by two functions. By mastering this concept, students gain valuable skills for solving more complex problems in physics, engineering, and economics. The video emphasizes key strategies and common pitfalls, ensuring a solid foundation for further study in calculus and its applications.
Understanding the region between two functions is essential for students who wish to excel in calculus. The space enclosed by two functions can be tricky to visualize at first, but with practice, it becomes more intuitive. Additionally, the skills learned here are not just limited to mathematics; they are also applicable in various fields such as physics and engineering. For instance, solving complex problems in physics often requires a solid understanding of these concepts.
Find the area of the region enclosed by the parabolas y=(x−3)2 and y=−x2+8x−15
Step 1: Understand the Problem
We need to find the area of the region enclosed by two parabolas. The given parabolas are y=(x−3)2 and y=−x2+8x−15. To find the area between these curves, we need to determine the points of intersection and then integrate the difference of the functions over the interval defined by these points.
Step 2: Find the Points of Intersection
To find the points of intersection, we set the equations equal to each other and solve for x: (x−3)2=−x2+8x−15 Expanding and simplifying, we get: x2−6x+9=−x2+8x−15 Combining like terms, we obtain: 2x2−14x+24=0 Factoring out the 2, we get: x2−7x+12=0 Factoring the quadratic equation, we find: (x−3)(x−4)=0 Therefore, the points of intersection are x=3 and x=4.
Step 3: Determine the Bounds and Curves
We now have our bounds for integration: x=3 and x=4. The top curve is y=−x2+8x−15 and the bottom curve is y=(x−3)2.
Step 4: Set Up the Integral
To find the area between the curves, we integrate the difference of the top curve and the bottom curve from x=3 to x=4: ∫34((−x2+8x−15)−(x2−6x+9))dx Simplifying the integrand, we get: ∫34(−2x2+14x−24)dx
Step 5: Evaluate the Integral
We now evaluate the integral: ∫34(−2x2+14x−24)dx The antiderivative of −2x2 is −32x3, the antiderivative of 14x is 7x2, and the antiderivative of −24 is −24x. Therefore, we have: (−32x3+7x2−24x)34 Plugging in the bounds, we get: (−32(4)3+7(4)2−24(4))−(−32(3)3+7(3)2−24(3)) Simplifying each term, we find: (−3128+112−96)−(−354+63−72) Further simplification gives: (−3128+16)−(−18+63−72) (−3128+16)−(−27) (−3128+16+27) Converting 16 and 27 to fractions with a common denominator, we get: (−3128+348+381) (3−128+48+81) (31) Therefore, the area of the region enclosed by the parabolas is 31 square units.
Here are some frequently asked questions about areas between curves:
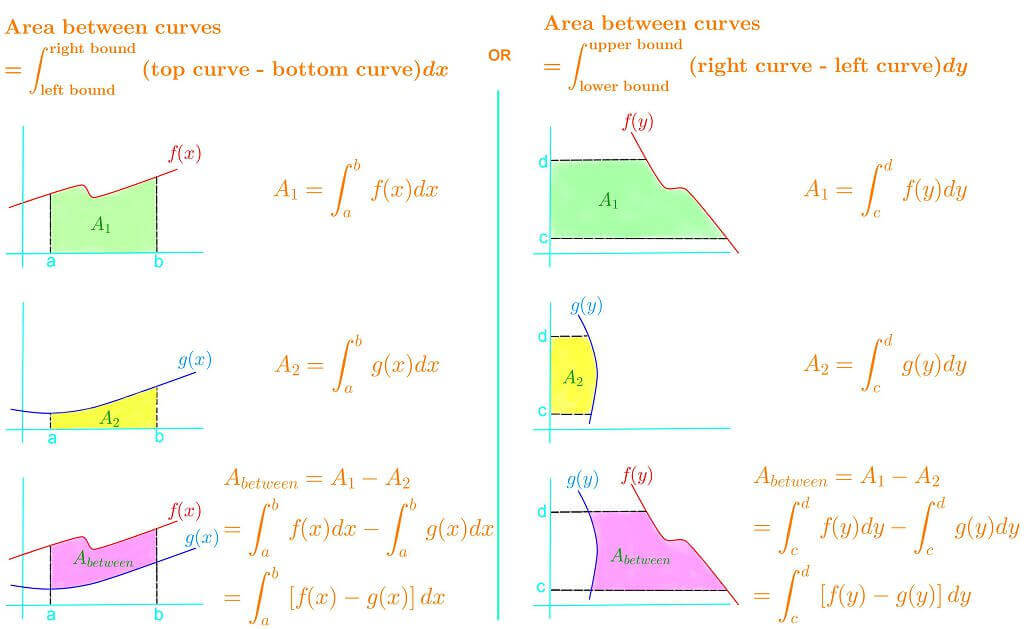
1. What is the basic formula for finding the area between two curves?
The basic formula for finding the area between two curves f(x) and g(x) from a to b is:
Area = (a to b) (f(x) - g(x)) dx
Where f(x) is the upper curve and g(x) is the lower curve. It's crucial to correctly identify which function is the upper and which is the lower within the given interval.
2. How do you handle intersecting curves when calculating the area?
When curves intersect, you need to split the integral at the intersection points. First, find the intersection points by setting the functions equal to each other. Then, set up separate integrals for each subinterval, making sure to correctly identify the upper and lower functions in each subinterval. Finally, sum the results of all integrals to get the total area.
3. When should you consider integrating with respect to y instead of x?
Integrating with respect to y (vertical integration) is useful when dealing with curves that are more easily expressed as functions of y, or when curves are vertical or nearly vertical in the xy-plane. This approach can simplify problems where expressing curves as functions of x would be complex or impossible.
4. How can you check if your area calculation is correct?
To verify your area calculation, you can: 1. Use technology like graphing calculators to visualize and compute the area. 2. Estimate the area visually by comparing it to simple geometric shapes. 3. Solve the problem using a different method (e.g., horizontal vs. vertical integration) and compare results. 4. Check your work for common errors like incorrect limits of integration or misidentified upper/lower functions.
5. What are some real-world applications of calculating areas between curves?
Calculating areas between curves has numerous practical applications, including: 1. In physics, to compute work done by a variable force. 2. In economics, to calculate consumer and producer surplus. 3. In engineering, to determine fluid flow rates or pressure distributions. 4. In statistics, to find probabilities in continuous probability distributions. 5. In biology, to model population growth or drug concentration over time.
Understanding the areas between curves is a crucial concept in calculus and advanced mathematics. However, to fully grasp this topic, it's essential to have a solid foundation in certain prerequisite subjects. Two key areas that play a significant role in comprehending areas between curves are graphing reciprocals of quadratic functions and upper and lower bounds.
Let's start by exploring the importance of graphing reciprocals of quadratic functions. This skill is crucial for visualizing and understanding the shapes of various curves that might be involved in area calculations. When dealing with areas between curves, you'll often encounter different types of functions, including reciprocals of quadratic functions. Being able to graph these functions accurately allows you to visualize the region between two curves more effectively, which is the first step in calculating the area.
Moreover, proficiency in graphing functions for area calculation helps you identify key points of intersection between curves, which are essential for setting up the integral to compute the area. By mastering this prerequisite, you'll be better equipped to tackle more complex problems involving areas between curves, as you'll have a strong visual understanding of the functions' behavior.
Equally important is the concept of upper and lower bounds. In the context of areas between curves, understanding upper and lower functions is crucial. When calculating the area between two curves, you need to determine which function serves as the upper bound and which serves as the lower bound within the given interval. This knowledge is fundamental for correctly setting up the integral and ensuring accurate results.
The ability to identify and work with upper and lower bounds also helps in more advanced applications, such as optimization problems or analyzing the behavior of functions within specific regions. By having a solid grasp of this concept, you'll be better prepared to handle various scenarios involving areas between curves, including cases where the upper and lower functions may switch roles at certain points.
In conclusion, mastering these prerequisite topics - graphing reciprocals of quadratic functions and understanding upper and lower bounds - is essential for success in working with areas between curves. These foundational skills provide the necessary tools for visualizing, setting up, and solving problems related to this important mathematical concept. By investing time in strengthening your knowledge in these areas, you'll build a robust framework for tackling more complex calculus problems and applications involving areas between curves.



