Applications of Inequalities: From Theory to Practice Discover how inequalities shape real-world decision-making. Master techniques for solving complex problems, from budgeting to production planning, and enhance your analytical skills.
Get the most by viewing this topic in your current grade. Pick your course now.
Intros
Examples
- Solving two-variable linear inequalities graphically
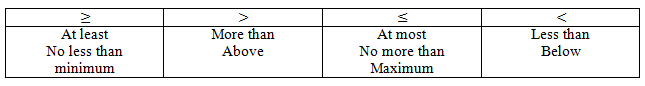
A restaurant charges adults $30 and children $20 for a set dinner. How many adults and children do they have to serve such that they will have a revenue of more than $2700? - Thomas participates in a professional gaming tournament. He scores 1 point for every game he wins and scores 0.5 points for every game that ends in a draw. How many wins (W) and draws (D) should there be such that he will score at least 8 points in total?
- Solving systems of two-variable linear inequalities graphically
A company needs to restock the stationary at the office. A pen (P) costs $2 and a marker (M) costs $5. Since they are on a tight budget, they can spend no more than $100 in restocking. However, they want to purchase at least 40 items in total so that the stationary store will provide free delivery. How many pens and markers is the company able to purchase?
Introduction to Applications of Inequalities
Welcome to our exploration of the Applications of Inequalities, a fundamental concept in mathematics with wide-ranging real-world implications. This section begins with an introductory video that underscores the significance of inequalities in mathematical studies and everyday problem-solving. As we delve deeper, we'll uncover how inequalities are not just abstract concepts but powerful tools used in various real-world situations and word problems. From optimizing business decisions to solving scientific challenges with inequalities, inequalities play a crucial role. We'll examine practical examples that demonstrate how these mathematical principles are applied in fields such as economics, engineering, and social sciences. By understanding the applications of inequalities, you'll gain valuable insights into how mathematics shapes our world and helps us make informed decisions. Join us as we bridge the gap between theoretical knowledge and practical application in this fascinating area of mathematics.
-
What are the most common inequality symbols used in mathematics?
The most common inequality symbols are:
- (greater than or equal to)
- > (greater than)
- (less than or equal to)
- < (less than)
These symbols are used to express relationships between quantities in mathematical expressions and real-world scenarios.
-
How do you translate "at least" into a mathematical inequality?
"At least" is typically translated to the inequality symbol "" (greater than or equal to). For example, if a problem states "x is at least 5," it would be written mathematically as "x 5". This means that x can be 5 or any number greater than 5.
-
What is linear programming and how does it use inequalities?
Linear programming is an optimization technique used to find the best outcome in a mathematical model whose requirements are represented by linear relationships. It uses inequalities to represent constraints on resources or conditions. For example, in a production problem, inequalities might represent limitations on raw materials or labor hours. The goal is to maximize or minimize an objective function (like profit or cost) while satisfying these inequality constraints.
-
How are inequalities used in everyday life?
Inequalities are used in many everyday situations, such as:
- Budgeting: Ensuring expenses don't exceed income
- Time management: Allocating sufficient time for tasks
- Speed limits: Staying below the maximum allowed speed
- Cooking: Using the right amount of ingredients
- Shopping: Finding items within a price range
In these cases, inequalities help in making decisions and setting boundaries.
-
What is the difference between strict and non-strict inequalities?
Strict inequalities use the symbols ">" (greater than) or "<" (less than) and do not include the equality case. For example, x > 5 means x must be strictly greater than 5, not including 5 itself. Non-strict inequalities use "" (greater than or equal to) or "" (less than or equal to) and include the equality case. For instance, x 5 means x can be 5 or any number greater than 5. Understanding this difference is crucial in correctly interpreting and solving inequality problems.
Understanding the applications of inequalities is a crucial skill in mathematics, but to truly grasp this concept, it's essential to have a solid foundation in several prerequisite topics. One of the fundamental skills needed is the ability to perform application of integer operations. This skill is vital when translating word problems into inequalities, as it allows you to manipulate numerical values accurately within the context of real-world scenarios.
Building upon this foundation, solving multi-step linear inequalities becomes a critical skill. This process involves understanding how to manipulate equations with inequality symbols, which is directly applicable to many real-world situations where you need to find a range of possible solutions rather than a single answer.
As you progress, solving quadratic inequalities becomes another important skill. This topic extends your understanding to more complex scenarios, allowing you to analyze situations that involve parabolic relationships and find solutions that satisfy these more advanced inequalities.
Visualization is also a key aspect of working with inequalities. Graphing quadratic inequalities in two variables helps you represent these mathematical concepts visually, making it easier to interpret and solve complex problems. This skill is particularly useful in fields like economics, engineering, and data science.
When it comes to practical applications, understanding linear programming word problems is essential. This topic combines inequalities with optimization techniques, allowing you to solve real-world problems involving resource allocation, profit maximization, and cost minimization.
Lastly, a strong grasp of the distributive property in algebra is crucial. This property is often used when solving complex inequalities, especially those involving parentheses or multiple variables. It allows you to simplify expressions and solve equations more efficiently, which is invaluable when dealing with applications of inequalities in various fields.
By mastering these prerequisite topics, you'll be well-equipped to tackle the applications of inequalities with confidence. Each of these skills builds upon the others, creating a comprehensive understanding that will serve you well in both academic and real-world contexts. Remember, mathematics is a cumulative subject, and a strong foundation in these prerequisites will make your journey through more advanced topics smoother and more rewarding.